It’s an unpresupposing little letter, $x$. In fact, that’s the reason we use it to represent something we don’t know. But how do you write it down? When Vijay Krishnan tweeted a link to an American college professor’s page on mathematical handwriting, I was shocked to learn that he thought adding a hook to a simple cross was sufficient to differentiate letter-$x$ from times-$\times$.
So I asked our Twitter followers how they write $x$. The Cambrian explosion of diversity in answers I received was eye-opening – I’m glad I asked!
I’ve collected the tweets together in a story on Storify.
In summary, it seems that no. 3 is prevalent only in the British Commonwealth and Russia, while no. 2 only appears in the US. Continental Europeans seem to use no. 1, though the calligraphic capital $\mathcal{X}$ caused some confusion. Commentators on either side of the Atlantic were aghast at each other’s orthography, and thought the reasons for their own methods were self-evident. I think we spread a little understanding in the end though.
As a postscript, I have to mention the reference for all things to do with mathematical notation, Cajori’s A History of Mathematical Notations ((Warning: if you start reading Cajori, the Sun might go up and down a few times before you stop reading Cajori.)). In particular, Cajori tells us that it was probably Descartes who introduced the use of $x$ (and $y$ and $z$) to represent unknowns.
Sadly, it doesn’t look like Cajori concerns himself with handwritten maths after the adoption of printing in Europe, so I don’t know where or when the British/Russian $x$ first appeared. Florian Cajori: lightweight scholar!
And that’s my final word on the letter $x$.
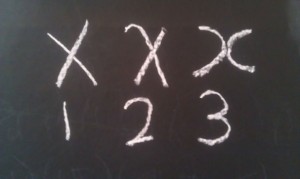
In this link: http://t.co/hVCVPGFZ , you can see how Sir Isaac Newton wrote his xs, back in the days when knighthoods meant something. It looks to me like something like )/( , meaning he wouldn’t have to take his quill off of the paper.