Irregulars | The Aperiodical

You're reading: Irregulars

Continued fractions

By David Benjamin. Posted November 29, 2024

This is a guest post by David Benjamin.

Philip Henslowe’s Card Trick

By Colin Beveridge. Posted September 23, 2024

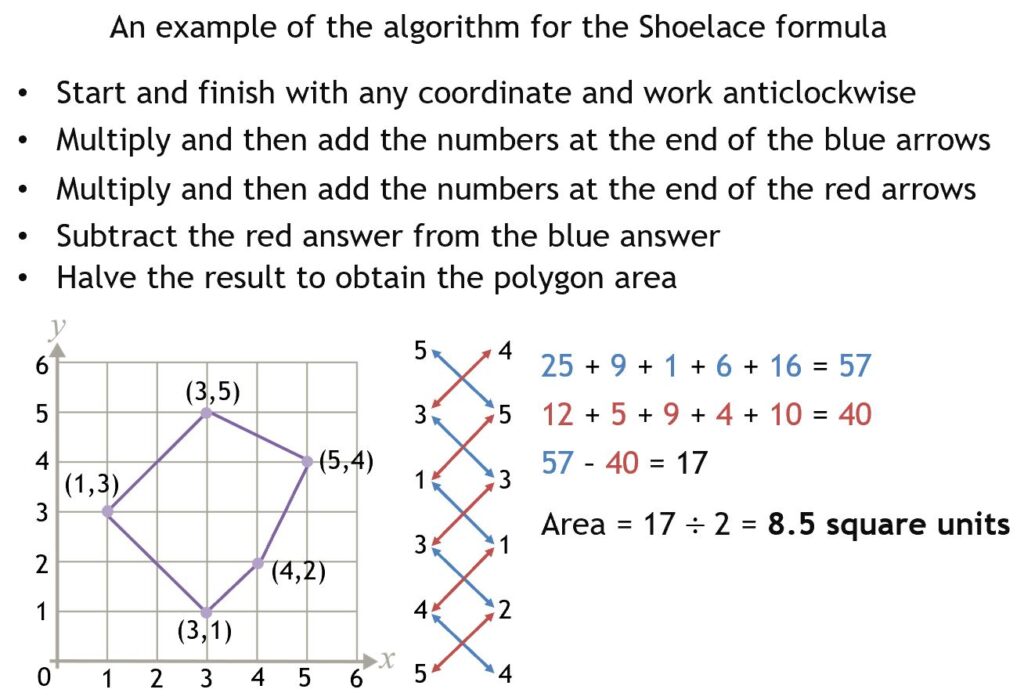
In a dimly-lit tavern on the South Bank of the Thames, Philip Henslowe — builder and owner of the Rose Theatre — is celebrating the success of Shakespeare’s latest blockbuster, Henry VI Part I, among the cutthroats, actors and other lowlife of London. He spreads thirteen playing cards on a table in a circle. “Pick a card,” he grins. “Any card.”

Henslowe, one of Elizabethan theatre’s most important figures, kept a diary. It’s mainly the accounts of the theatre and records of loans, but among the administrivia are some gems — including the following card trick:

A handwritten diary page describing a card trick recorded by Philip Henslowe. — Dulwich College Archive MS VII f18v — with kind permission of the Governors of Dulwich College

My dogged team of researchers is looking into it, but there are very few documented card tricks from this era — and most of them are sleight-of-hand or forces. Tiago says this might be related to something written by Pacioli in 1478, and I understand there are Italian deck-stacking tricks from the first half of the 16th century. While it’s relatively unremarkable now, it seems quite sophisticated for its time.

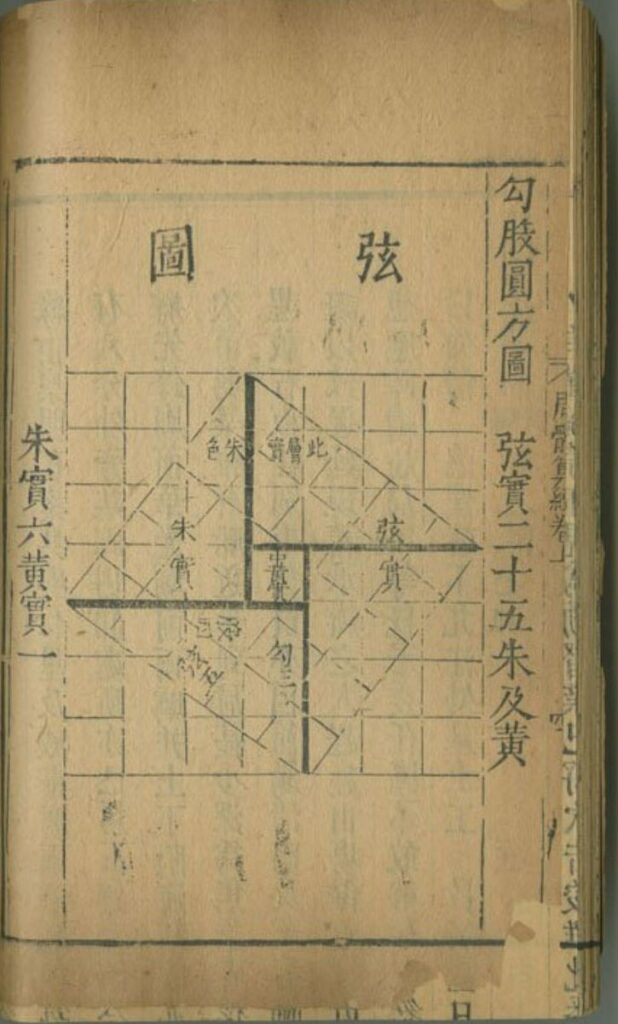
Luckily, the trick was transcribed by W. W. Greg barely 300 years after having been scrawled out by Henslowe:

Screenshot of a PDF of the transcription. Text reads: "A watche at cardes to tell a man at what ower he thincketh to Risse proved trewe"; then a horizontal line; then "tacke xij cardes [w^th (and)] the knaue of clubes & laye them Round licke a clocke turnynge them all ther faces downward but the knave of clubes & laye hime vnder neth licke you^r watche & laye them this- then aske the ptie at what ower he will Risse & leat him kepe yt to hime seallfe & yf he thincke vij then poynte hime a a card to teall frome & bead hime yf he thincke vij to teall the card viij observinge this Rewle frome your leafte hand a cownt; that j for xv always & when you will have the ptie ^tell a poynt hime to tell toward you^r Right hand to what card you will a poynte hime you muste tell to your sealfe as before toward you leafte hand be ginynge at the j wch is xv & so tell them vpward as thus 15 16 17 18 19 20 21 22 23 24 & w^ch of al thes nvmbers that you poynte vpon bead him tell frome that w^ch he thinketh in his mind tel he haue towld to so maney toward his Right hand & then leat hime turn yt vp & yt shalbe that w^ch he thincketh a proved." To the right is a diagram of the numbers 1-12 arranged in a circle, with an X in place of 10.

Now, I don’t know about you, but I’m not fluent in shorthand-infused streams-of-consciousness written in Early Modern English. Maths communication has evidently come a long way in the last 400 years. Here’s the best I can do as a more-or-less faithful translation:

Take 12 cards and the jack of clubs and lay them in a circle like a clock, all face down except for the jack. Put the jack at the bottom like on your watch [ed: I have never owned a watch with the jack of clubs at the bottom of it, but let’s roll with it], laid out like [the picture]. Then ask the volunteer what time they will get up and to keep it to themself.

Tell them to pick a card to count from [ed: It’s unclear to me whether the trickster or the volunteer picks the card — it doesn’t make a difference, so I’d let the volunteer do it]. Starting from this card and moving clockwise, they should count from their card up to 15 — so if they picked 7, they should count on eight cards.

Going around the circle, you count aloud clockwise while pointing at the cards, saying “15” on the first card clockwise from the jack, “16” on the second and so on up to 26. Tell the volunteer that whichever number you said when you pointed at their current card, they should count anticlockwise from their secret number up to that number.

When they flip the card they land on, it will be the number they first thought of.

I presume “a p^roved” is Early Middle English for “and everyone said WOW! That’s amazing.”

But it doesn’t work.

If you follow the instructions — which, like a game of Telephone that started centuries before the telephone was invented, have been written down from Henslowe’s memory, transcribed by an expert from unclear manuscript, and then translated into modern-day English by someone unqualified to do so. Hi! — you’ll find your “tada!” falls flat, because it’s not their secret number.

Let’s try it: I get up when I want, except on Wednesdays when I’m rudely awakened by the dustmen at 6am. And, rolling a 13-sided die¹ to decide where to start, I get card #3. I need to count on 9 clockwise from there (to make it up to 15), so I end up on card #12. That’s been given the number 26, so I need to count counterclockwise from my number (6) up to 26 — that is, 20 cards backwards. That takes me to 5.

¹ Yes, I do own a 13-sided die. Why do you ask?

Close, but no not-yet-introduced-to-England cigar.

It turns out that, whatever card you start from, and whichever number you pick, you’ll end up on the card immediately before your secret number. This suggests an easy fix: start your counting-aloud from 14 at card #1.

In case you want to do the trick yourself correctly, here are instructions for my version:

Tell your volunteer to think of a secret number from 1 to 12 (don’t let me control your mind!).
Tell them to pick a card (any card! don’t tell me what it is) and count clockwise from their chosen card, starting from their secret number and ending at 15. Have them tell you the card they land on.
Now tell them the numbers attached to the card: whatever “time” their card is on the clock face, give them a number 13 higher.
They must now move anticlockwise from their chosen card, starting from their secret number, ending on the number attached to the card.
Turn over the card they ended up on and say “abracadabra!”.

My glamorous assistant Bill goes through the trick with me. Better magicians than me — which is pretty much everyone — will have ideas about improving the patter and performance.

NOW it works. But why?

You know what else, apart from the later Shakespeare plays, telephones, and cigars, hadn’t arrived in Elizabethan London? I’ll tell you: modular arithmetic. At least, modular arithmetic as we know it — working with remainders goes back to at least Sun Zi in the third century CE, but Euler and Gauss’s formalisations of it were still 150 years away.

I don’t know what Henslowe’s mathematical background was — he was certainly competent at regular arithmetic — so I don’t know whether he understood why the trick worked, whether he came up with it himself, or anything about the history of it. All the same, I’m certain he wouldn’t have used the modulo function.

(In case you’re one of today’s lucky 10,000: modular arithmetic uses the remainder left over when you divide by a given number, like on a clock: 16:00 is the same as 4pm, and we’d say we were working “modulo 12” or “mod 12”, because we are lazy and modulo is far too long a word. The numbers 4 and 16 have the same remainder when you divide them by 12. In this problem, we’ll be working modulo 13.)

Let’s say you’ve picked secret number $s$ and you decide to start from card #$c$. You’re going to count on $ 15-s $ cards from there, so you end up at card #$ (c + 15 – s ) $. (We can think of card 14 as the same as card 1 and so on.)

The number I assign to it is 13 more than the card number. Modulo 13, that’s just the card number — but doing it this way ensures we don’t have to deal with negative numbers. (Negative numbers had probably reached England by this point, but I don’t imagine they were the kind of thing you’d want to have in a card trick.)

In any case, the volunteer is currently at card #$ (c +15 – s ) $ and has been given the target # $ (c +28 – s )$ to count to, starting at their secret number $ s $. That means they’re going to move $ (c +28 – 2s) $ cards back the way they came, starting at card #$ (c + 15 -s ) $. Moving backwards makes it a subtraction, so we work out $ (c + 15 – s ) – (c +28 – 2s ) $ to see that we end up on card #$ (s – 13)$.

And, because there are 13 cards, that’s the same as card #$s$, which has the volunteer’s secret number written on it.

Boom.

One more twist, though

When I talked to young Bill about it, he asked a tremendous mathematical question: “would it work with a number other than 15?” The kid is ten years old, and already making me mutter “good GRIEF, where did that come from?” about three times a month.

The answer is… you don’t need it to be 15. In fact, the first half of the trick is mathematically irrelevant². You could ask them to spell out their secret number in a language of their choice, you could ask them to add their age to their best Parkrun time in minutes, you could ask them to spin a coin and pick the card it lands closest to. It doesn’t matter in the slightest, as long as they pick a card.

² That doesn’t mean it’s not an important part of the trick! I think it’s helpful to demonstrate how you want the final bit counted, and it misdirects the volunteer/audience into thinking there must be something clever going on.

If that’s card #$ C $, then they subtract $ (C + 13) – s $ from it — which again leaves you on card #$ (s – 13) $, which is card #$ s $.

Even knowing the maths behind it, I think this is still a pretty impressive trick. To someone frequenting a smoky Elizabethan tavern, it must have looked like, well, magic.

Thanks to Rob Eastaway for sending me the trick. His book on the maths of Shakespeare, Much Ado About Numbers, is available wherever good books etc. Thanks also to Paul O’Malley and Tiago Hirth for historical help, and to Calista Lucy and the Governors of Dulwich College for permission to reproduce the manuscript page.

Row $ \sqrt{-1} $ of Pascal’s triangle

By Colin Beveridge. Posted July 31, 2024

Hi! My name is Colin, and I am a PROPER mathematician now. I’ve made a contribution to the Online Encyclopaedia of Integer Sequences.

From Zero to Hero: a Euclidean proof

By Colin Beveridge. Posted June 18, 2024

It used to live, unloved, in the A-level formula book: a mysterious result relating the area of a triangle to its sides. The most interesting thing about it was its name: Heron’s formula. (As far as I can make out, the chap’s name was Hero of Alexandria, and if you do a possessive in Greek it goes into the genitive case, which makes it Heron’s Formula. You might want to debate this; I regretfully decline.)

The Call To Adventure

Love Triangle is available wherever good books etc. are, from June 20th – and signed preorders are available from Maths Gear

So there I was, peacefully proofreading Matt Parker’s forthcoming book Love Triangle when I was shocked by a vicious — and frankly unprovoked — assault on the very idea of Heron’s formula.

Hidden in Plane Sight

By Elliott Baxby. Posted April 18, 2024

This is a guest post by Elliott Baxby, a maths undergraduate student who wants to share an appreciation of geometrical proofs.

I remember the days well when I first learnt about loci and constructions – what a wonderful thing. Granted, I love doing them now; to be able to appreciate how Euclid developed his incredible proofs on geometry.

Pythagoras and his theorem

By David Benjamin. Posted October 14, 2023

In this guest post, David Benjamin shares a cornucopia of concepts and stories relating to Pythagoras and his famous theorem.

I admit to mild irritation when I’m told that Pythagoras’ theorem is $a^2+b^2=c^2$. The theorem is based on area – in particular, that of squares. There are many proofs of the theorem and in this post we present a miscellany of Pythagorean Theorem curiosities, including some of my favourite proofs, the theorem’s links to algebra, geometry and number theory, an assassination of a president of the USA, an alleged murder in Greece, an infinite spiral of surds, Gauss and coordinate geometry – plus another connection between Pascal and Fibonacci.

The theorem

A right-angled triangle. A square has been drawn on each side of the triangle. The length of each square is equal to the length of the side it is drawn on.

A square is added to each side of a right-angled triangle as shown in the above image. The sum of the the areas of the two smaller squares is equal to the area of the largest square. If the hypotenuse of the triangle has a length of $c$ and the other two sides are of length $a$ and $b$ then $a^2+b^2=c^2$

When the length of each side of the triangle is a positive integer, the three numbers make a Pythagorean triple. $(3, 4, 5)$ is the smallest triple with $3^2+4^2=5^2$. The Chinese text Chou Pei Suan Ching – original title Zhoubi – (周髀算经), (The Arithmetical Classic of the Gnomon and the Circular Paths of Heaven) gives this visual proof for the $(3, 4, 5)$ triple.

An image of a page from the Chinese text Chou Pei Suan Ching showing a visual proof for the triangle with sides $3, 4,$ and $5$ units — A visual proof for the Pythagorean triple $(3, 4, 5)$ from the Chinese text *Chou Pei Suan Ching*

$(3, 4, 5)$ is a primitive triple since $3, 4$ and $5$ are coprime – their only common divisor is $1$. $(n\times3, n\times4, n\times5), n = 2, 3, 4,…$ are part of the same ‘family’ and clearly not primitive triples. Another primitive triple is $(5, 12, 13)$ and an ordered sequence of hypotenuses for such triples are listed here. In the sequence I was surprised to see $185$ appearing twice. In fact there are exactly four distinct triples with hypotenuse $185$. With the aid of a spreadsheet, I was able to find them: $(57, 176, 185), (60, 175, 185),(104, 153, 185)$ and $(111, 148, 185)$

Generating Pythagorean triples

The sequence $\frac{1}{1},\frac{3}{2},\frac{7}{5},\frac{17}{12},\frac{41}{29},\frac{99}{70},\frac{239}{169},\frac{577}{408},\frac{1393}{985},…$ produces a Pythagorean triple from every other term beginning with $\frac{7}{5}$:

$\frac{7}{5}=\frac{3+4}{5} \rightarrow 3^2 + 4^2 = 5^2$

$\frac{41}{29}=\frac{20+21}{29} \rightarrow 20^2 + 21^2 = 29^2$

$\frac{239}{169}=\frac{119+120}{169} \rightarrow 119^2 + 120^2 = 169^2$

$\frac{1393}{985}=\frac{696+697}{985} \rightarrow 696^2 + 697^2 = 985^2$

…

As an added bonus, the decimal equivalent of each term of the sequence converges to $\sqrt2$, in a similar way the Fibonacci sequence converges to the golden ratio $\psi=\frac{1+\sqrt5}{2}$

Another method to find Pythagorean triples uses consecutive even numbers and the sum of their reciprocals as shown below.

Choose any pair of consecutive even numbers:

$8$ and $10$ $\rightarrow \frac{1}{8} + \frac{1}{10} = \frac{9}{40} \rightarrow 9^2 + 40^2 = 1681 = 41^2$

$12$ and $14$ $\rightarrow \frac{1}{12} + \frac{1}{14} = \frac{13}{84} \rightarrow 13^2 + 84^2 = 7225 = 85^2$

$20$ and $22$ $\rightarrow \frac{1}{20} + \frac{1}{22} = \frac{21}{220} \rightarrow 21^2 + 220^2 = 48841 = 221^2$

Euclid of Alexandria (325BC – 265BC) was a Greek mathematician who wrote a treatise, The Elements – a collection of 13 books. Books 1 to 6 are on plane geometry and books 7 to 9 on number theory. Euclid created a formula for generating Pythagorean triples from any pair of positive integers $m$ and $n$, where $m>n$:

($m^2-n^2, 2mn, m^2+n^2$) is the triple.

If $m=7$ and $n=3$, the triple is ($40, 42, 58$) $\rightarrow 40^2 + 42^2 = 3364 = 58^2$

If $m=89$ and $n=11$, the triple is ($7800, 1958, 8042$) $\rightarrow 7800^2 + 1958^2 = 64673764 = 8042^2$

A lovely link between Pascal, Fibonacci, Euclid and Pythagoras comes via any four consecutive Fibonacci numbers

$F_{1}$	$F_{2}$	$F_{3}$	$F_{4}$	$F_{5}$	$F_{6}$	$F_{7}$	$F_{8}$	$F_{9}$	$F_{10}$
$1$	$1$	$2$	$3$	$5$	$8$	$13$	$21$	$34$	$55$

The first 10 Fibonacci numbers

Using $3, 5, 8, 13$

Multiply the first and the last numbers $\rightarrow 3 \times{13}=39$
Multiply then double the middle two numbers $\rightarrow 5 \times{8}\times{2}=80$
Sum the squares of the middle two numbers $\rightarrow 5^{2}+8^{2}=89$
The Pythagorean triple is $(39, 80, 89) \rightarrow 39^{2}+80^{2}=7921=89^{2}$

$F_{1}$ to $F_{4}$ gives the primitive triple $(3, 4, 5)$

$F_{2}$ to $F_{5}$ gives the primitive triple $(5, 12, 13)$

$F_{3}$ to $F_{6}$ gives the triple $(16, 30, 34)$

$F_{7}$ to $F_{10}$ gives the primitive triple $(715, 1428, 1597)$

In addition, for any set of four consecutive Fibonacci numbers, $F_{7}$ to $F_{10}$ for example, the following connection is true

$7+10=17$ and the $17^{th}$ Fibonacci number is $1597$, the third member of the triple, the hypotenuse of the triangle!

Amazingly, if we use $m$ and $n$ as consecutive Fibonacci numbers when using Euclid’s method, then the last number of the triple is again a Fibonacci number

$m$	$n$	Triple	Fibonacci number
$2$	$1$	$(3, 4, 5)$	$5^{th}$
$3$	$2$	$(5, 12, 13)$	$7^{th}$
$5$	$3$	$(16, 30, 34)$	$9^{th}$
$8$	$5$	$(39, 80, 89)$	$11^{th}$
$13$	$8$	$(105, 208, 233)$	$13^{th}$

Another pattern is created with the the sequence of odd numbers $5, 7, 9, 11, 13,…$ The $15^{th}$ Fibonacci number is $610$, the hypotenuse when $m=21$ and $n=13$

A visual proof of the theorem

I first came across a visual proof of Pythagoras’ theorem for all right-angled triangles in Roger B. Nelsen’s wonderful book Proofs Without Words, Exercises in Visual Thinking. Nelsen noted the proof (author unknown, circa B.C. 200?) is adapted from the Chou Pei Suan Ching. The two images below combine to show the proof:

Two side by side multi-coloured squares demonstrating a visual proof of Pythagoras' theorem for all right-angled triangles. The proof is realised when the four right-angled triangles inside one square are placed inside the other square in different positions. — A visual proof of Pythagoras’ theorem for all right-angled triangles

A proof by trapezium area and an untimely painful death

James Garfield (November 19, 1831 – September 19, 1881) was elected as the United States’ 20th President in 1880. He was assassinated after just 200 days in office after being shot on July 2, 1881, in a Washington railroad station. Garfield remained mortally wounded in the White House for many weeks where Alexander Graham Bell, inventor of the telephone, attempted to locate the bullet with an induction-balance electrical device which he had designed. Bell and physicians were unsuccessful in their attempts and Garfield died from an infection and an internal haemorrhage on September 19, 1881.

An engraving of the moment in a Washington railroad station when President Garfield is being shot. — Garfield, shot by Charles J. Guiteau, collapses as Secretary of State Blaine gestures for help. Engraving from *Frank Leslie’s Illustrated Newspaper*.

In 1876, Garfield had an elegant proof of Pythagoras’ theorem published. The proof makes use of the formulae for the areas of a triangle and a trapezium. The proof is demonstrated in the image below

Three triangles are arranged to form a trapezium. The sum of the areas of the triangles are equated with the area of the trapezium to prove Pythagoras' theorem.

A proof using the Shoelace formula

The brilliant German mathematician Johann Carl Friedrich Gauss (30 April 1777 – 23 February 1855) developed a formula to calculate the area of a polygon if every vertex of the polygon lies on a known Cartesian coordinate. The formula is widely known as the Shoelace formula and makes use of the calculation to find the determinant of a 2 by 2 matrix.

Text reads: "An example of the algorithm for the Shoelace formula
Start and finish with any coordinate and work anticlockwise
Multiply and then add the numbers at the end of the blue arrows
Multiply and then add the numbers at the end of the red arrows
Subtract the red answer from the blue answer
Halve the result to obtain the polygon area"
Below this, the coordinates of of a pentagon are displayed on a cartesian grid. Gauss' shoelace formula is used to calculate the area of the pentagon.

John Molokach observed that the Pythagorean theorem follows from Gauss’ Shoelace Formula, as shown below

A square with coordinates $(a, 0), (a+b, a), (b, a+b), (0, b)$ is shown. Pythagoras' theorem is proved using the shoelace formula.

It’s not just squares

If the same regular $n$-gon, $n$ = 3, 4, 5,.. is drawn on each side of a right-angled triangle, then the sum of the areas of the two smaller $n$–gons equals the area of the $n$–gon on the hypotenuse. Semicircles also produce the same result. As $n\rightarrow \infty$, a regular $n$-gon approaches a circle and so circles, where the sides of the triangle act as tangents to the circles can be said to satisfy Pythagoras’ theorem. The same result can be obtained by rotating the semicircles through $180^ \circ$ and adding matching semicircles.

Regular hexagons are added to each side of a right-angled triangle. The exact area of each hexagon, written in surd notation, is displayed inside each hexagon. The sum of the two smaller hexagon areas is equal to the area of the hexagon on the hypotenuse of the triangle. — Three regular hexagons drawn on a $3, 4, 5$ triangle with $\frac{27\sqrt3}{2}+\frac{48\sqrt3}{2}=\frac{75\sqrt3}{2}$

The spiral of Theodorus of Cyrene

When $n$ is not a square number, $\sqrt{n}$ is called a surd

Pythagoras and his followers, the Pythagoreans, believed that the universe can be explained by whole numbers and the ratio of whole numbers. Their moto – “All is number” – was carved above the entrance of their meeting place. However, a spanner was thrown in the works when Hippasus of Metapontum, one of the Pythagoreans suggested that $\sqrt2$, the length of the hypotenuse of the right-angled triangle with sides $1, 1$ and $\sqrt2$, could not be written as the ratio of two whole numbers. Such numbers are now called irrational and it was approximately 200 years before Euclid of Alexandria proved $\sqrt2$ was irrational. The Pythagoreans were sworn to secrecy and one legend suggests that Hippasus was thrown off a boat and drowned for revealing his discovery to non-Pythagoreans. Then again, some believe Hippasus drowned because he revealed how to construct a dodecahedron inside a sphere. The ($1, 1$,$\sqrt2$) triangle is the start of the spiral of Theodorus. Further right-angled triangles, each containing a side of length $1$ unit, are added sequentially to produce the sequence $\sqrt2, \sqrt3, \sqrt4, \sqrt5,…$. The sequence is the length of each new hypotenuse, as shown in the diagram below. Theodorus probably stopped at $\sqrt17$ as it the length of the hypotenuse of the triangle before the triangles begin to overlap. The spiral can be expanded here.

The Aperiodical