All Squared, Number 9: Miscellanea with CP and Cushing

We have an unusual All Squared podcast for you this time. My good friend David Cushing has been asking to do a podcast for absolutely ages. We couldn’t decide on a single topic to talk about, so instead I suggested we just sit down and chat about maths in general, like we do when there isn’t a microphone in front of us.

We talked for about an hour and a half, but because I’m completely stupid we lost a big chunk of it when the microphone switched off. To make things even worse, we recorded in a room with a ridiculously loud fan, so there’s that to contend with. Anyway, we talked about some fun stuff, so I think it’s worth listening to.

Here are some links relevant to the things we talked about.

David would like you to know that $5 \times 16017 = 80085$.
The book David brought was Topology, by James Munkres.
Brouwer’s fixed-point theorem says that for any continuous function $f$ with certain properties mapping a compact convex set into itself there is a point $x_0$ such that $f(x_0) = x_0$.
The pancake theorem is referred to by MathWorld as “a two-dimensional version of the ham sandwich theorem”, so CP wins. The ham sandwich theorem says that the volumes of any $n$ $n$-dimensional solids can be simultaneously bisected by an $(n-1)$-dimensional hyperplane.
The hairy ball theorem says that there is no nonvanishing continuous tangent vector field on even-dimensional $n$-spheres – there’s always a point on the sphere where the function is zero.
The black hole information paradox says that it’s possible for a black hole to destroy information. In 2004 Stephen Hawking conceded his bet that information is destroyed, so CP wins again. (Guess who’s writing this summary)
The book CP brought was Only Problems, Not Solutions by Florentin Smarandache. It turns out he’s a bit of a character!
The family of sequences which contains a sequence for each digit, except inexplicably 1, was “Primes with $n$ consecutive digits beginning with the digit $D$”
Problem 102 of Only Problems… contained this cool diagram:
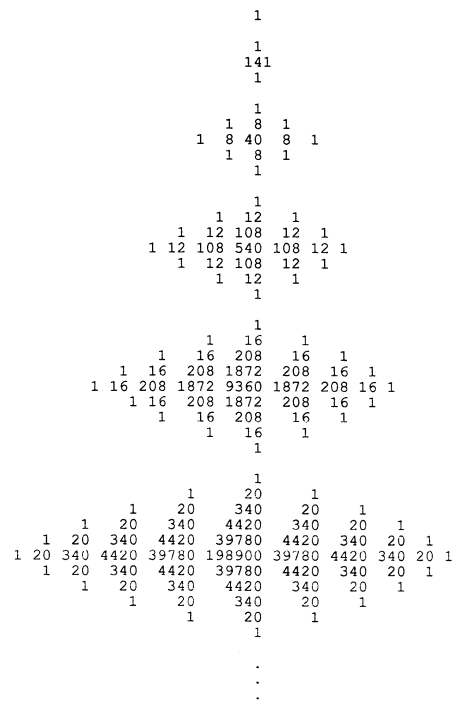
We can’t remember what “the Russian book” was. Sorry!
The powerful numbers are sequence A060355 in the OEIS.
Paul Erdős made a conjecture on arithmetic progressions.
The Bee Gees consisted of brothers Barry, Robin, and Maurice Gibb. That’s three people: a powerful triple.
$x^2 – 8y^2 = 1$ is a Pell equation, and the reason why the continued fraction representation of $\sqrt{8}$ generates consecutive pairs of powerful numbers.
The Muddy Children puzzle is a good introduction to public announcement logic. The slides we were looking at were “The Muddy Children: a logic for public announcement”, by Jesse Hughes.
Analysis vs Algebra predicts eating corn?
David was playing Wuzzit Trouble by InnerTube Games. It was reviewed here by Colin Beveridge last month.