The MathsJam conference has a baking competition. My friend the archaeologist Stephen O’Brien tweeted a while ago a link to a fun blog post ‘Edible Archaeology: Gingerbread Cuneiform Tablets‘. Babylonian tablets are among the earliest written evidence of mathematics that we have, and were produced by pressing a stylus into wet clay.

So it was that I realised I could enter some Babylonian-style tablets made from gingerbread.

I made a gingerbread reconstruction of a particular tablet, YBC 7289, which Bill Casselman calls “one of the very oldest mathematical diagrams extant“. Bill writes about the notation on the tablet and explains how it shows an approximation for the square root of two. I’m sure I didn’t copy the notation well, because I am just copying marks rather than understanding what I’m writing. I also tried to copy the lines and damage to the tablet. Anyway, here is my effort:

In addition, I used the rest of the dough to make some cuneiform biscuits. I tried to copy characters from Plimpton 322, a Babylonian tablet thought to contain a list of Pythagorean triples. Again, Bill Casselman has some interesting information on Plimpton 322.

Below, I try to give a description of my method.

Making the tablets

I tried the recipe given but I don’t know if I mistranslated the American cups measurements, but it came out too dry, so I adjusted the ingredients and tried again before making it the third time for real. Below is a description of what I did.

The idea here is to make a gingerbread that tastes good but crucially has the stiffness to take the cuneiform writing and that doesn’t rise much so the writing stays in place while it cooks. There’s a lot of chilling to harden the dough. I should mention that you make the dough one day and cook it the next.

Ingredients:

• 500g plain white flour;
• 110g soft brown sugar;
• 160g butter;
• 145g treacle;
• 1 medium egg;
• 1 teaspoon bicarbonate of soda;
• 2 teaspoons ground ginger powder;
• half teaspoon cinnamon;
• half teaspoon nutmeg;
• half teaspoon salt.

Method:

Day one, mix the dough.

1. Weigh the butter and cut up into lumps, leave on a plate to warm up for at least 20 minutes.
2. Mix the dry ingredients in a bowl.
3. Add the butter and mix up.
4. Add the treacle and egg and mix well. I used a food processor. It made a stiff dough.
5. Compress into a bowl, cover with cling film and put in the fridge overnight.

Day two, make the gingerbread.

1. Roll out the dough. I made a big tablet and a set of little biscuits. I put a tea towel on the side with greaseproof paper on top, and rolled on this using a rolling pin and some flour. The dough is quite happy to be pushed around and cut up.
2. Put the rolled out dough on greaseproof paper on baking sheets and put it in the fridge for half an hour to re-stiffen.
3. Cuneiform writing using a square handle of a chopstick. The linked blog post has a lot on this, including a nice video demonstration of the technique. I bought some cheap chopsticks online, and while the handles were square, they were a bit too rounded. This meant the impressions made in the gingerbread were not very clear, especially after baking. My parents had a sharper-handled chopstick and I was able to use this for the real thing. So it is important to get the right tool. I also used a blunt knife for making lines and a teaspoon for scraping out sections of the tablet I was copying that were broken.
   
4. After scribing, refrigerate again while heating up the oven.
   
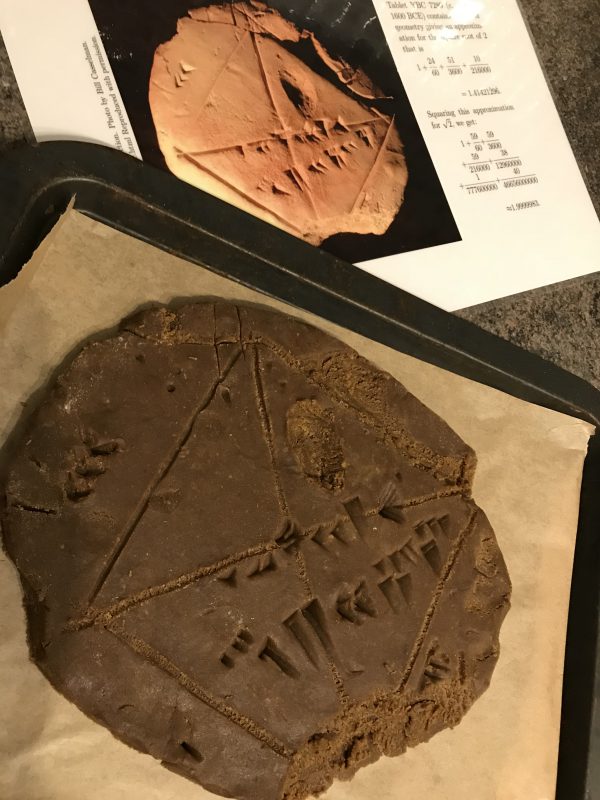
5. I cooked mine at gas mark 4 for 10 minutes (biscuits) or 12 minutes (large tablet). Our oven is a bit quirky, though, so you might want to experiment. The first go I over-cooked them a little.

Probably by now you are firmly of the view that I am not a recipe writer! There is a lot more detail in the original blog post and I recommend reading this and watching the short video before starting.

I am grateful to Farrell Monaco for sharing her cuneiform gingerbread recipe, and to Bill Casselman for his pages on Babylonian mathematics tablets.