I found Joe Bartholomew’s blog while googling for a fact to do with the 42-gon. In Girih Extended he elaborates on the Islamic girih patterns, which are constructed from translations and rotations of a few polygons, by adding scaled polygons.
In Girih Seven, he used polygons whose internal angles are multiples of $\frac{\pi}{7}$ instead of the $\frac{\pi}{5}$ used in traditional girih.
I didn’t find the fact I was looking for, but I did find a different one: according to Joe, the 42-gon is also called the tetracontakaidigon.
Site: Joe Bartholomew
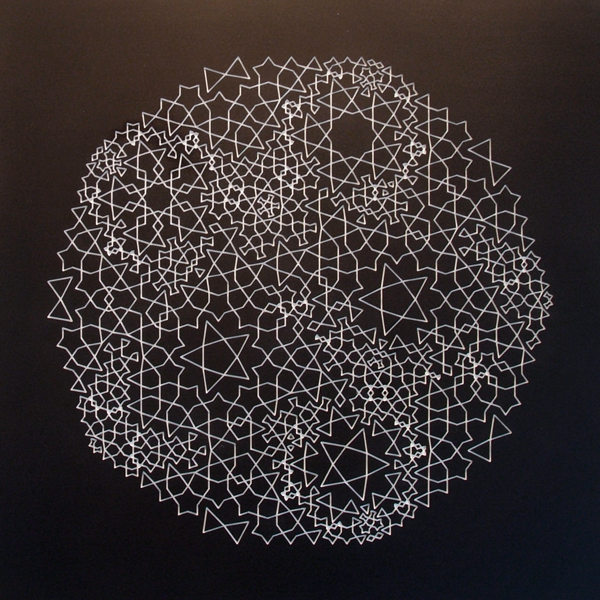
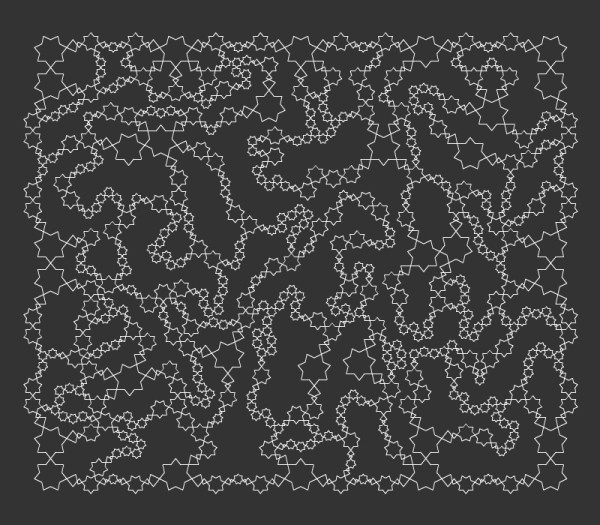
The regular 42-gon is constructible by origami, since it is expressible as a product of a power of two, a power of three, and a product of distinct Pierpont primes.