Here’s a roundup of mathematical things that have happened in March 2022.
You're reading: Posts By Christian Lawson-Perfect
- American Mathematical Society
- London Mathematical Society, UK
- Institute of Mathematics and its Applications, UK
- Société Mathématique de France
- Australian Mathematical Society
- Canadian Mathematics Society
Now I’m calculating with constructive reals!
A while ago I made myself a calculator. I don’t know if anyone else uses it, but for the particular way I like doing calculations, it’s been really good. You’d think that if a calculator does anything, it should perform calculations correctly. But all calculators get things wrong sometimes! This is the story of how I made my calculator a bit more correct, using constructive real arithmetic.
One thing you need to think about when making a calculator is precision. How precise do the answers need to be? Is it OK to do rounding? If you do round, then it’s possible that errors accumulate as you compose operations.
I’ve always wanted to make a calculator that gives exactly correct answers. This isn’t strictly possible: there are more real numbers than a finite number of bits of memory can represent, or a digital display can show, no matter how you encode them. But I’m not going to use every real number, so I’ll be happy with just being correct on the numbers I’m likely to encounter.
Each Edge Peach Pear Plum
At the 2021 UK MathsJam Gathering, I gave a talk on a subject that has bothered me more than is reasonable: the graph-theoretic layout of the narrative of the baby’s book Each Peach Pear Plum, by Janet and Allan Ahlberg.
It’s one of my son’s favourite books to fall asleep to. It was his older sister’s favourite, and mine and my wife’s when we were little. I agree with the quote on the back cover, that it’s “the perfect first book”.
BUT
Aperiodical News Roundup – February 2022
Here’s a roundup of mathematical things that have happened in February 2022.
Ukraine
The deeply troubling and developing situation in Ukraine has implications for the 2022 International Congress of Mathematicians (ICM) due to take place in St. Petersburg, Russia in July. A group of Ukrainian mathematicians has issued a call for mathematicians to boycott the event. National organisations around the world have been issuing statements setting out their positions, standing down their participation and calling on the International Mathematical Union to not hold the event as planned. Here are some we spotted:
The International Mathematical Union (IMU) itself wrote to its member organisations expressing its deep concern, acknowledging the calls and saying it is assessing the situation.
Other news
The organisers of the Gathering 4 Gardner recreational maths conference have announced that this year’s event, taking place in April, will be a hybrid event with 50% discount for online-only places, making them a snip at $200. Registration is restricted to previous attendees and invitees, but it is possible to nominate yourself or someone else for an invitation.
Casualties of the recent storms in the UK apparently also include Newton’s apple tree – not the actual tree an apple fell on his head from, but scions of the original are planted all over the UK and one of the ones at Cambridge, which was planted in 1954, hasn’t survived the combined effects of Storm Eunice and gravity. More info in this excellent Twitter thread.
The Royal Statistical Society has released a report entitled Behind the numbers: The RSS puts the statistical skills of MPs to the test, in which they report the results of asking an anonymous unspecified group of Labour and Conservative MPs a series of simple stats and probability questions. The survey concluded that while MPs performed better than they did in a similar test ten years ago, their stats skills were still sub-par. It may not be as unambiguous as the research seems to claim though – Rob Eastaway has thoughts about the questions used.
Prizes

Canadian number theorist Dr. Matilde Lalín is to receive the Krieger-Nelson prize, awarded since 1995 by the Canadian Mathematical Society to recognise outstanding contributions in the area of mathematical research by a female mathematician. (via Jordan Ellenberg)

The winners of the 2022 Mathical book prize, an annual award for fiction and nonfiction books that inspire children of all ages to see maths in the world around them, have been announced. The winners look to include some lovely titles, including Maryam’s Magic – the story of mathematician Maryam Mirzakhani – and the fantastic-sounding Uma Wimple Charts Her House. (via Jordan Ellenberg)
And finally
If you like that kind of thing, you can buy a bunch of cheap maths puzzle book PDFs in a Humble Bundle (via Adam Atkinson). And if you like proof assistants, there’s now a Proof Assistants Stack Exchange.
This island is big enough for the both of us
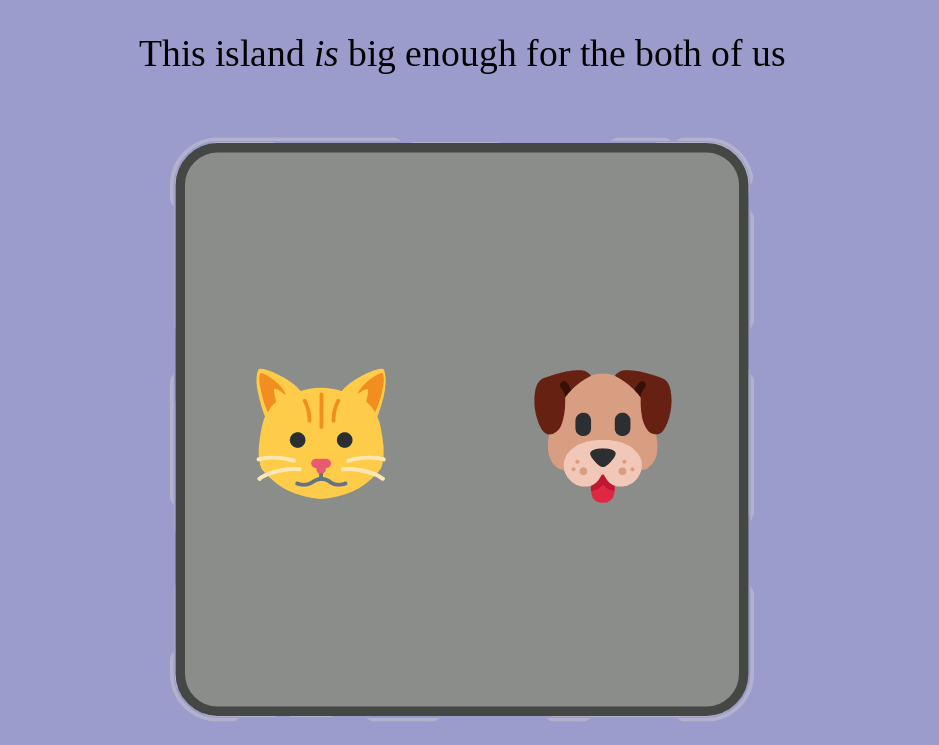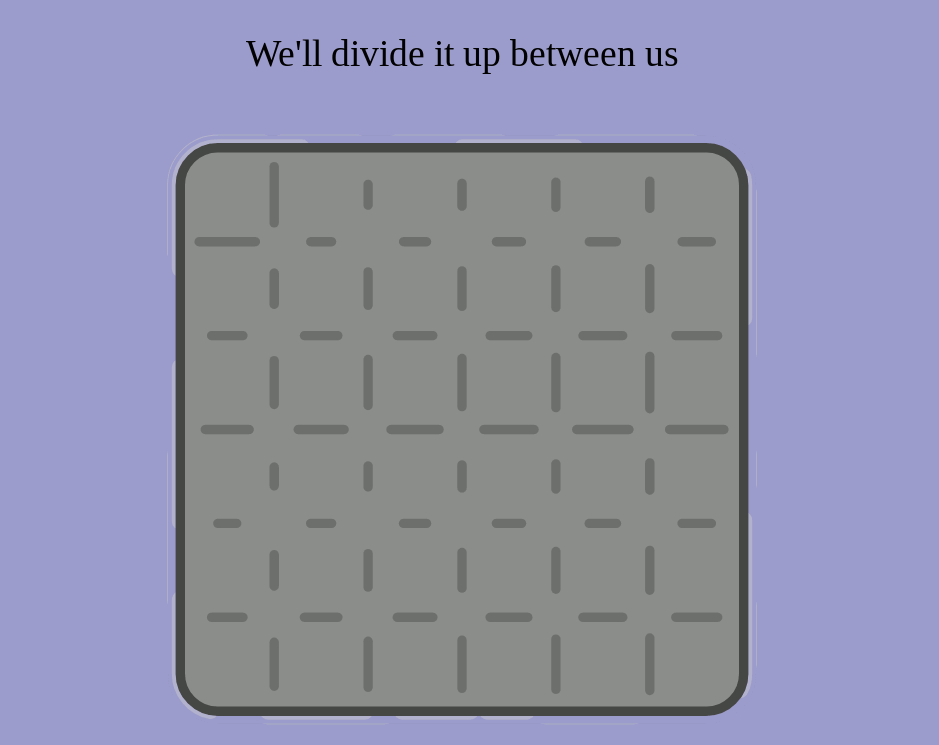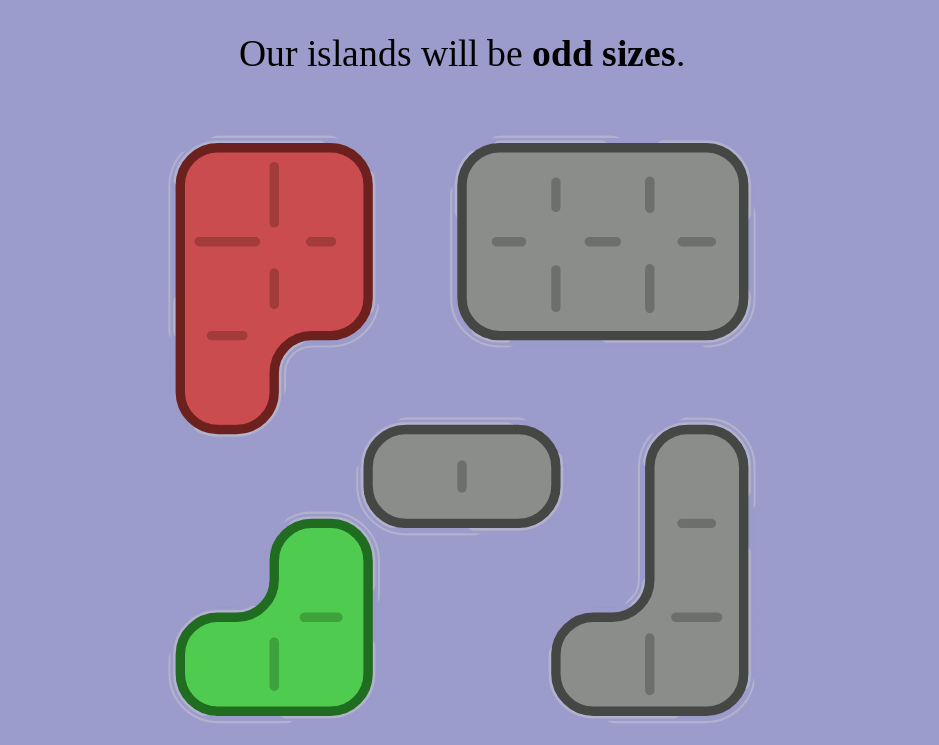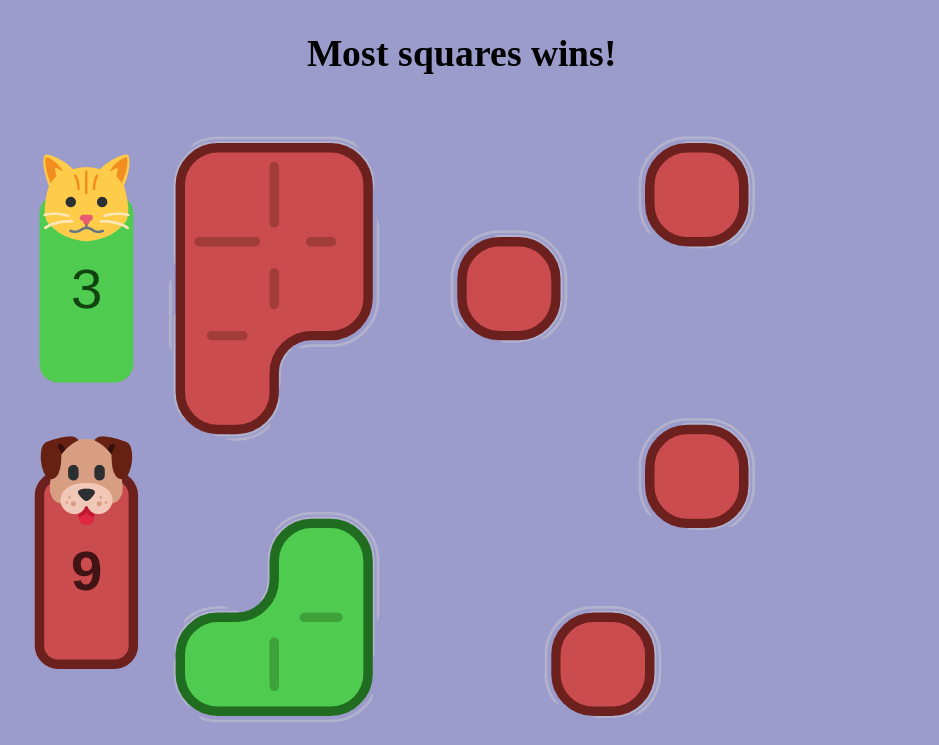
I’ve made a little game.
Mathematicians on TV at Christmas, 2021
A surprising number of mathematicians (including some friends of the Aperiodical) have been on UK telly this Christmas!
Nira Chamberlain on University Challenge
Winner of the Big Internet Math-Off 2018, Nira Chamberlain, captained the Portsmouth University team in an episode of the University Challenge Christmas special (on iPlayer, and the episode on YouTube). We’re pleased to see that since his Math-off victory, Nira continues to introduce himself wherever he goes as ‘The World’s Most Interesting Mathematician’.
Paul and Katie on Only Connect again
Aperiodical editor Katie Steckles and site regular Paul Taylor, accompanied by fellow mathematician Ali Lloyd, appeared in the Only Connect Champion of Champions special as part of their team the Puzzle Hunters. Having won series 16 this year, they were invited back to take on the winners of series 15, the 007s. Watch out for at least one maths question! The episode is on iPlayer, and on YouTube.
Ri Christmas Lectures
This year’s lectures were on the science behind virology and the pandemic, and hosted by Professor Jonathan Van-Tam. As part of the second episode, mathematician Professor Julia Gog joined to explain how mathematical modelling can be used to study the spread of viruses, around 38 minutes in. (Slightly worryingly, JVT claims he isn’t any good at maths, so he had to get someone in to help explain it).
All three episodes are available on iPlayer, and will be added to the Ri YouTube channel once they come off there (around the beginning of Feb).
Katie shows you how to make a kumihimo braid
The Queen Elizabeth Prize for Engineering and Alom Shaha have organised a Month of Making – each day, a different person shows how to make a nice handmade Christmas gift.
Today it’s our own Katie’s turn. Her gift is a simplified version of a kumihimo, a Japanese form of braid.
There’s a little bit more information on the QE Prize site.


