The Online Encyclopedia of Integer Sequences contains over 200,000 sequences. It contains classics, curios, thousands of derivatives entered purely for completeness’s sake, short sequences whose completion would be a huge mathematical achievement, and some entries which are just downright silly.

For a lark, David and I have decided to review some of the Encyclopedia’s sequences. We’re rating sequences on four axes: Novelty, Aesthetics, Explicability and Completeness.

A000959
Lucky numbers.

1, 3, 7, 9, 13, 15, 21, 25, 31, 33, 37, 43, 49, 51, 63, 67, 69, 73, 75, 79, 87, 93, 99, 105, 111, 115, 127, 129, 133, 135, 141, 151, 159, 163, 169, 171, 189, 193, 195, 201, 205, 211, 219, 223, 231, 235, 237, 241, 259, 261, 267, 273, 283, 285, 289, 297, 303, ...

Explicability

Christian: Explain.

David: It’s like the primes but it isn’t.

Christian: That isn’t enough explanation. 

David: It’s like that guy’s sieve, but it isn’t.

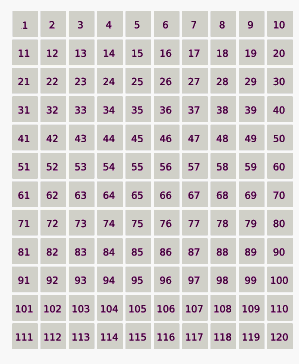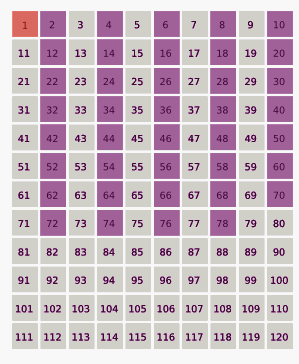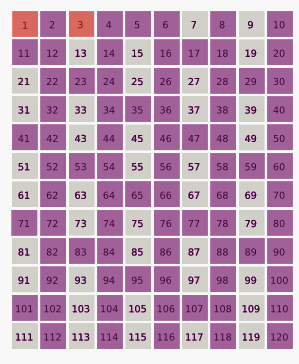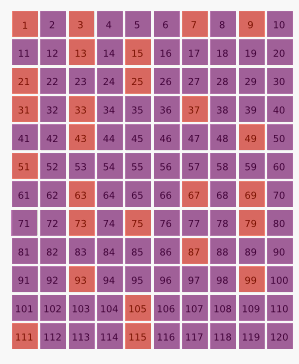
Christian: Sheesh. It’s the natural numbers, with every other number removed, then every third number, then….

David: Whoah whoah whoah. Not quite. Write out the natural numbers. Look at the first non-one term, which is 2. Delete every second term. Look at the next non-one term we haven’t already looked at. Which is 3. Delete every third term which has not yet been deleted. That’s the important bit.

Christian: Repeat?

David: Yes.

Christian: Well, I sort of remembered it. It’s fiddly. Three?

David: Yep, three’s there.

Christian: I mean the score.

\[ \frac{3}{5} \]

Aesthetics

David: Looks like the primes, so no obvious pattern. It has some good-looking facts though.

Christian: Such as?

David: It has its own prime number theorem. And it has a Goldbach conjecture – known as the “I should be so lucky” conjecture.

Christian: Is that true?

David: No…. BUT! But, Perfect! I challenge you to express 200 as the sum of two lucky numbers.

Christian: Lemme have a look… $193+7$.

David: A million and eight.

Christian: The OEIS doesn’t give numbers that big. Talking of big numbers: score?

David: FIVE!

…

David: BILLION!

Christian: Let’s just take the five.

\[ \frac{5}{5} \]

Novelty

Christian: At least it isn’t the primes. I quite like it.

David: I forgot something. I have a complaint about its aesthetics.

Christian: We just did aesthetics!

David: But the one is ugly! This does have relevance to the novelty. What if we don’t include the 1 and delete every second number, so 2 stays and 3 doesn’t?

Christian: Then we get… A045954: the even-lucky numbers.

David: What if we don’t have 1 or 2?

Christian: Where’s this going?

David: It isn’t novel. Or at least, not five points of novel.

Christian: What would we give a five for novelty?

David: Didn’t we give the all 7s sequence a five?

Christian: So we’re picking at random?

David: No…

\[ \frac{4}{5} \]

Completeness

David: How many do we know?

Christian: If the last four reviews have taught us anything, it’s that that is a terrible way of assigning a completeness score.

David: Fine, we know enough to appreciate the asymptotic behaviour. Five.

Christian: Not going to argue with that.

\[ \frac{5}{5} \]

Total Score

\[ \frac{3+5+4+5}{20} = \frac{17}{20} \]

Christian: Our first score which doesn’t cancel!

David: I think it should lose one for that.

\[ \frac{17-1}{20} = \frac{16}{20} = \frac{4}{5} \]

Christian: I disagree. Give one back.

\[ \frac{4+1}{5} = \frac{5}{5} = 1 \]

David: A perfect score!

…

David: Can we have a picture of Kylie Minogue?