Note: If you’re looking for instructions on solving Rubik’s cube from any position, there’s a good page at Think Maths.
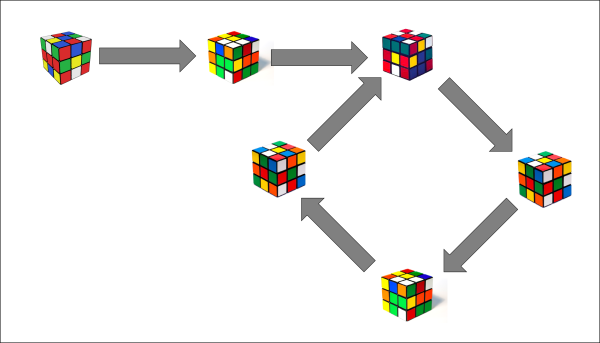
One day some years ago I was sat at my desk idly toying with the office Rubik’s cube. Not attempting to solve it, I was just doing the same moves again and again. Particularly I was rotating one face a quarter-turn then rotating the whole cube by an orthogonal quarter-turn like this:
Having started with a solved cube, I knew eventually if I kept doing the same thing the cube would solve itself. But this didn’t seem to be happening – and I’d been doing this for some time by now. This seemed worthy of proper investigation.
The first thing to sort out is, how did I know the cube would eventually solve itself if I kept doing this same thing over again? Well, a pretty obvious fact about a Rubik’s cube its that it’s non-random. If two cubes are in the same state and you do the same moves on both, they’ll both end up in the same state. The initial state and the moves together determine the end state. So as you repeat the same sequence of moves, the cube tracks through different states in a predetermined way. There’s a finite number of states the cube can be in (rather more than the 3 billion that the product’s marketing originally claimed but still a finite number) so eventually it will hit a state it’s been in before. From then the cube will be stuck Groundhog-Day-style in its previous pattern; the same states and the same moves producing the same results.
But this doesn’t prove that the cube will return to its initial solved state. Why could the following not happen?
If the cube doesn’t go back to a solved state, it must do this, landing on some previously seen non-solved position and from there entering a loop. But look at the first cube in the loop. It’s reached from two different states. Another pretty obvious fact about the Rubik’s cube is that any move, or sequence of moves, can be reversed. If I can twist a face then spin the cube, I can just as well spin the cube the other way then twist it backwards. Doing a move then doing its inverse will have no net effect on its state. So suppose the cube is in one of the two states feeding in to the loop, and I do my twist-and-spin move combo then its spin-and-twist inverse. In both cases we must end up with the cube as it started. But both start by twist-and-spinning the cube into the first state of the loop. But then we have the curious situation where both cases involve having a cube in this state, both have the spin-and-twist ‘undo’ moves applied, but both end up in different states. We know this doesn’t happen. The only way to reconcile this paradox is if the loop goes right back to the initial solved state, so that the two-into-one problem does not arise.
Compare this state of affairs to Conway’s Life, the ‘zero-player game’ wherein a grid of black and white squares changes over time according to a simple rule that can give rise to almost endless variety. Given an initial state for Life and its one repeated ‘move’, what can (and very often does) happen is that the game gets locked into an infinitely-repeating pattern that does not begin with a repetition of the initial game state. That’s because the move in Life is not invertible; you can reach the same position from two different previous states, so the ‘lasso’ pattern above does occur.
Now we know I will eventually ‘solve’ the Rubik’s cube with my twist-spin moves, but the question remains when? Since my office was in the maths department at Manchester University, we decided to work it out, and do it the only way we knew how: with stickers and computers.
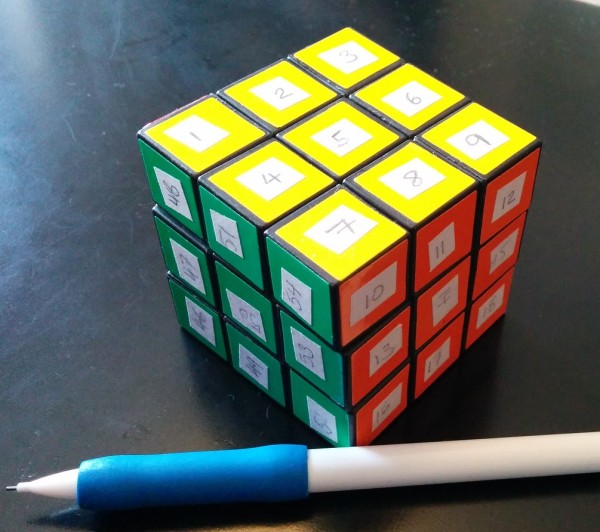
With the little ‘facelets’ of the cube numbered 1 to 54, we can track the effect of a move on the cube by seeing how the numbers swap places. Doing my quarter-turn we see that facelet 1 goes to the position previously occupied by facelet 37, facelet 2 goes to the position previously occupied by facelet 38, and so on. When doing serious maths, to avoid constantly typing the phrase “goes to the position previously occupied by”, we might write this as
\[ \scriptsize{\left( \begin{array}{ccccccccccccccccccccc}
1&2&3&37&38&39&27&26&25&46&47&48&34&36&30&28&35&33&29&31\\
\downarrow&\downarrow&\downarrow&\downarrow&\downarrow&\downarrow&\downarrow&\downarrow&\downarrow&\downarrow&\downarrow&\downarrow&\downarrow&\downarrow&\downarrow&\downarrow&\downarrow&\downarrow&\downarrow&\downarrow\\
37&38&39&27&26&25&46&47&48&1&2&3&36&30&28&34&33&29&31&35\end{array}\right).} \]
If we’re feeling particularly terse (we always are) we might write that even more compactly as
\[ (1\ 37\ 27\ 46)(2\ 38\ 26\ 47)(3\ 39\ 25\ 48)(34\ 36\ 30\ 28)(35\ 33\ 29\ 31) \]
Here we start with $1$, which goes to the position previously occupied by $37$, so we look at $37$ next, and so on. If we get back to the number we start with we put brackets round that cycle and start again with the smallest number we haven’t looked at yet. (We know this will form separate tidy cycles for basically the same reason we know the states of the cube form a cycle.)
So we got the stickered cube, squinted at a number, twisted or turned the cube and worked out whose position that number took over. Doing so we arrived at the cycles for both the twist and the spin:
\begin{align} \mathrm{twist} &= (1\ 37\ 27\ 46)(2\ 38\ 26\ 47)(3\ 39\ 25\ 48)(34\ 36\ 30\ 28)(35\ 33\ 29\ 31) \end{align} \begin{align} \mathrm{spin} &= (1\ 3\ 9\ 7)(2\ 6\ 8\ 4)(25\ 27\ 21\ 19)(20\ 22\ 26\ 24) (12\ 54\ 34\ 37)(11\ 51\ 35\ 40)(10\ 48\ 36\ 43)(13\ 47\ 33\ 44)(14\ 50\ 32\ 41) (15\ 53\ 31\ 38)(16\ 46\ 30\ 45)(17\ 49\ 29\ 24)(18\ 52\ 28\ 39). \end{align}
The twist moves four strips of three numbers round in little cycles of four, and the eight outer squares on the twisted face move in two more cycles of four. The spin has a more far-reaching effect, changing the position of every sticker except the two at the ‘poles’ of the rotation, $5$ and $23$.
Once you have two moves written down in this way, it’s easy to see the effect of one move followed by another: you just work out what happens to each number in turn. So the first move takes $1$ to the original position of some other number, call it $n$ ($n$ itself has of course also moved elsewhere). Where does the second move now take $1$? Well we know where it takes $n$ on the solved cube, and we know $1$ is now in that position, so the move now takes $1$ to whatever position it takes $n$ to in the solved cube. Once we’ve done this for every number,we’ll have written down the combined twist-and-spin move in the same form as above.
From this we can work out how many times it would need to be repeated to get back to the start. This last step shows the power of the cycle-based notation. A single cycle of length $n$ just needs to be repeated $n$ times so that every number shuffles back to its original position. And since none of the cycles interfere with each other, the number for a whole move is just the smallest number that’s a multiple of the lengths of all the cycles. Once we’ve found this, we’ll have the number of repeats needed to get the cube back to solved. What’s more, we’ll have worked it out without even having to actually do the two moves over after the other, let alone repeat them again and again. It doesn’t matter how ridiculously high the number turns out to be, we can get the answer with the same moderate amount of effort.
Although, as I’ve explained, it’s relatively simple to work out the number of repeats needed from this information, needing one slightly laborious calculation to get the sequence for the combined twist-spin, and a quick comparison of the lengths of the cycles, we instead plugged the cycles into a computer program. We used MAGMA, which is designed for doing calculations in the areas of maths that this is a pretty simplistic example of. You can try this yourself by pasting the below code into the online MAGMA calculator:
G:=Sym(54);
twist:=G!((1,37,27,46)(2,38,26,47)(3,39,25,48)(34,36,30,28)(35,33,29,31));
print "Order of twist";
Order(twist);
spin:=G!((1,3,9,7)(2,6,8,4)(25,27,21,19)(20,22,26,24)(12,54,34,37)(11,51,35,40)(10,48,36,43)(13,47,33,44)(14,50,32,41)(15,53,31,38)(16,46,30,45)(17,49,29,42)(18,52,28,39));
print "Order of spin";
Order(spin);
print "Order of twist*spin";
Order(twist*spin);
Plugging this into the computer gives the answer, which is 1,260. If you were doing serious maths you would call this the ‘order’ of the move sequence, as you can see from the name of the command used above. I find it pretty astonishing that two moves that individually each only need to be done four times to return to the initial state can get that much bigger when combined. This raises the obvious question: what’s the highest number of times that any sequence of moves would have to be repeated to return the cube to its initial state? Well we can find that out too, but first: a technicality!
One of my moves was to rotate the whole cube a quarter-turn. Well, some people might argue that that isn’t a proper move – I didn’t really change the cube. I may as well have sat it on a table and myself walked round to a different side. These purists would rewrite my two-move sequence as a four-move one: twist the front face, then the right face, then the back face then the left face. This four-move sequence still takes 1,260 twists to solve itself, but that’s now ‘just’ 315 repeats of the sequence. (A technicality within a technicality: if I’m counting rotating the whole cube as a single move, do I count a solved but rotated cube as being back in its initial state? I guess I shouldn’t – luckily this doesn’t arise with my sequence of moves. But probably best to stick with the purists’ view from now on.) It’s a little disappointing that we’ve had to drop from the impressive-sounding 1260 to a mere 315, but in our new system we can more easily determine the highest number of repeats possible.
If you’re going to tackle a question like this, it helps to have some notation worked out so you can talk about things like “a quarter twist of the front face clockwise and then a quarter twist of the back face anticlockwise by which I mean anticlockwise if you were round the back looking at it which is sort of clockwise when you do it by reaching round from the front” without needing to go and have a lie down. Luckily, many of the first people to seriously tackle Rubik’s cubes were mathematicians, so this is not a problem. The standard notation is now to use the letters $F$, $R$, $L$, $B$, $U$ and $D$ to denote a quarter twist of the front, right, left, back, top (up) and bottom (down) faces respectively (clockwise from the point of view of someone looking straight on at that face). Sequences of moves are just written as the corresponding sequence of letters, so my move was $FRBL$. A half-turn of, say, the front face can be written $FF$ (since it’s just two quarter turns) or more snappily as $F^2$. Since a quarter-turn anticlockwise is three clockwise quarter-twists, and is also the inverse of the clockwise quarter-twist, we can write it as $FFF$, $F^3$ or $F^{-1}$ (for “the inverse of $F$”).
The six lettered twists comprise a complete basic set of Rubik’s cube moves: everything you can do on the cube can be considered to be a sequence of these six moves. So if we tell a computer the permutations for these six moves, and if our computer is smart enough, it can tell us what combination of these has the longest ‘repeat time’. This is trickier than I’ve made it sound: effectively you have to work out the entire structure of the group of all sequences of moves and how they interlock. But if you do this, you find that the most repetitions any sequence needs is a familiar number: 1,260. One such move consists of five twists of various faces (four quarter-turns and one half-turn), written $RU^2D^{-1}BD^{-1}$. At a couple of seconds per twist, doing this sequence would take three-and-a-half hours to solve the cube. Still, this move is a little elaborate, and the random move-sequence I tried got pretty far up the scale of futility. In fact 315 is the best you can do with a sequence of up to four of the basic moves, so I claim a small victory for idly toying with objects as a means of uncovering some of their deeper mathematical structure.
I did not understand anything i am only 11 years old
i know right this too hard i am only nine
Me too
ohh
I could not understand the last part
well, obviously this is’nt meant for children whatsoever.
I’m 23 and I didn’t get it.
I’m 55 i also did not understand
ex-actly whoever did this must hate us kids
I am an 11 year old and I understand but I was too lazy to read through IT
same here
I don’t know this.
I can solve a rubicks cube and I’m ten and I get it
i can solve a 5×5 but i still dont get it
Me too
Im 12 and i get it.
I’m 9 and I understand
Im also nine
im 9 and made my own robot.but this is jibber jabber
What’s your robots name?
SAMUEL LEMONDORF! HA HA HA!!!
grammer suks
i’m only 8 and i know to how build a better robot more gooder than you
Ur English is appalling but I’m 11 and I understand it. It’s easy
im 11 and i made a robot(2 of them(identical)), and i SOMEWHAT get it. SOMEWHAT.
I am 13 and this makes sense. A better (and simpler) way to solve a Rubik’s Cube in 5 minutes or less (from a solved state) is to repeat the same move over and over. I turn the top row right, then the right column down, top row right, right column down. NO TURNING INVOLVED and I COULD DO IT AT AGE OF 7. Make sure that you know somebody who can solve a Rubik’s Cube, just in case you mess it up. After a few tries, you’ll have the rhythm down-pat and can show your friends how they too can solve a Rubik’s Cube.
I’m three months old and am hearing this all completely coherently.
WTF ARE YOU REALLY THREE MONTHS OLD? WHATS YOUR AGE !? DUDE?
Me too
M8 Im 2.99 fite me
lol
Same fam
Fake
there is a way to LOGICALLY solv it in about 2 min or less.
howww?????????????????
Im 15 my hight sccore of rubiks cube solve is 48 second
How?
lol i did it in just under 20 secs tho my average is 35 my method is cfop with advanced f2l and 4lll
I don’t see this as solving a Rubik’s Cube. To be able to solve a Rubik’s cube means being able to take any scramble and figure out how to solve it. This is essentially the same as turning 1 side and then turning it back. You could say you technically solved a Rubik’s Cube, but really you can’t.
This website helped me a lot thank you
Your comment makes no sense. If it made sense, that would mean that it actually made sense, but it doesn’t. This is essentially the same as typing nonsense into the comment section. You could say you technically left a comment, but really you didn’t.
SOOOOOOO HAAAAAARD
YOU NEED A GOOD IQ TO SOLVE A RUBIK CUBE
it is to easy to solve
Well its called “speed cubing” if you learn all the algorithms then you can slove it in like a minute or so.
I’m 11 and “Laura’s” method works better than the weird turning method
Yes but you are a girl and not all boys have the same mental capacity of you even thought some do the usually can’t comprehend until they are atleast 13
wtf
Yeah but I’m 11 and I don’t get it. Well, I sort of get the first half anyway.lol
Doing it the way your describing doesnt change all sides. Thats why its easier.
Wow smart one, if some people like me are actually smart than they know that you can turn top layer, right, “twice”, and right layer, down, “twice.”
I AM IONE HUR OLD UND UNDUERTSAND DIZ PURFEKTY
Figure it out by your self. It’s more self confidence. What I don’t have.
Oh
ohhhhhhh.1st time in my life i got a rubik,s cube to solve but didnt understand much plz some one help me
Your Comment here… oh!its too headache and boring
I can easily agree to that
Makes no sense
Lol
Hello. I understand that you can comprehend this but do us all a favor and use correct Rubik’s language. Instead of the top column right, use U because that is what is should be. For example, Ri, Di,, R, D which would be used for solving corners in the beginners method
I really recommend just learning normally though, I’m 11 and its not hard at all!!
Because this way you’ll be able to solve it from a Scrambled state
If u think about it turning the top one right then turning the right one down will never solve a scrambled Rubix cube because the bottom left four blocks will never move so if them bottom four are different they will never solve themselves because they are never moved
What?! How can u understand all this? #confusedmuch
Fake!!!!
No nobody understands
im 11 and I get it
Just keep trying
No
How did you do that
Yes me too
What the crap this did not help and I’m 19
im 11 and looking for an easy to do method
anyone know one
Many people call it a beginners method. It just takes a long time to memorize, but im younger than you and i did it!
I am 10 and it is stupid
laura’s strategy works so well thanks
Didnt understand any thing
Lol. Its stupid
i think the best method would be to do a beginers method or lbl(layer by layer) this method involves a few algs but its realy not that hard and when you enjoy it even more you could move on to 2 look oll (oriantaion last layer) and 2 look pll (permutation last layer) im only 12 I use 2 look oll and 2 look pll so it wont take you to long to gat the hang of it plus I average 28 seconds with 2 look but if you are doing a 2×2 then you could do lbl (layer by layer) or Ortega or even beginners method I hope this helped and that it wasn’t to hard to understand
Hope You Like This Tricks …
http://www.general-knowledge-quiz.com/rubikscube/instructions.html
There’s not easy way to solve a Rubik’s cube, you have to memorize the cases and the turn sequences and put them together to solve it
wow i had solved it easily
I’m 14 with best solve 40sec you need practise
I feel the same way I’m stuck proper bad
i finished it when i was seven
I need more help than I expected
Me too
same
Agreed
same here
I’m 12 and I solve cubes daily
Easy
Im nine too and its hard and i didn’t understand anything
I’m only 11 goin to be 12
I’m 12 and I’m also confused
This is not a tutorial on “How to solve a Rubik’s Cube”… This is either a joke, or the author needs a few more classes in group theory. Here’s a start: http://www.math.harvard.edu/~jjchen/docs/Group%20Theory%20and%20the%20Rubik's%20Cube.pdf
The author holds a PhD in finite group theory.
age is just a number
Me too
Same here
I know I’m only ten and I didn’t see any steps in there…
If you move the right side clockwise and then the back counter-clockwise, after 102 moves the cube will resolve itself given that you start when the cube is solved. The alhorithm looks like this R B’. And is said “right, back prime”. Hope this helped
im 14 and i understood it
Yeah, I didn’t really understand much either. It obviously isn’t for kids our age. :-(
me tooooooooo
I understand it and im 11 too
I ALSO DONT ‘COZ I AM 12 NOW
it takes to long to put all the numbers on and we can’t see all the numbers
I am 13, i can’t solve a cube but i understand everything.
I neither
can you solve it and it is so hard and I am 10
exactly
I don’t get it only 9
noobs, I am 12 and I hold the record. use cfop
Yea I’m only 11 too
im 11 and this is soooo hard i dont understand
Sammmmmeeeee
Yeah, I don’t understand this!!!
I’m 15 and i can’t even solve a rubik’s cube in general
REALLY IM 10
Hey you are already 19.
Do you understand now?
yep me too
I am 11, and this hard to understand, and did not help my cubing in any way.
This was simply easy to understand, though it didn’t really help at all.
its 2021
Same here
haha its so ez i can do it! im a cubear
minor hahaha
If we spin it in the same position like shown in the video will it come to its original state
this did not help one bit
how long did it take you?
@ fatima: yes it will.
In fact, the process of repeating the same basic sequence is a way to construct moves that only touch a small group of cubies. (I believe this is the term for the little cubelets that make up the Rubik’s cube.) For example, doing $F^2 R^2$ three times is a famous way to exchange two pairs of 2-colored cubies.
“315 is the best you can do with a sequence of up to four of the basic moves”
A more difficult question is: what is the absolute top bound?
I wonder if an answer can be achieved using group theory. I recall there was a lot of this stuff done in the 1980s.
Also, “solve” usually means bring the cube to order from a given (messed-up) state.
I also like using brackets (if you noticed).
Okay first of all stop talking geek these are only kids trying to solve their Rubik’s cubes I’m pretty sure I’m the only other person who understands what your talking about like I said they’re only KIDs not geeks like you and me.
Be nice, some kids do understand what “geek” says, some might not. It’s life, deal with it.
Can u plz reply how to solve rubik’s cube?(simple way?)
Since you’re dissecting the mathematics of Rubik’s cube, I have another question. At how many faces do I have to look at to guarantee that the cube is solved without looking at the rest?
What I mean is, having 1 of the 6 faces solved does not mean that the other 5 are solved as well; they may still be shuffled. The same way, having 2 of the faces solved does not mean that the other 4 are solved as well.
Just looking at 5 faces and seeing them solved will, however guarantee that the 6th is also solved. Is the same true for 4 faces or 3?
If you can only see four faces there are ways the other two faces might be incorrect. If the two faces are adjacent, the edges can be wrong. If they are opposite, the centres could be wrong.
While this might appear true at a first look, it isn’t.
A minimum of four centers can be in disorder at a time. The centers are physically linked in an immutable way.
Also, a single edge piece- can not by itself be in disorder. If flipped, there must be another flipped as well. If switched, there must be at least two others also switched.
However, you are right to say that four sides is not sufficient to determine success:
Looking at the cube in the photo above (the one in which numbered labels were attached). Imagine that Green and Orange are the unseen sides, and all the rest are solved. Faces 11 and 51 could be switched without your knowledge, as could faces 17 and 49. The same principle holds true if the unseen sides are opposite.
This is a fascinating application of math to every day objects. Most people don’t notice that everything is an equation.
oh well it dont work
You are right
Those are not brackets, they are parentheses ( ). These are brackets = [ ]
In British English, () are ‘brackets’ and [] are ‘square brackets’.
What do you call parenthetical statements—bracketory?
() are also called parentheses, but they’re more often called brackets. So we use “parenthetical”. If you’re looking for consistency, English is not the right place to look.
You’d also call a statement parenthetical if, like this one, it’s enclosed by commas or dashes. The punctuation marks which we may or may not call ‘parentheses’ are named after the sort of clause they contain rather than the other way round.
While English doesn’t have a simple set of rules, there are very few exceptions to the vast set of complex regulations.
I don’t have this on any authority, but in my experience and to the best of my knowledge, each “inconsistency” is actually just subject to a very specific rule that doesn’t have many application.
For example:
“I before E, except after C”
Recently, people have added:
“Or when making ‘eɪ’, as in neighbor or beige.”
(Note: ‘eɪ’ is the phonetic description for English’s ‘long A’ sound).
Other exceptions include ‘society’ and ‘science’, both of which can be pointed out as a rule in which the clever little rhyme above does not apply because the I and the E are con part of the same syllable.
Yes, English is weird, complicated, and unintuitive, but I do believe it is mostly consistent.
PS: In case you think you’ve caught me with one of the words in the last remark, know that it actually can be traced back to an Old English version that had two syllables, fitting into one of the rules above and one other major rule: everything in English is inevitably abbreviated, slurred, or otherwise shortened over time.
Hi guys
How to solve a mixxed, small, rubiks cube?
I can’t even solve the cube
Ya, I am with the 11 boy on this. aka, I got nothing.
I’m 12 and all that was just ridiculously shit
Using large vocabulary makes it sound made up just to impress the young
u are rouing
An interesting discussion.
However, your two basic assumptions make the problem much simpler.
1. The unsolved cube was first obtained by a finite sequence of your “transformations” from the pristine “solved” state of the cube.
2. The transformation is reversible.
Hence, you can solve the cube by a finite sequence of the inverse transformation.
Well, yes. The first assumption is the point of the article, and the second one is true for all moves on a Rubik’s cube.
Do you mean that if we repeat the same steps again and again, starting from a solved cube, we will get a solved cube again?
Yes, that is what this means. I wouldn’t really call this “solving” the cube. Given any unsolved cube with no knowledge of how it was obtained, this would not help to solve it. It solves a very special case whose solution is obvious anyway. It is never the less interesting.
Thank you for the article! Ever since I was the kid in 80s I wondered if this kind of quarter turning can get the cube back to the solved state. I think the highest I got before giving up was about 200 turns. Now I know the answer: 1260.
i am also with the 11 year old !!!
i got nothing ! even though i am 15!!!!
nooo it goes back keep on dooing it it does a lot of loops then goes back its called a trigger
Does anyone know an easy way that truly works properly in 2 moves and 1-5mins for an 11year old
no such moves
its all fake
Dunno.Soz
Fuck its hard
Pretty nice comments overall :)
You draw all ages it seems.
i want a way to solve it simply without the use of memorized algorithams
im just 8 years old
No this is not right, if you spin and twist the cube, the top face will eventually come up again, after I think 72 moves, the whole cube will be done again after 6 of these, 72 * 6 does not equate to 3 billion, therefore only a fraction of the possible combinations are possible with this one move. Try it, it takes about 15 minutes.
i am 14. my record is 15 seconds
You can’t do it within 15second by using that equation
I am 13 and my record is 12 seconds
If you want to know how to solve a Rubiks Cube, just go to this website, http://www.howtocube.com/, and bhy the way, here is some moves that mess up from solved and then solve it again: R,L,R,L,R,L,R,L,R,L,R…
I can solve the cube without following any particular algorithm. I just search for the color that is needed to be matched and rotate that column remembering the colors that are going to be disturbed. Doing the same I manage to solve the cube each and every time.
i am getting mad this rubix cube
Good but bad
Wow! This is truly fascinating. What a mind-blowing concept, and yet it makes so much sense. :)
I AM 10 YEAR ‘s OLD.
I AM STUDYING SANATANA DHARAMA VIDYASALA ENGLISH MEDIUM SCHOOL.
AT ALAPPUZHA .
MY HOME WAS KAIJOONDI JUNCTION.
I BUY RUBIX WHILE I HAVE 8 YEAR’s OLD.
BUT I CVAN DO IT !!!!!!!!!!!!!!!!!!!!!
I DO ONE COLOUR AT ONE TIME.
THIS IS NOTHING . I DIDNOT UNDERSTAND THIS.
ANY LIKE THIS ?
PLESE REPLAY
WITH YOUR ADRESS……………………………………
i will tell you. NARNIA BUSINESS!
NO IDEA
man dont be silly not much people say lol
I’m 22 and idk what the fuck I’m doing here
im 6 years old and i can solve the rubiks cube in 1 second litraly i just do 1 thing and it solvves the intire rubiks cube
I’m 13, i don’t understand iT ALL
I like it
wwwwwwwwwwwwhhhhhhhhhhhaaaaaaaaaaaaaaaaaaaa?
Does it really work? And I’m a nine year old.
I CANT DO IT
GOT it
this is easy and im only 11 years old
i think that this was easy
this is easy
i didn’t get it i am 11 years old
GOT STUCK!!!!
I wasn’nt able to get the white corners and match em’ with the centre………..
…………..DIDNT WORK AT ALL.
USE LESS ALGORITHMS
Oh boy
Are you mad
my friend can do it in 30 freaking seconds… at 10
I totally agree this cube is hard D:
I’m so confused.
I feel like throwing the cube away.
This is stupid. I can do a rubiks cube in 45 seconds using algorithms from any scrambled state whereas this can only be used on a solved cube and isn’t really solving it. For that matter, turn one side 4 times and you also have a “solved” cube.
Yes bro
hey I am going to try it and see if it works.
im 9 i dont undesrstand
duuuuuuuuuuuuuuuuuhhhhhhhhhhhhhhhhhhhhhhhrrrrrrrrrrrrrrrrrrrrrrrrrrrrr
This is the worst algorithm ever. Even I could think of that and I’m just a kid.
What the heck I am 11 this was all blah blah to me give me a break
Dafaq?
This is so hard
More like 20k steps.
Just cut to the chase man
This is so stupid I don’t under
stand anything
Me too. All i understand is that the dumb stupid video ruined my rubix cube. I had one side done but then i thought the video showed you how to complete it so now i have to figure out how to complete one side again thanks to that stupid video!
It’s so easy guys, just do 3:4″ 4:3″ 3:4″ 4:5″ 4:5″ 5:4″ 6:8″.
its not easy, but someone on my bus can do the rubix cube in 8 seconds and hes in 3rd grade. Im in 5th grade and i cant even do it. And im awesome at solving puzzles. One time i solved a puzzle in 6 hours in one day and its a 500 piece puzzle. If i can do that, im sure that i could solve the rubix cube but i cant
OMG!The king of cubing on such an amateur website!
Well I live in India and average around ~70 secs.But you do it in just ~6 secs!Even though I use Cfop with algorithmic F2L and 4LLL,the move goes very high,around 110moves.Please help and suggest.
Which means F2L, FL2, FL2, F2L. If you do that algorithm 5-6 times, than your cube will be solved, ( any cube ). Try it guys, you can start from any position, pick up your cube THE WHITE TOWARDS YOU AT ALL TIMES, and start from scratch, I hope you guys do well in solving it.
I dont get any of this number stuff im only 10 1/2
the only thing that i can complete is one side only i all ways do the white side first but then when im done with the white side i cant do anything else. But someone on my bus when i go to school in the morning, someone in 3rd grade can complete the rubix cube in 8 seconds
Who will make me understand ill give him free rechare of 1000
This is sooooo confusing! (-_-) (-_-)
\( (> <) )/
/ \ / \
all the single ladies, all the single ladies…lol
i am from planet Nibiru and wondering why now only did i read all your comments
If you need practice buy a 2×2 rubiks cube.(for beginners)?
i m just born..15 min ago..and trying to play with the cube..this srap is not helping me..
im 12 and this is soooo confusing
Right
Up
Bottom Inversed
and the last move is
Cube (clockwise)
hey man.. .it’s too hard to understand…..make it simple…
Same
what he said
That’s very easy method to solve a rubik’s cube
I’m 12 and I understood a little bit
I don’t get this and I’m 10 1/2 years old and a girl so that doesn’t mean that girls are always smarter than boys and all these comments I’ve read make sense but then I don’t get the other comments Don make any sense so yeah I have a rubik’s cube so if u think breaking the rubik’s cube is cheating I don’t because I was so fist rated when I couldn’t do it
I just learned how to solve it regularly, it’s fun and I can solve it in 25 seconds on average.(personal best is 18.563)
I’m 14 and I’ve done it in 23.54 sec. :-)
The Rubik’s Cube is an amazing puzzle. It just takes practice and practice and practice. You just need to get used to the mindset. I’m 14 but anybody can learn. Just play with it, trying different patterns from its solved state. If you mess it up, you can always just take it apart and put it back together lol
Im nine and this is harder then anything iv ever done
(You guys make no sense) oh how old is the guy who said he was a month old???????
Sorry i mean 3 moths old.
Im negitive 8 and im slightly befudled with algorithm you used
I solved it for 5 secs only
Actually you can do it in 1:30 with layer by layer.
I don’t get it…
I can solve a ruby’s cube regularly, but I’m trying to find a way to solve it in under 40 seconds. This makes no sense what so ever. Not even what I asked for
I’m 17 (apparently that matters). I have over 15 cubes (only like 3 of which are 3x3x3s). My record on a 3×3 is 45 seconds which is not terrible fast but not worthy. When I’m bored I sit around messing with my cube figuring out patters and logic. This entire post made complete sense, the writer clearly is very smart bit does not actually know how to solve a cube. In his video he is doing hi “algorithm” the hard way, but it still works. I really like how he mathematically calculated it but he must have missed something in his calculations . He is exactly 60 short, which is interestong because after sixty of these moves the top of the cube solves and it takes exactly 22 of these sets of 60 to solve it. I have counted it many times. Somehow he came up with 21 which makes no sense because cubes resolve after doing a repetitious algorithm in round even numbers that can in some way be divisible or multiples the amount sides, species and or the sides being moved.
I would like to correct my incorrect counting from my previous comment, since then I have redone this and realized it is actually 21 sets of 60 which is 1260.
THIS SUCKS ITS HORRIBLE
thanks it was really easy with this step my record is 9 seconds.
very good thanks!
Liam
May 23rd, 2016
age is just a number
false! Age is the amount of times you’ve been around the sun. hahahahahahahahaha!!!!!!!!!!!
I know how to solve the cube and i’m just 14.
I solved it in 1m 14 sec.
So easy!Better learn the standard method to solve it.I’m just aged 13 now but,after 4 months of practice,I can solve it under 80seconds
damn it help me solve a rubik’s cube pls lol
me too
I don’t know what I’m supposed to do
Yeah I’m 11 and I don’t understand a thing, well bits of the first half anyway. :-(
I agree it is quite boring.
I dont really get this. ..just one thing that i kinda get and will ask is; this steps only work wgit a rubiks cube that is already solved right? Or will it also work with one that is scrambled??
If you start with a scrambled cube, it will go through its own cycle of 1260 positions and return to the scramble you started with. That cycle of 1260 positions won’t have any positions from the “main” cycle in it (except if by a massive coincidence you started with one of the scrambled positions in the main cycle, in which case you’ll go round the main cycle but starting in a different position).
For some reason I understood it and I’m 10.
I didn’t read it but I know what you are saying.
I am 12 and i know how to solve a rubies cube in 28 seconds! This is true but you have to do it from the when you have the rubies cube already finished and done so this can’t really help any of you that don’t know how to solve one! sry!
I am 11 years old and i can’t understand any thing
I can’t understand any thing
i do no
Thanxxxxx
please help i suck im 13 and still cant solve mine and ive been working on it since i was four
I know how to solve a cube without any move
This did not help one bit.
hoooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooooow!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!DO YOU SOLVE THE CUBE!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!
Ur real savage
Ikr
omg im not even born and i understand this, pfft, troglodytes
Well I’m 53 and… I don’t remember why even came here. ;)
i did not understand anything what rubbish
i’m 5 year old and i understand everything
But the method deviates little bit form my primary solution to this equation
Im 5 and I understand everything.
Well I would like to say the method deviates little bite form an easier path to the primal solution to this equation.
Thank you.
Im gay.
Hahaha
I DONT UNDERSTAND THIS BULL SH*T
I don’t understand thus bullshit of a method. I tried it and it doesn’t work. I woukd not recommend this stupid shitty ass method on how to solve the method. NO ONE TRIED THIS METHOD AT ALL THE PERSON WHO MADE THIS METHOD I A FRAUD AND A LIER DON’T LISTEN TO THEM
Does anyone know how 2 do it????
> needing one slightly laborious calculation to get the sequence for the combined twist-spin
But then again, maybe it’s just on-par with the initial work done to write out the cycles for the spin!
If you combine the two moves you listed:
[twist]
(1 37 27 46)
(2 38 26 47)
(3 39 25 48)
(34 36 30 28)
(35 33 29 31)
followed by:
[spin]
(1 3 9 7)
(2 6 8 4)
(25 27 21 19)
(20 22 26 24)
(12 54 34 37)
(11 51 35 40)
(10 48 36 43)
(13 47 33 44)
(14 50 32 41)
(15 53 31 38)
(16 46 30 45)
(17 49 29 24)
(18 52 28 39)
you get
[twist+spin]
(1 12 54 34 43 10 48 9 7)
(2 15 53 31 40 11 51 35 44 13 47 6 8 4)
(3 18 52 28 37 21 19 25 36 45 16 46)
(14 50 32 41)
(17 49 29 38)
(20 22 26 33 24)
(27 30 39)
If you count, these independent cycles have lengths:
9, 14, 12, 4, 4, 5, and 3.
In prime factors, that is:
3*3, 2*7, 2*2*3, 2*2, 2*2, 5, and 3
The cycle will repeat when all the independent cycles meet up, which is the least common multiple of all the independent cycle lengths. Which is the product of maximum power of each prime across all the cycles:
2*2 * 3*3 * 5 * 7
Which is your 1260.
i am 41 and i want to be a tree!
I don’t understand it one bit I’m 11 years old and it’s like stupid cause they just show you what it looks like but they don’t really help you and it’s annoying and I don’t get it so they suck
I can solve a cube in 30 seconds but this makes no sense
I’m 13 and I understand this. If you read the whole thing it makes sence.
huh
its been a while since ive last been on here. you literaly don’t have to pay attention to this website to solve a Rubiks cube. there is no alorithm to solve any rubiks cube at any time. however, if you have extreme skill, the mind of a robot, you can easily solve any rubiks cube in 24 moves or less. ive been able to solve a 3×3 rubiks cube for years now. the best way to do it is by this link https://www.rubiks.com/media/guides/RBL_solve_guide_CUBE_US_5.375×8.375in_AW_27Feb2020_VISUAL.pdf. its the most basic way to solve a rubiks cube, but it still takes tallent. as time goes on, you can move onto the cfop meathod. im still learning some of the cfop meathod, so i can solve a rubiks cube significantly faster that i used to. my record is 38 seconds, so once i learn all of the cfop i can solve it faster. the world record is 3.47 seconds, so ill never get there. if anyone can find someone who knows how to solve a rubiks cube in 24 moves or less, contact me at dominik.nebelski@gmail.com so that i can hopefully train them to break the record.
Hee
I did not understand anything i am only 10
years old
I’m think there’s a flaw in your impossibility of loops argument. You are assuming that the loop repeats after a complete (integer) set of the original moves. But there’s nothing stopping it from completing a loop after a fractional number of steps, i.e. the last step need not complete the original move but end part way. Then you don’t have a paradox.