 I am now one of the editors of MSOR Connections, a peer-reviewed practitioner journal that welcomes research articles, case studies and opinion pieces relating to innovative learning, teaching, assessment and support in mathematics, statistics and operational research in higher education.
I am now one of the editors of MSOR Connections, a peer-reviewed practitioner journal that welcomes research articles, case studies and opinion pieces relating to innovative learning, teaching, assessment and support in mathematics, statistics and operational research in higher education.
You're reading: Posts By Peter Rowlett
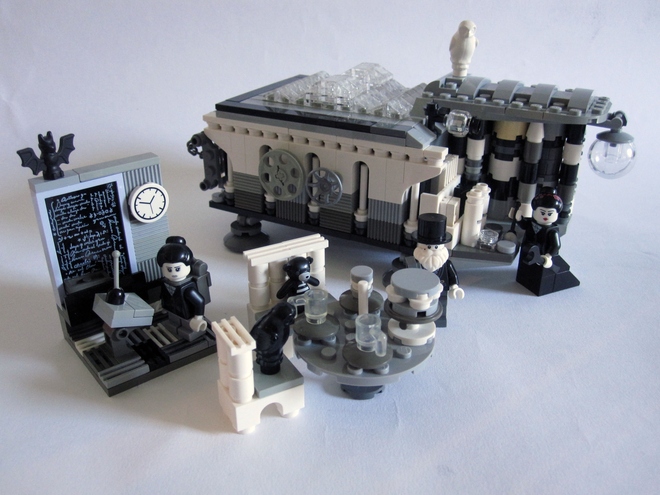
Analytical Engine, Lovelace & Babbage LEGO Ideas proposal needs support
LEGO have a system where people can propose new LEGO sets. If they get 10,000 supporters, they will be reviewed by LEGO. If LEGO like the idea, it may become an actual set they sell (and the person who proposed the idea benefits with 1% of net sales and other rewards).
Anyway, Stewart Lamb Cromar (an e-learning chap at University of Edinburgh) has proposed a set based on the Analytical Engine and featuring Ada Lovelace and Charles Babbage minifigures (including, apparently, spanners). An idea has to get 1,000 supporters in its first year or it will expire; this one has passed that bar in less than two months and has over 2,600 supporters at time of writing.
Anyway, I think it looks quite cool. To support it is free, though you have to sign up for a LEGO ID and answer a short survey: ‘What would you expect this product to cost (USD)?’, ‘How many do you think most people would buy?’, ‘Who do you think this project would be good for?’ and ‘How difficult would you say this project would be to build?’. It only took a couple of minutes (I was supporter no. 2604).
Expect more Ada Lovelace this year as it’s the 200th anniversary of her birth on 10th December. For example, on 17th September at 9pm BBC Four is showing a documentary by Hannah Fry: Calculating Ada: The Countess of Computing.
More information
Maths helps maths graduates get professional jobs
The Destination of Leavers of Higher Education (DLHE, pronounced ‘deli’) survey sends a questionnaire to all UK university graduates six months after graduation and this gives some idea of what happens to students once they graduate. It is flawed, but has a high response rate and is an interesting tool.
There is a second type of DLHE survey, which is longitudinal. This surveys graduates 3.5 years after graduation, and the 2010/11 longitudinal data has just been released. This deserves some investigation and I don’t have time right now, but I did notice a couple of tables that make me proud of my subject.
#realfaceofmath
Kit Yates has asked mathematicians to post a picture of themselves using the hashtag #realfaceofmath, in the hope of dispelling the incorrect stereotype that all mathematicians are geeky white guys with beards and glasses (hi!).
Naked Scientists on the Clay Millennium Prize Problems, f. Katie Steckles
The Naked Scientists Podcast has released an episode on the Clay Millennium Prize Problems, titled ‘The Seven Million Dollar Maths Mystery’. The episode description is:
This week, we’re investigating the Millennium Prize Problems – a set of mathematical equations that, if solved, will not only nab the lucky winner a million, but also revolutionise the world. Plus, the headlines from the world of science and technology, including why screams are so alarming, how fat fish help the human fight against flab, and what’s the future of money?
Better yet, the episode includes a contribution from our very own Katie Steckles talking topology, Poincaré and Perelman.
The episode is available to listen or download as a podcast or, less conveniently, at 5am tomorrow on Radio 5 Live (or later on iPlayer). Not a listener? Read a transcript.
Programming to investigate Quarto
I was invited to contribute to a special issue of The Mathematics Enthusiast on ‘Risk – Mathematical or Otherwise‘, guest edited by Egan J Chernoff. I wrote about the Maths Arcade and programming strategies for a game we play there called Quarto. Really, I was sketching an outline of an idea to encourage student project work.
My title is ‘Developing Strategic and Mathematical Thinking via Game Play: Programming to Investigate a Risky Strategy for Quarto‘ and the abstract is below.
Physics with hidden calculus
Crossing campus this afternoon, a student whose exam is later this week asked me “when you ask a real-world question on the exam and you want us to solve an ODE, can we just do it using formula we memorised from A-level physics?” Like what? “Like with one of the distance questions we might just use $v^2 = u^2 + 2as$.” I said that if they were relying on a result we didn’t use in the module and that they hadn’t proven, this would be a problem.
In the latest Taking Maths Further podcast (Episode 19: Computer games and mechanics), we had a puzzle that we say could be answered roughly, but the precise answer 23.53 (2 d.p.) required a little calculus. On Twitter, @NickJTaylor said
https://twitter.com/NickJTaylor/status/597725017884008450