The Aperiodical 

You're reading: Phil. Trans. Aperiodic.

Log-log! Who’s there? Not a power law!

By Christian Lawson-Perfect. Posted January 26, 2013



This is a story about a particularly awful bit of science reporting. It’s taken me a while to sort out what’s going on. First I thought it was bad maths, then I thought it was bad science, and now I think it might be bad journalism. But it might be none, all, some, or a superset of those. I didn’t really want to write this post because crowing about other people’s apparent failures isn’t great, but we took a team decision to cover it because of entertainment reasons. ((Unlike the BBC, we are not funded in a unique way, so we have no public service remit. I think that’s right.)) I’m going to make an attempt to tell you what I’ve found, a little bit of what I think, and then we can rejoin Team Positive Mental Attitude when it’s all over.

This is an ex-parrot. ‘Is plumage has dimension 1

By Christian Lawson-Perfect. Posted January 25, 2013



A paper by Lorenzo Pérez-Rodríguez, Roger Jovani and François Mougeot in Proceedings B, “Fractal geometry of a complex plumage trait reveals bird’s quality“, claims that the measurement of the fractal dimension of a red-legged partridge’s chest plumage is a good indicator of its health.

I know what you’re thinking: another ‘non-mathematicians pick trendy term to describe something rather different’ story, but actually the authors do quite a good job of explaining and justifying their method. I’m convinced!

Plumage patterns are the product of reaction-diffusion systems which probably don’t really produce fractal dimension, but the researchers needed a fairly easy and consistent way of measuring the complexity of patterns. A healthy bird can produce more melanin, which can produce more complicated patterns. For the level of detail needed, the researchers say that the box-counting method of computing fractal dimension is a quick way of measuring the effect they’re looking for.

Paper: Fractal geometry of a complex plumage trait reveals bird’s quality, by Lorenzo Pérez-Rodríguez, Roger Jovani and François Mougeot, in Proceedings of the Royal Society B.

via Slashdot

The Best Writing on Mathematics 2012

By Christian Lawson-Perfect. Posted November 9, 2012

Every year, Princeton University Press gathers together a small anthology of the best writing on mathematics from the past 12 months.

The Best Writing on Mathematics 2012 was released last week. Now that Princeton’s web servers have been dried out after Hurricane Sandy’s visit, I can give you its blurb:

This annual anthology brings together the year’s finest mathematics writing from around the world. Featuring promising new voices alongside some of the foremost names in the field, The Best Writing on Mathematics 2012 makes available to a wide audience many articles not easily found anywhere else–and you don’t need to be a mathematician to enjoy them. These writings offer surprising insights into the nature, meaning, and practice of mathematics today. They delve into the history, philosophy, teaching, and everyday occurrences of math, and take readers behind the scenes of today’s hottest mathematical debates. Here Robert Lang explains mathematical aspects of origami foldings; Terence Tao discusses the frequency and distribution of the prime numbers; Timothy Gowers and Mario Livio ponder whether mathematics is invented or discovered; Brian Hayes describes what is special about a ball in five dimensions; Mark Colyvan glosses on the mathematics of dating; and much, much more.

The “much, much more” alluded to above includes our very own Peter Rowlett’s collection of essays “The unplanned impact of mathematics”, which was published in Nature last year. And at only £13.95, just £1.95 more than what Nature is asking for Peter’s article alone, The Best Writing on Mathematics 2012 is a steal.

The Best Writing on Mathematics 2012 at Princeton University Press. $19.95/£13.95 in paperback or ebook.

A recreational maths seminar?

By Christian Lawson-Perfect. Posted November 2, 2012

Would you be interested in taking part in a sort of online video-chat seminar about recreational maths? Then read on!

Advances in pure nonsense

By Christian Lawson-Perfect. Posted October 17, 2012

Congratulations to Professor Marcie Rathke of the University of Southern Northern Dakota, whose paper, Independent, Negative, Canonically Turing Arrows of Equations and Problems in Applied Formal PDE has been accepted for publication by the journal Advances in Pure Mathematics.

Here’s a snippet:



Actually, uncongratulations to Prof Rathke, who doesn’t exist, and congratulations instead to Nate Eldredge whose Mathgen program created the paper, along with severe disapprobations to Scientific Research Publishing of “P. O. BOX 54821, Irvine CA”, for apparently not checking that even the title of the paper they received makes sense.

Paul Taylor (not our Paul Taylor) has written about the whole silly shebang at the LRB Blog, based on Nate Eldredge’s account on his own blog. The comments from the “anonymous referee” are hilarious!

 

Proof News

By Christian Lawson-Perfect. Posted October 8, 2012

Here’s a little catch-up with the status of the claimed proofs of some big statements that were announced recently.

At the end of August, Shin Mochizuki released what he claims is a proof of the abc conjecture (link goes to a PDF). Barring someone spotting a huge error, it’s going to take a long time to verify. It’s mainly quiet at the moment, apart from a claimed set of counterexamples to one of Mochizuki’s intermediate theorems posted by Vesselin Dimitrov on MathOverflow, which was quickly shut down because the community there didn’t approve of MO being used to debate the validity of the proof. No doubt there are other niggles being worked out in private as well.

At the start of September, Justin Moore uploaded to the arXiv what he claimed was a proof that Thompson’s group F is amenable. Like Mochizuki’s abc proof, experts thought Moore’s proof was highly credible. We were waiting for my chum Nathan to write about it, since his PhD was all about Thompson’s groups F and V, but it turns out we don’t need to: at the start of this week, Justin retracted his paper because of an error which “appears to be both serious and irreparable”. The amenability of Thompson’s group F has been proven and disproven many times, so I still want Nathan to tell me (and you) all about it.

In lighter news, via Richard Green on Google+, recent uploads to the arXiv show that Goldbach’s conjecture and the Riemann hypothesis are true. I’d love to know how it feels to upload a six-page paper which you know proves something like the Riemann hypothesis. It must be a lovely state of mind. Certainly much better than what people like Moore and Mochizuki must go through, waiting for the first email to arrive telling them they’ve made a terrible mistake and their work is not yet complete.

If I’ve inspired you to have a go yourself, look at Wikipedia’s list of unsolved problems in mathematics and take a crack at one this weekend. Can’t hurt ((Disclaimer: depending on levels of ability, perseverance and agreement with consensus reality, attempts to solve these problems may well ruin your life)) to try!

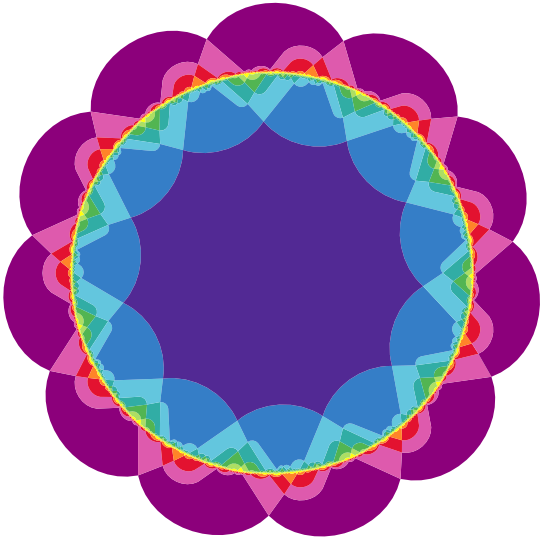
11-category Venn diagram drawn

By Christian Lawson-Perfect. Posted September 2, 2012

Little known fact: some sized Venn Diagrams have never been drawn. In case you missed it when it whipped round Twitter a few weeks ago: it looks like someone finally cracked the 11-Venn diagram, and it’s a cracker!