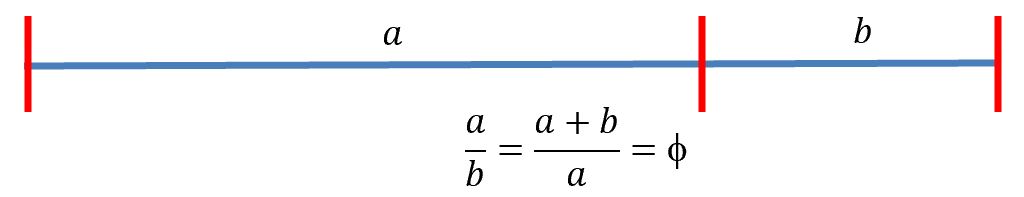Hi! My name is Colin, and I am a PROPER mathematician now. I’ve made a contribution to the Online Encyclopaedia of Integer Sequences.
You're reading: Posts Tagged: fibonacci
- Multiply the first and the last numbers $\rightarrow 3 \times{13}=39$
- Multiply then double the middle two numbers $\rightarrow 5 \times{8}\times{2}=80$
- Sum the squares of the middle two numbers $\rightarrow 5^{2}+8^{2}=89$
- The Pythagorean triple is $(39, 80, 89) \rightarrow 39^{2}+80^{2}=7921=89^{2}$
- The Euclidean distance formula and it’s extension to higher dimensions
- The Pythagoreans and music – Donald Duck in Mathmagic Land
- Biography search of included mathematicians
- 2 High School Students Prove Pythagorean Theorem – proof awaiting verification
- Pythagorean trigonometric identities
- The latest Mersenne prime and a Pythagorean triple
- Related themes – Legendre’s three-square theorem and Fermat’s theorem on sums of two squares
Pythagoras and his theorem
In this guest post, David Benjamin shares a cornucopia of concepts and stories relating to Pythagoras and his famous theorem.
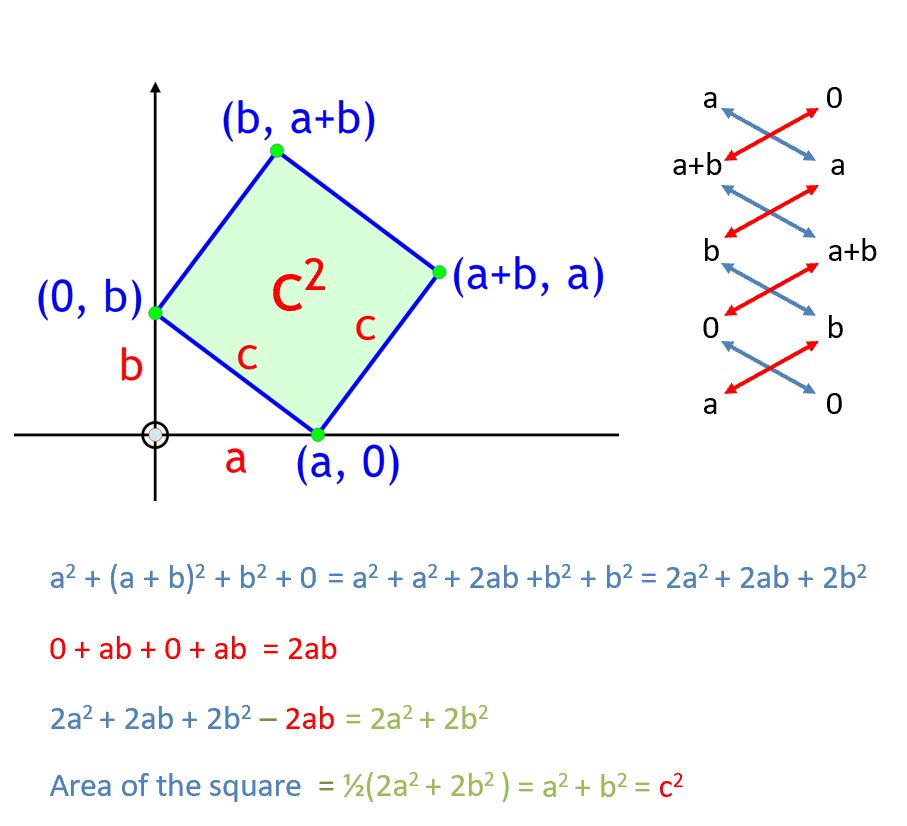
I admit to mild irritation when I’m told that Pythagoras’ theorem is $a^2+b^2=c^2$. The theorem is based on area – in particular, that of squares. There are many proofs of the theorem and in this post we present a miscellany of Pythagorean Theorem curiosities, including some of my favourite proofs, the theorem’s links to algebra, geometry and number theory, an assassination of a president of the USA, an alleged murder in Greece, an infinite spiral of surds, Gauss and coordinate geometry – plus another connection between Pascal and Fibonacci.
The theorem
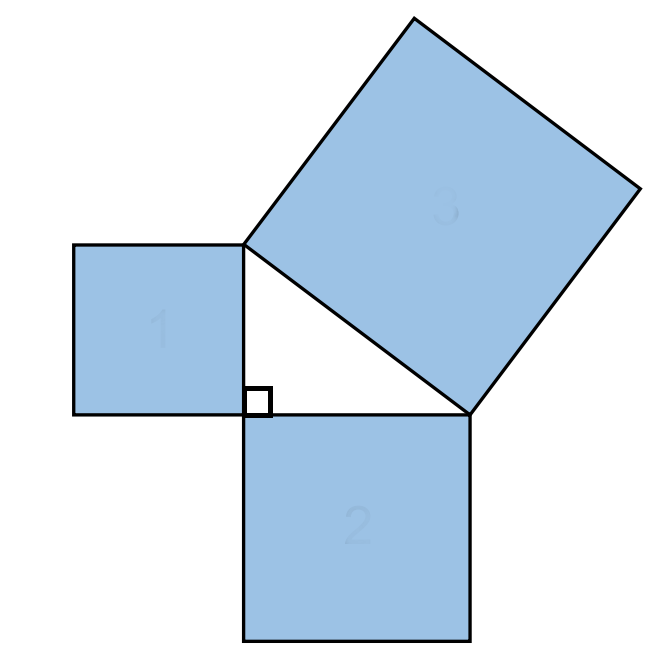
A square is added to each side of a right-angled triangle as shown in the above image. The sum of the the areas of the two smaller squares is equal to the area of the largest square. If the hypotenuse of the triangle has a length of $c$ and the other two sides are of length $a$ and $b$ then $a^2+b^2=c^2$
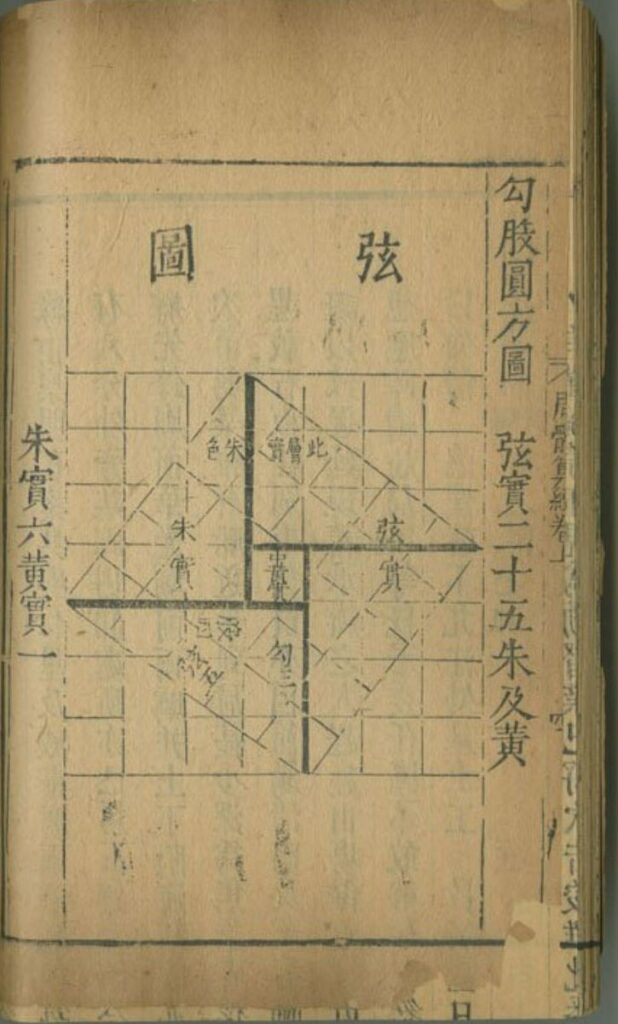
When the length of each side of the triangle is a positive integer, the three numbers make a Pythagorean triple. $(3, 4, 5)$ is the smallest triple with $3^2+4^2=5^2$. The Chinese text Chou Pei Suan Ching – original title Zhoubi – (周髀算经), (The Arithmetical Classic of the Gnomon and the Circular Paths of Heaven) gives this visual proof for the $(3, 4, 5)$ triple.
$(3, 4, 5)$ is a primitive triple since $3, 4$ and $5$ are coprime – their only common divisor is $1$. $(n\times3, n\times4, n\times5), n = 2, 3, 4,…$ are part of the same ‘family’ and clearly not primitive triples. Another primitive triple is $(5, 12, 13)$ and an ordered sequence of hypotenuses for such triples are listed here. In the sequence I was surprised to see $185$ appearing twice. In fact there are exactly four distinct triples with hypotenuse $185$. With the aid of a spreadsheet, I was able to find them: $(57, 176, 185), (60, 175, 185),(104, 153, 185)$ and $(111, 148, 185)$
Generating Pythagorean triples
The sequence $\frac{1}{1},\frac{3}{2},\frac{7}{5},\frac{17}{12},\frac{41}{29},\frac{99}{70},\frac{239}{169},\frac{577}{408},\frac{1393}{985},…$ produces a Pythagorean triple from every other term beginning with $\frac{7}{5}$:
$\frac{7}{5}=\frac{3+4}{5} \rightarrow 3^2 + 4^2 = 5^2$
$\frac{41}{29}=\frac{20+21}{29} \rightarrow 20^2 + 21^2 = 29^2$
$\frac{239}{169}=\frac{119+120}{169} \rightarrow 119^2 + 120^2 = 169^2$
$\frac{1393}{985}=\frac{696+697}{985} \rightarrow 696^2 + 697^2 = 985^2$
…
As an added bonus, the decimal equivalent of each term of the sequence converges to $\sqrt2$, in a similar way the Fibonacci sequence converges to the golden ratio $\psi=\frac{1+\sqrt5}{2}$
Another method to find Pythagorean triples uses consecutive even numbers and the sum of their reciprocals as shown below.
Choose any pair of consecutive even numbers:
$8$ and $10$ $\rightarrow \frac{1}{8} + \frac{1}{10} = \frac{9}{40} \rightarrow 9^2 + 40^2 = 1681 = 41^2$
$12$ and $14$ $\rightarrow \frac{1}{12} + \frac{1}{14} = \frac{13}{84} \rightarrow 13^2 + 84^2 = 7225 = 85^2$
$20$ and $22$ $\rightarrow \frac{1}{20} + \frac{1}{22} = \frac{21}{220} \rightarrow 21^2 + 220^2 = 48841 = 221^2$
Euclid of Alexandria (325BC – 265BC) was a Greek mathematician who wrote a treatise, The Elements – a collection of 13 books. Books 1 to 6 are on plane geometry and books 7 to 9 on number theory. Euclid created a formula for generating Pythagorean triples from any pair of positive integers $m$ and $n$, where $m>n$:
($m^2-n^2, 2mn, m^2+n^2$) is the triple.
If $m=7$ and $n=3$, the triple is ($40, 42, 58$) $\rightarrow 40^2 + 42^2 = 3364 = 58^2$
If $m=89$ and $n=11$, the triple is ($7800, 1958, 8042$) $\rightarrow 7800^2 + 1958^2 = 64673764 = 8042^2$
A lovely link between Pascal, Fibonacci, Euclid and Pythagoras comes via any four consecutive Fibonacci numbers
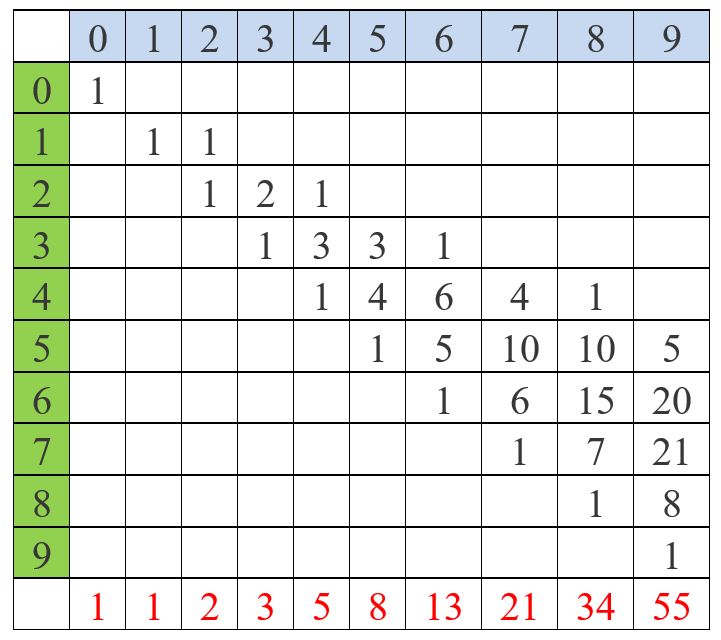
| $F_{1}$ | $F_{2}$ | $F_{3}$ | $F_{4}$ | $F_{5}$ | $F_{6}$ | $F_{7}$ | $F_{8}$ | $F_{9}$ | $F_{10}$ |
| $1$ | $1$ | $2$ | $3$ | $5$ | $8$ | $13$ | $21$ | $34$ | $55$ |
Using $3, 5, 8, 13$
$F_{1}$ to $F_{4}$ gives the primitive triple $(3, 4, 5)$
$F_{2}$ to $F_{5}$ gives the primitive triple $(5, 12, 13)$
$F_{3}$ to $F_{6}$ gives the triple $(16, 30, 34)$
$F_{7}$ to $F_{10}$ gives the primitive triple $(715, 1428, 1597)$
In addition, for any set of four consecutive Fibonacci numbers, $F_{7}$ to $F_{10}$ for example, the following connection is true
$7+10=17$ and the $17^{th}$ Fibonacci number is $1597$, the third member of the triple, the hypotenuse of the triangle!
Amazingly, if we use $m$ and $n$ as consecutive Fibonacci numbers when using Euclid’s method, then the last number of the triple is again a Fibonacci number
| $m$ | $n$ | Triple | Fibonacci number |
| $2$ | $1$ | $(3, 4, 5)$ | $5^{th}$ |
| $3$ | $2$ | $(5, 12, 13)$ | $7^{th}$ |
| $5$ | $3$ | $(16, 30, 34)$ | $9^{th}$ |
| $8$ | $5$ | $(39, 80, 89)$ | $11^{th}$ |
| $13$ | $8$ | $(105, 208, 233)$ | $13^{th}$ |
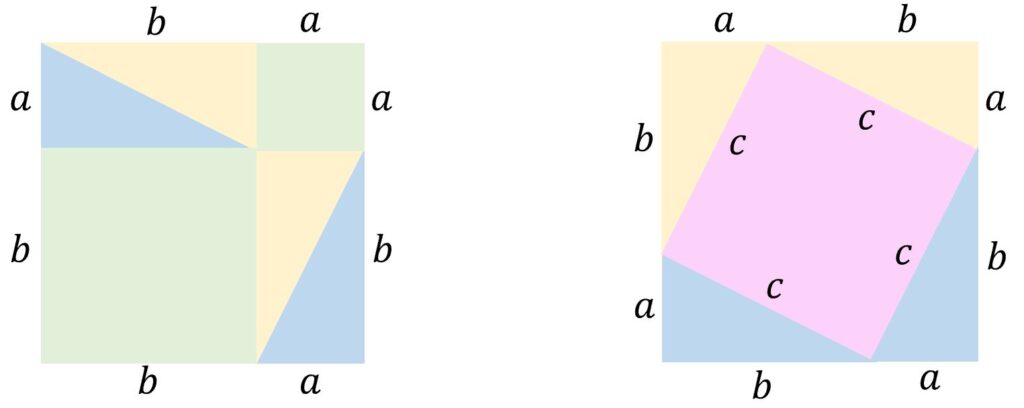
A visual proof of the theorem
I first came across a visual proof of Pythagoras’ theorem for all right-angled triangles in Roger B. Nelsen’s wonderful book Proofs Without Words, Exercises in Visual Thinking. Nelsen noted the proof (author unknown, circa B.C. 200?) is adapted from the Chou Pei Suan Ching. The two images below combine to show the proof:
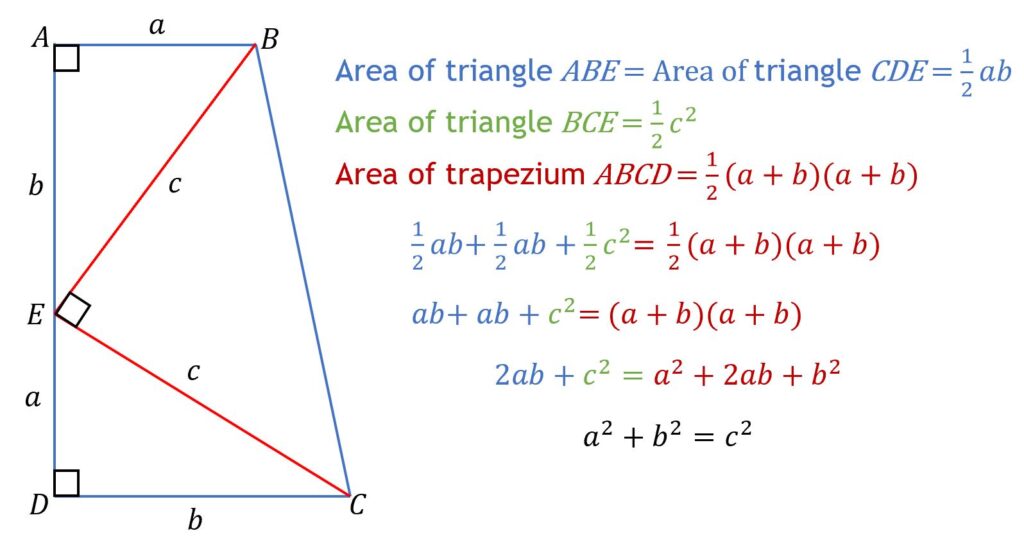
A proof by trapezium area and an untimely painful death
James Garfield (November 19, 1831 – September 19, 1881) was elected as the United States’ 20th President in 1880. He was assassinated after just 200 days in office after being shot on July 2, 1881, in a Washington railroad station. Garfield remained mortally wounded in the White House for many weeks where Alexander Graham Bell, inventor of the telephone, attempted to locate the bullet with an induction-balance electrical device which he had designed. Bell and physicians were unsuccessful in their attempts and Garfield died from an infection and an internal haemorrhage on September 19, 1881.
In 1876, Garfield had an elegant proof of Pythagoras’ theorem published. The proof makes use of the formulae for the areas of a triangle and a trapezium. The proof is demonstrated in the image below
A proof using the Shoelace formula
The brilliant German mathematician Johann Carl Friedrich Gauss (30 April 1777 – 23 February 1855) developed a formula to calculate the area of a polygon if every vertex of the polygon lies on a known Cartesian coordinate. The formula is widely known as the Shoelace formula and makes use of the calculation to find the determinant of a 2 by 2 matrix.
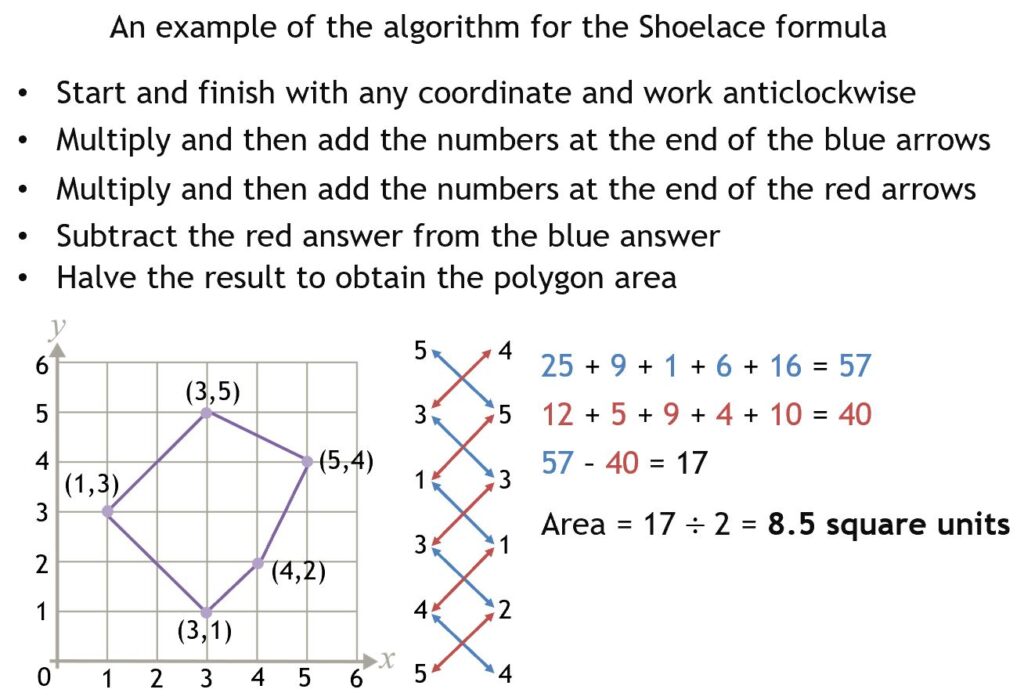
John Molokach observed that the Pythagorean theorem follows from Gauss’ Shoelace Formula, as shown below
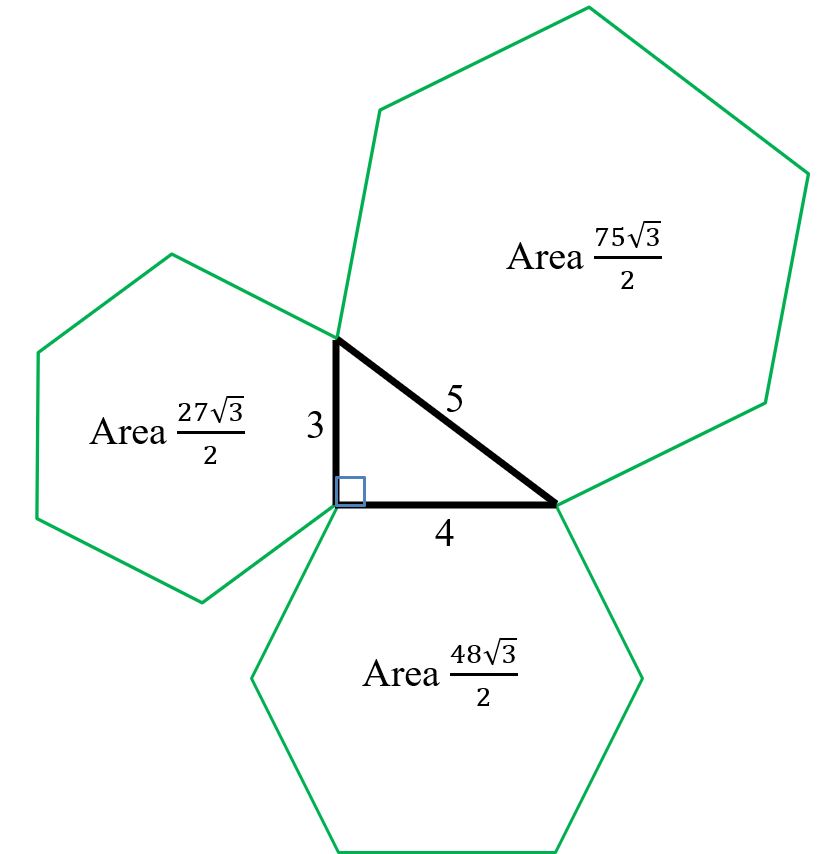
It’s not just squares
If the same regular $n$-gon, $n$ = 3, 4, 5,.. is drawn on each side of a right-angled triangle, then the sum of the areas of the two smaller $n$–gons equals the area of the $n$–gon on the hypotenuse. Semicircles also produce the same result. As $n\rightarrow \infty$, a regular $n$-gon approaches a circle and so circles, where the sides of the triangle act as tangents to the circles can be said to satisfy Pythagoras’ theorem. The same result can be obtained by rotating the semicircles through $180^ \circ$ and adding matching semicircles.
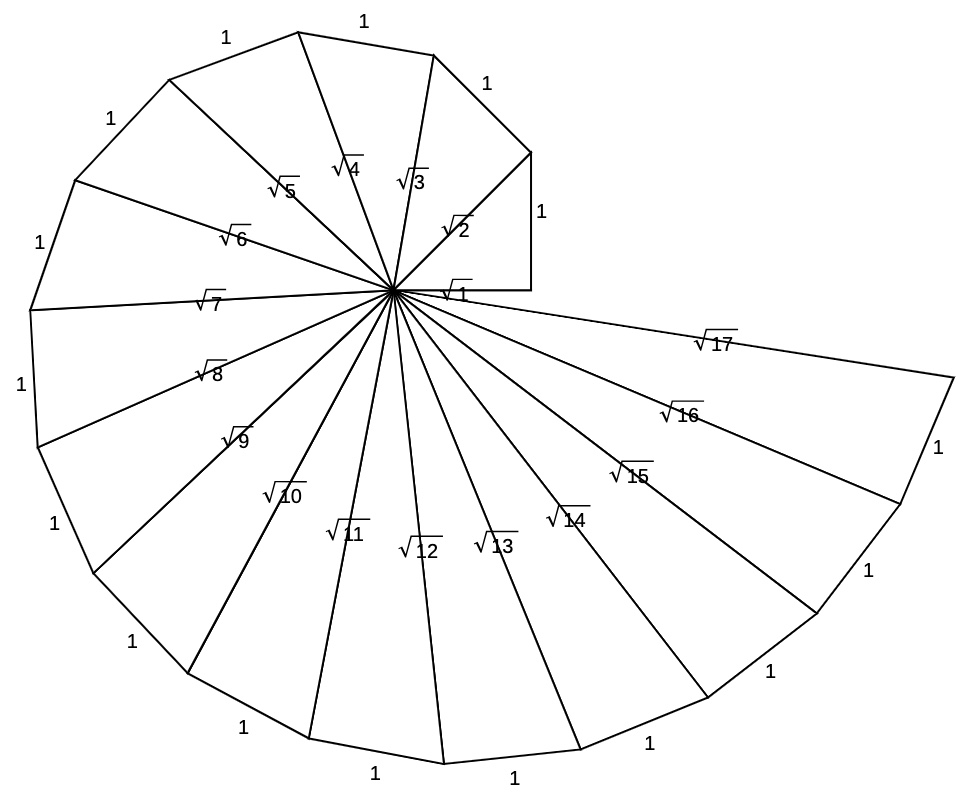
The spiral of Theodorus of Cyrene
When $n$ is not a square number, $\sqrt{n}$ is called a surd
Pythagoras and his followers, the Pythagoreans, believed that the universe can be explained by whole numbers and the ratio of whole numbers. Their moto – “All is number” – was carved above the entrance of their meeting place. However, a spanner was thrown in the works when Hippasus of Metapontum, one of the Pythagoreans suggested that $\sqrt2$, the length of the hypotenuse of the right-angled triangle with sides $1, 1$ and $\sqrt2$, could not be written as the ratio of two whole numbers. Such numbers are now called irrational and it was approximately 200 years before Euclid of Alexandria proved $\sqrt2$ was irrational. The Pythagoreans were sworn to secrecy and one legend suggests that Hippasus was thrown off a boat and drowned for revealing his discovery to non-Pythagoreans. Then again, some believe Hippasus drowned because he revealed how to construct a dodecahedron inside a sphere. The ($1, 1$,$\sqrt2$) triangle is the start of the spiral of Theodorus. Further right-angled triangles, each containing a side of length $1$ unit, are added sequentially to produce the sequence $\sqrt2, \sqrt3, \sqrt4, \sqrt5,…$. The sequence is the length of each new hypotenuse, as shown in the diagram below. Theodorus probably stopped at $\sqrt17$ as it the length of the hypotenuse of the triangle before the triangles begin to overlap. The spiral can be expanded here.
Further reading
Fibonacci, Lucas and the Golden Ratio in Pascal’s Triangle
This is the third in a series of guest posts by David Benjamin, exploring the secrets of Pascal’s Triangle.
Leonardo Pisano (1170-1250), now universally known as Fibonacci, was born in Pisa, Italy, where he was also living at the time of his death. He was educated in north Africa as his father worked there, representing the merchants of the Republic of Pisa when they were trading in Bugia, now called Béjaïa, a Mediterranean port in Algeria.
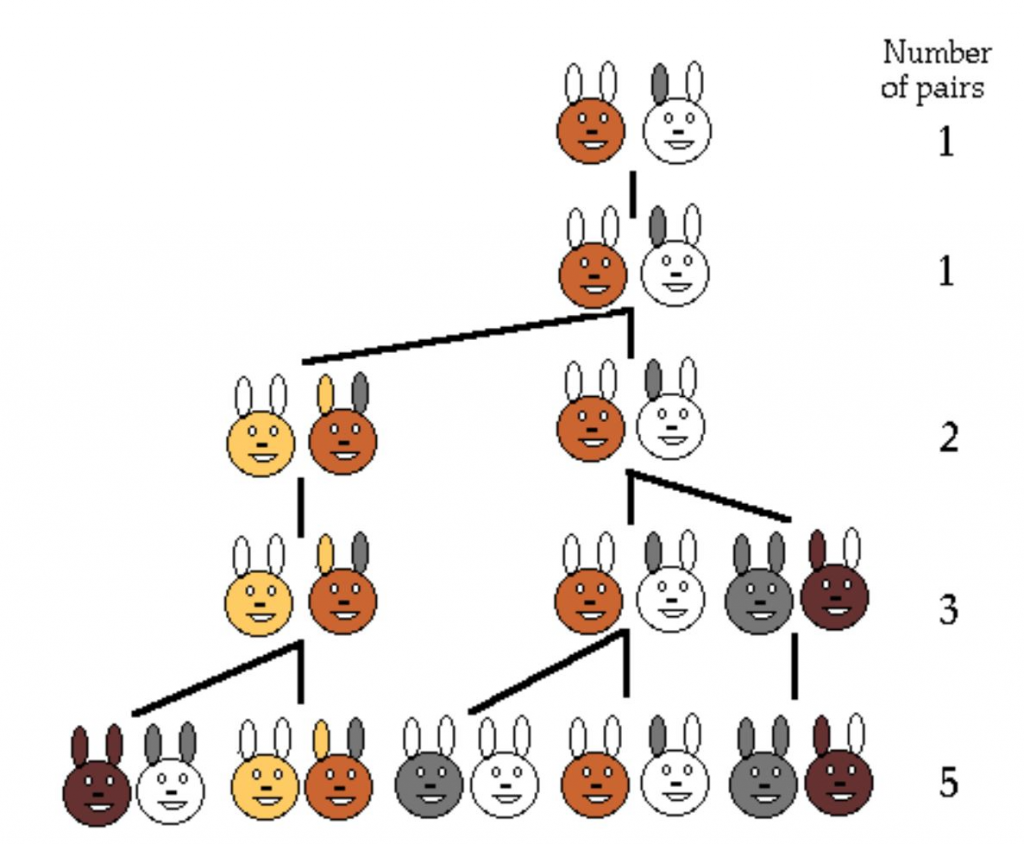
Fibonacci returned to Pisa in about 1200 where he wrote a number of important books. His book Liber abaci introduced the Hindu-Arabic place-valued decimal system and the Arabic numerals we now use. Books and any copies had to be handwritten, as it predated the printing press. Fibonacci is now mostly remembered for introducing the Fibonacci numbers and sequence which appeared in the third section of Liber abaci as a problem about rabbits:
A certain man put a pair of rabbits in a place surrounded on all sides by a wall. How many pairs of rabbits can be produced from that pair in a year if it is supposed that every month each pair begets a new pair which from the second month on becomes productive?
The resulting sequence is $1, 1, 2, 3, 5, 8, 13, 21, 34, 55…$ (although Fibonacci did not include the first term in the book).
The ratio of successive terms converges on the Golden Ratio, $\phi$.
$\phi = \displaystyle\frac{1 + \sqrt5}{2} \approx 1.618033988749. . .$
$\phi$ is an irrational number and is the positive solution of the quadratic equation $x^2 – x – 1 = 0$ Hence, since $\phi$ is the root of an integer polynomial, it is not transcendental, unlike $\pi$.
\[ \frac{1}{1} = 1 \qquad \frac{2}{1} = 2 \qquad \frac{3}{2} = 1.5 \qquad \frac{5}{3} = 1.666 \ldots \qquad \frac{8}{5} = 1.6\]
\[ \frac{13}{8} = 1.625 \qquad \frac{21}{13} \approx 1.615384 \qquad \frac{34}{21} \approx 1.619047 \qquad \frac{55}{34} \approx 1.617647 \qquad \ldots \]
Indeed, convergence to $\phi$ remains true if we start with any pair of Natural numbers and follow the same pattern where any term after the second is the sum of the previous two terms.
| Terms | Ratio |
| 3 | 2.33333… |
| 7 | 1.428571… |
| 10 | 1.7 |
| 17 | 1.58823… |
| 27 | 1.62962… |
| 44 | 1.61363… |
| 71 | 1.61971… |
| 115 | 1.61739… |
| 186 | 1.61827… |
| 301 | 1.61794… |
| 487 | 1.61806… |
| 788 | 1.61802… |
| 1275 | 1.61803… |
| Terms | Ratio |
| 5 | 0.6 |
| 3 | 2.66666… |
| 8 | 1.375 |
| 11 | 1.72727… |
| 19 | 1.57894… |
| 30 | 1.63333… |
| 49 | 1.61224… |
| 79 | 1.62025… |
| 128 | 1.61718… |
| 207 | 1.61835… |
| 335 | 1.61791… |
| 542 | 1.61808… |
| 877 | 1.61801… |
| Terms | Ratio |
| 2 | 0.5 |
| 1 | 3 |
| 3 | 1.33333… |
| 4 | 1.75 |
| 7 | 1.57142… |
| 11 | 1.36363… |
| 18 | 1.61111… |
| 29 | 1.62068… |
| 47 | 1.61702… |
| 76 | 1.61842… |
| 123 | 1.61788… |
| 199 | 1.61809… |
| 322 | 1.61801… |
In Liber abaci, Fibonacci included other numeracy problems – on perfect numbers, the Chinese remainder theorem and on the sum of arithmetic and geometric series. He wrote a book on geometry, Practica geometriae, and perhaps his most impressive work was Liber quadratorum in which he included methods for finding Pythagorean triples. But it is for his sequence for which he is mainly remembered.
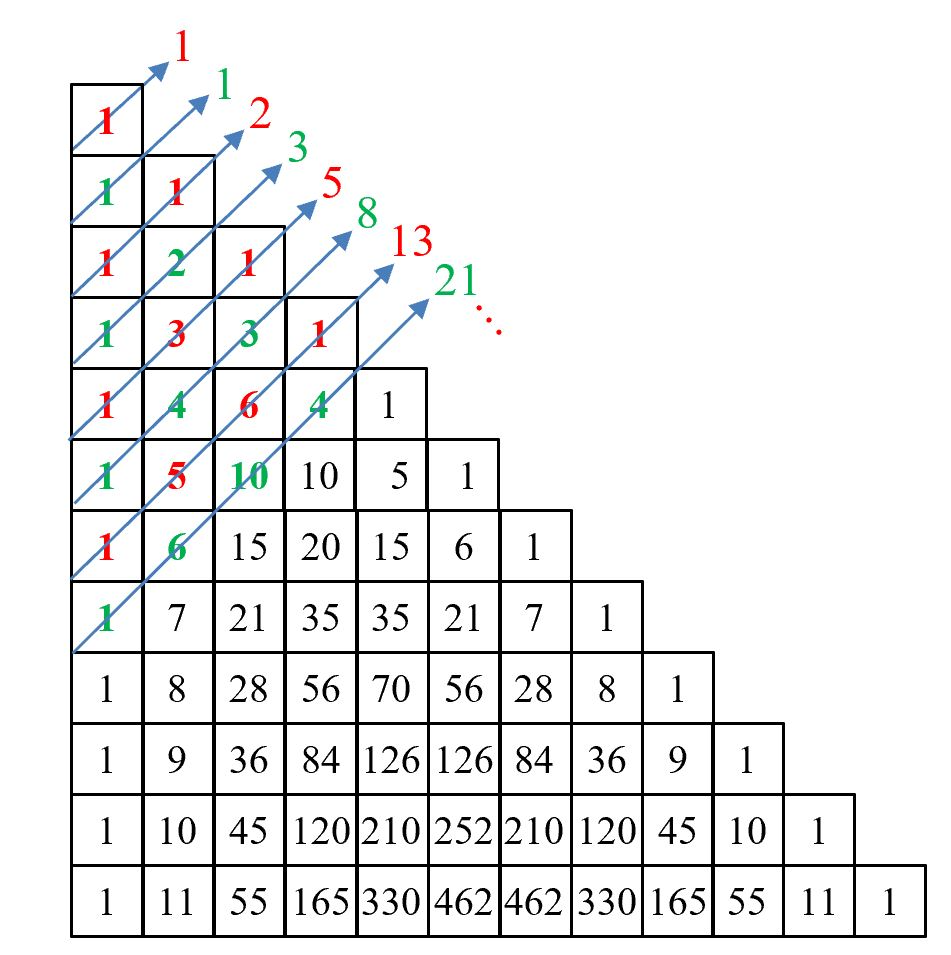
The Fibonacci Sequence in Pascal’s triangle
Finding out that the Fibonacci sequence can be found in Pascal’s triangle was a delight for me and I find it hard to think it is just a coincidence. To view Fibonacci’s sequence we can display the triangle as a right-angled triangle.
The Golden ratio in art, music and architecture
My interest in mathematics began when the film Donald Duck in Mathmagic Land was shown to our class in my first year at secondary school in Burnage, Manchester, England and as a teacher of mathematics I showed it in the lesson before Christmas to many year 7 groups.
The film illustrates how the Golden Rectangle has been used by artists and architects throughout history as well as connections between the golden ratio and music. The film mimics some of the novel Alice in Wonderland by Lewis Carroll, the pseudonym of the mathematician Charles Lutwidge Dodgson.
Further connections between the golden ratio and music can be found here and between the ratio and a Stradivarius violin here:
The Lady Blunt shown above shows the measurements connected to the golden ratio:
\[ \frac{a_1 +a_2}{a_2}=\frac{a_2}{a_1}=\frac{b_2}{b_1}=\frac{b_2}{c_2}=\frac{c_2}{c_1}=\phi \]
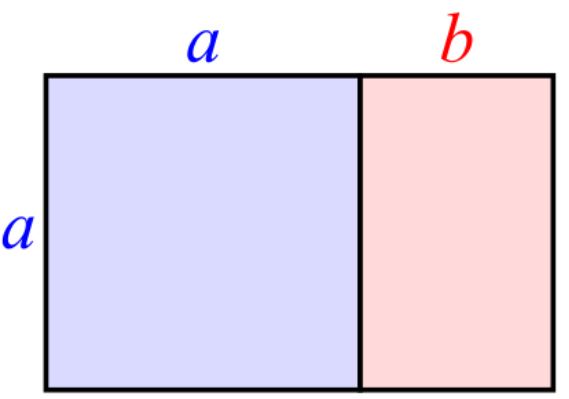
Below is a geometric interpretation of the golden ratio and the golden rectangle:
The Lucas numbers in Pascal’s triangle
The French mathematician François Édouard Anatole Lucas (1842-1891) served as an artillery officer in the Franco-Prussian War, and subsequently became professor of mathematics at the Lycée Saint Louis and then professor of mathematics at the Lycée Charlemagne, both in Paris. Lucas did a lot of work on number theory and was particularly interested in the Fibonacci sequence and devised the test for Mersenne primes which is still used today.
Lucas died of erysipelas (a bacterial skin infection) a few days after a freak accident. He was at a banquet when a fragment of a dropped plate flew up and cut his cheek.
His sequence, the Lucas sequence, begins with the pair of numbers $2$ and $1$ and its terms are generated in the same way as for the Fibonacci sequence.
$2, 1, 3, 4, 7, 11, 18, 29, 47, 76, 123, 199, 322, 521…$
There are a number of connections between the Fibonacci sequence and the Lucas sequence. The $3^{rd}$ Lucas number is the sum of the $1^{st}$ and $3^{rd}$ Fibonacci number, the $4^{th}$ is the sum of the $2^{nd}$ and $4^{th}$, the $5^{th}$ is the sum of the $3^{rd}$ and $5^{th}$, the $6^{th}$ is the sum of the $4^{th}$ and $6^{th}$,…
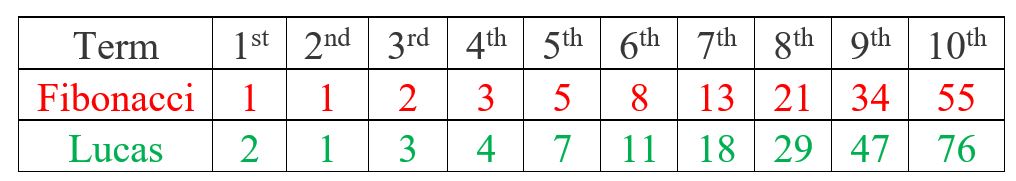
Division of the Fibonacci terms $2n$ and $n$ beginning with the $2^{nd}$ term yields the Lucas terms
$2^{nd} \div 1^{st} = 1 \div 1 = 1$
$4^{th} \div 2^{nd} = 3 \div 1 = 3$
$6^{th} \div 3^{rd} = 8 \div 2 = 4$
$8^{th} \div 4^{th} = 21 \div 3 = 7$
$10^{th} \div 5^{th} = 55 \div 5 = 11$,..
With some manipulation of Pascal’s triangle and some basic arithmetic, we can find the Lucas numbers in the triangle. We begin by setting out the triangle as below and sum the columns to obtain the Fibonacci sequence
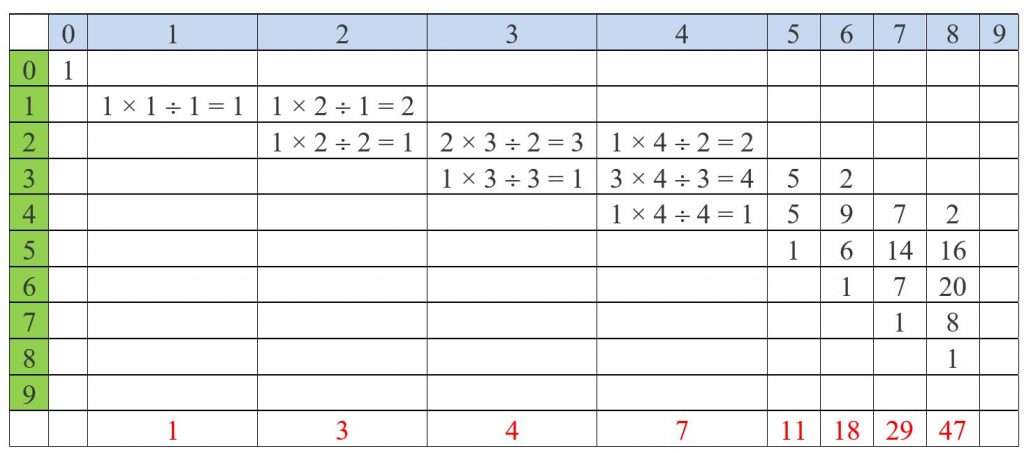
We now multiply each Pascal number by its column number and divide by its row number, starting with row $1$ column $1$ and then sum the new entries in each column. The first few calculations are shown below:
Generally, $\displaystyle\frac{\phi^n -(\frac{1}{\phi})^n}{\phi -(\frac{1}{\phi})}$ is the formula for the $n^{th}$ Fibonacci number, $\displaystyle\frac{\phi^n +(\frac{1}{\phi})^n}{\phi +(\frac{1}{\phi})}$ is the formula for the $n^{th}$ Lucas number and $\phi^n =\displaystyle \frac{L_n+ \sqrt5 \times F_n}{2}$, where $L_n$ and $F_n$ represent the $n^{th}$ Lucas and Fibonacci numbers respectively.
In the next part, we’ll consider some more connections between the triangle and particular numbers, and types of numbers.
Fi-Bee-onacci
Andrew Stacey: I have a confession to make that would probably get me thrown out of every respectable Mathematics Society – were I to belong to one.
I am not a fan of the Fibonacci sequence.
Neither am I keen on the golden ratio. It’s not even transcendental.
It’s not really their fault, it’s just that they get levered in everywhere whether they belong there or not. Particularly in discussions of nature and beauty, and this is exemplified by that ridiculous origin story. We’ve been subjected to a variety of bizarre origin stories over the years (cough radioactive spider cough) but the rabbit story is another level of bizarre.
So I was intrigued, and then delighted, when one of my students, who is a bee enthusiast, told me about a genuinely natural occurrence of the Fibonacci sequence in the ancestry of bees.
I’ll let her take up the story.
Guest post: Sequence Numbers
This is a guest post, sent in by David, who’s discovered an interesting property of numbers, and is looking for collaborators to take it further.
Mathematics is not a careful march down a well-cleared highway, but a journey into a strange wilderness, where the explorers often get lost. Rigour should be a signal to the historian that the maps have been made, and the real explorers have gone elsewhere.
– W. S. Anglin
A few years ago I saw a post on a website that showed that the inverse of 998,001 produces a decimal expansion that counts, using three digit strings, from 000 to 997 without error.
\[ \frac{1}{998,\!001} = 0.0000010020030040050060070080090100110120130\ldots \]
I immediately thought that this had to be a hoax. I decided to work it out to prove it was a hoax – after all some people put anything they want on the web whether it is true or not.
‘All that glitters is not golden’: a Fibonacci Day Roundup
Yesterday was 23/11, also known in some parts as 11/23, and you may recognise this as being a date made of the first four Fibonacci numbers. (Such numerical date-based Fibonacci coincidences haven’t been as exciting since 5/8/13, but at least this is one we can celebrate annually.) This meant that mathematicians everywhere got excited about #FibonacciDay, and spent the day talking about the amazing sequence. Here’s a round-up of some of the best bits, so you can celebrate Fibonacci day in style.
Science Showoff
Science Showoff is a monthly night which takes place in a pub in London, and features acts from all areas of science, who each have 9 minutes to perform an act – a science demo, a routine, songs, experiments – anything entertaining or fun. Having tried a little bit of the short-set, trying-to-be-funny type of science communication involved in Bright Club (a similar venture, giving researchers the chance to try stand-up comedy, which started in London and has now spread all over the country), I thought it would be good to give it another go – in fact, Science Showoff was recommended to me by someone who saw my Bright Club set in Manchester. I had prepared an 8-minute piece about Fibonacci numbers to perform in Manchester, inspired by my artist friend’s admission that she didn’t see how maths could be interesting in the same way as art; she wasn’t there to watch, but I went down well (and ran horribly over time). So I decided to reprise my set at Science Showoff in February 2012 – and this time it would be the right length, and would be new and improved with all the best jokes left in and the duds taken out.