Here’s the fifth match in Round 1 of The Big Internet Math-Off. Today, we’re pitting Tiago Hirth against Evelyn Lamb.

Take a look at both pitches, vote for the bit of maths that made you do the loudest “Aha!”, and if you know any more cool facts about either of the topics presented here, please write a comment below!

Tiago Hirth – Non-abelian Borromean rings

Tiago Hirth is a Mathemagician for the Circo Matemático (Mathematical Circus), part of the Ludus Association, and PhD student at the University of Lisbon.

So I first came across this particular trick around Christmas 2016 while searching for mathematical rope tricks. I stumbled upon a three year old article (at the time) of the Scientific American blog describing a trick first performed by a Curtis McMullen and its mathematical explanation. I’m not sure if he originally came up with the performance, but recently at the G4G meeting we, Jordan Gold (a world famous magician), Colin Wright (he’s one of the competitors so I won’t praise him too much, but he is awesome too) and I, came up with an alternate performance that got rid of the carabiners in favor of a more tactile approach to the illusion. A similar performance had been suggested a while back by Mathematical Circus colleague Francisco Picado. During the same meeting in Atlanta some additional thoughts and illustrations of how the effect works came to mind in conversations and performances for and with multiple people.

Many may already be familiar with similar demonstrations and games of thought, from problems like the Picture Hanging Problem. Quick run down: You have one or more nails around which you wrap a cord. Find a way to twirl the cord around the nails so that removing any one of them, the whole cord slides off (WARNING: in real world situations friction is not to be disregarded). If you haven’t done so, now is the time, potential spoilers to follow.

We can view the string as a path, starting at the paining and ending at the painting. As such it has a starting-point, $A$, and an end-point, $B$, and can therefore be oriented. It happens that the path is looped and $A$ and $B$ coincide, the painting (for simplicity of visualisation). The path leading around the nails ‒ from none, $i = 0$, to as many as we want, for an index of nails, $i$ ‒ is homotopic to a combination of simpler oriented paths, $\alpha_i$, with respect to each nail and which way it wraps around. In this notation $\alpha_1^2$ means that the string is looped twice around the first nail in positive orientation, that is counterclockwise. $\alpha_3^{-5}$ means the the string loops clockwise five times around the third nail. For no nail every path is loose, which is our identity, and we are set to do algebraic operations. For 1 nail it is easily visualized that $\alpha\alpha^{-1}$ falls off, this is, that path is homotopic to the constant loop, no nail. Further any path we choose to make around the nail simplifies to be constant once the only nail is removed.

This simplification holds true for more nails as well. The picture hanging problem thus becomes: Find a non-constant sequence of paths that cancels itself out once a nail is removed. For two nails we get solution is $\alpha_1\alpha_2\alpha_1^{-1}\alpha_2^{-1}$. For three nails $[[\alpha_1, \alpha_2], \alpha_3]$ is a solution. Here, $[\alpha_1, \alpha_2] = \alpha_1\alpha_2\alpha_1^{-1}\alpha_2^{-1}$ is also known as the commutator of $\alpha_1$ and $\alpha_2$ and gives us an indication of the extent to which our paths fail to be commutative (swap things around to get back to the identity). For an arbitrary number of nails, the formula $[[\alpha_1,\alpha_2], \alpha_3] \ldots, \alpha_k]$ holds. If this was too confusing or to incomplete an explanation, you can learn more on YouTube, there are some really nice videos out there.

Now back to the rope trick. The carabiners, or clasped fingers, are nothing more than nails in disguise. Once we intertwine the fingers or carabiners we end up restoring the commutative property to the system and everything falls loose. Another way to look at this, is to resort to Borromean Rings. In the case of the rings no two rings are linked, but each ring holds the other two in place by passing in their “middle”. If we are to link two, the third falls off. And that is precisely what is happening in our trick.

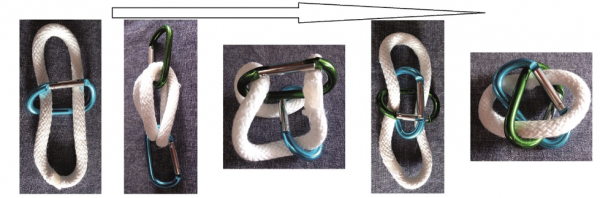
If you have seen this particular trick before, know of similar tricks, have more explanations, or otherwise are interested in mathematical rope magic, topology and the like I’d love to know more.

Evelyn Lamb – The Wallis sieve

Evelyn Lamb is a freelance math and science writer based in Salt Lake City, Utah, USA. She writes the blog Roots of Unity for the Scientific American blog network and co-hosts the podcast My Favorite Theorem with Kevin Knudson. You can find her on Twitter at @evelynjlamb, on Instagram at @evelynjlamb, or subscribe to her newsletter, Stuff Evelyn wants you to read.

This is a neato little space. It looks kind of like the Sierpiński carpet, which is already very cool, but instead of taking a square, dividing it into ninths, removing the middle ninths of the remaining squares, and so on, you remove a smaller proportion each time.

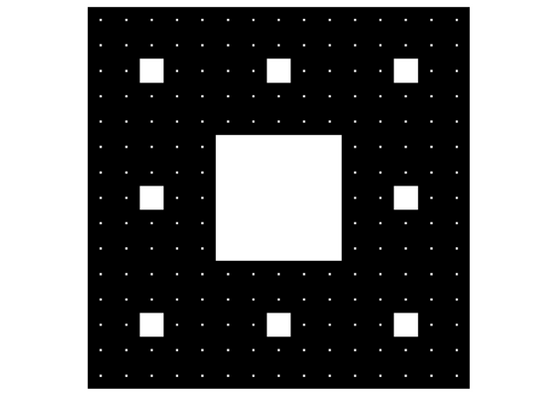
Start with a square, divide it into a 3×3 grid of smaller squares, and remove the middle one. Then divide the remaining eight squares into 5×5 grids of squares and remove the middle one, so 1/25 of the total area, then remove the middle 1/49 of the remaining squares, and so on. It looks very cool, and the area has a neat relationship to π.

I’ve got some pictures and more details about the sieve and π in my blog post about it. I found out about it via this Matt Parker video:

So, which bit of maths has tickled your fancy the most? Vote now!

 Loading ...

The poll closes at 9am BST on the 7th. Whoever wins the most votes will get the chance to tell us about more fun maths in round 2.

Come back tomorrow for our sixth match in round 1, pitting Matt Parker against Matthew Scroggs, or check out the announcement post for your follow-along wall chart!