This month we had a lovely MathsJam, with plenty of old and new faces and a disturbing quantity of activity related to black and white counters. I’d brought a big pile of them and some stuff to do with them, and we got cracking.
First, we looked at the lovely puzzle of which numbers can be written as a sum of consecutive integers – some can, and some can’t, and you can use a pile of counters of size n to determine whether n can be done, by trying to build some kind of truncated triangle of rows whose lengths increase by one. The group all had a good crack at this, and after initially noticing a pattern, which was quickly confirmed by others having found the same pattern, we all had a go at a proof. I explained the proof I’d seen of this, which hinges on divisors, and there was also quite a nice one posted on Twitter (spoilers).
We then moved on to a second counter-based investigation, which involved splitting a row or pile of n counters into two parts, at which point you write down the product of the sizes of the two groups (e.g if you split a row of 5 counters into 2 and 3, you’d write down $2\times 3=6$). Then repeat this for any section of the coins which is in a group of size more than one; e.g. take the new set of 3, split it into 1 and 2 and write down $1 \times 2=2$. When you’ve split the entire set into singles, take the sum of the products you’ve written down. We determined that this value will be the same for any value of n, and the numbers form a pleasing sequence, of which Paul wrote a quite nice proof by induction.
It was at this point that @robiebasak arrived with a large cake, which we promptly demolished (having already eaten @diffractionman‘s flapjacks and crisps) and had a crack at a cube assembly puzzle brought by @samheadleand, which involved each piece having a different colour of faces when viewed from each of the six directions, and the cube had to be assembled keeping all the pieces in the right orientation so all the colours pointed the same way, even inside the cube. I’m not sure if anyone actually solved it, but it was a lovely puzzle. According to its box, it can also be played as a board game in five different ways.
We also saw Sam’s amazing homemade version of SET, which she’d made as a present for Robie, a maths/Python fan:
Playing fake set @MathsJam Manc :) pic.twitter.com/BymLVw9Lpp
— Sam Headleand (@samheadleand) July 23, 2013
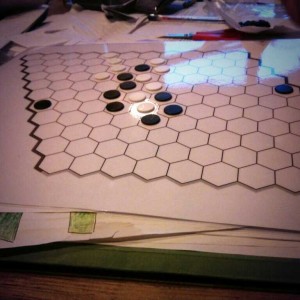
Then we carried on with counter puzzles – next up, coin fountains. The rules are defined as follows: you have to arrange n coins (or in our case, counters) so that they form one block which has a flat base, and any counter higher up in the arrangement is supported by two below it. The number of possible distinct fountains for n coins follows the familiar pattern $1, 1, 2, 3, 5 \ldots$ but then dives off into a controversial 9, and anyone who was thinking Fibonacci numbers gets a smack in the maths-face. The actual sequence, for integer sequence fans, is OEIS A005169. We prodded at this for a while, but didn’t manage to come up with a proof.
At this point, the counters were co-opted for a game of Hex – the blockbusters-style game invented by game theorist John Nash, and discussion continued. We also discussed the nice proof that the alternating sum of reciprocal powers of two sums to a third, which I saw David Bedford give in a recent talk on infinite series.
That’s about all we got to this month – see you next month for more MathsJam recapping!