Happy New Year! And welcome to the first Carnival of Mathematics of 2014. The Carnival is a monthly roundup of blog posts on or related to mathematics, from all over the internet. Posts are submitted by authors and readers, and collated by the host, whose blog it’s posted on. This month, the Carnival has pulled in here at The Aperiodical, and we’re all ready with our party hats for the celebration of mathematical blogging that implies.
You're reading: Features
- From Bracket 1: A002210, the decimal expansion of Khintchine’s constant.
- From Bracket 2: A001220, the Wieferich primes.
- From Bracket 3: A001462, Golomb’s sequence.
- From Bracket 4: A023811, the largest metadromes in base $n$.
- From the first round of reviews, we picked the one whose score we fiddled the least: A010727, all the 7s.
- And the wildcard is A058883, the wild numbers.
An enneahedron for Herschel
The building where I work is named after Alexander Stewart Herschel. I suspect this is because it used to be the home of the physics department, since he was an astronomer, but it works for us too because he also has a pretty cool graph named after him.
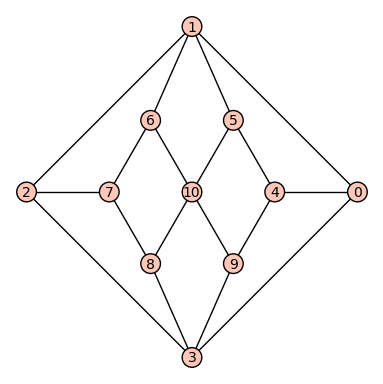
An embedding of the Herschel graph in the plane
Helpfully, it’s called the Herschel graph. It’s the smallest non-Hamiltonian polyhedral graph – you can’t draw a path on it that visits each vertex exactly once, but you can make a polyhedron whose vertices and edges correspond with the graph exactly. It’s also bipartite – you can colour the vertices using two colours so that edges only connect vertices of different colours. The graph’s automorphism group – its symmetries – is $D_6$, the symmetry group of the hexagon. That means that there’s threefold rotational symmetry, as well as a couple of lines of reflection. It’s hard to see the threefold symmetry in the usual diagram of the graph, but it’s there!
Anyway, at the start of the summer, one of the lecturers here, Dr Michael White, told me about this graph and asked if we could work out how to construct the corresponding polyhedron. Making a polyhedron is quite simple – take the diagram on the Wikipedia page, pinch the middle and pull up – but it would be really nice if you could make a polyhedron which has the same symmetries as the graph.
Integer Sequence Review Mêlée Hyper-Battle DX 2000, THE GRAND FINALE
Welcome to the Field of Dreams. Talking of which: why can’t you grow wheat in $\mathbb{Z}/6\mathbb{Z}$?
Anyway, we’re finally here: the Grand Finale of our tournament to find the Integest Sequence 2013. Here’s a reminder of the sequences vying for the title:
It’s a been a long, hard battle. We’ve seen some good sequences, some bad sequences, and an awful lot of plagiarised GIFs. So, without further ado, it’s time to start the
Maths at the Fringe
Starting next week, the historic city of Edinburgh will be taken over by entertainers of all types, performing comedy, dance, theatre and music, entertaining visitors to their massive world-famous festival fringe. Since discerning mathematicians sometimes also enjoy being entertained, I thought I’d write a roundup of the shows maths has non-empty intersection with.
First up, since we haven’t mentioned him in a while, it’s Alan Turing! No, his reanimated corpse isn’t performing edgy stand-up, but theatre company Idle Motion is performing a visual theatre piece entitled That Is All You Need To Know, celebrating the work of Bletchley Park codebreakers. Alan Turing Alan Turing Alan Turing.
How to Win at Pointless
For the benefit of overseas readers, or British readers in full-time employment, I should briefly explain the concept of daytime TV quiz phenomenon Pointless. The pinnacle of British public service broadcasting, it’s shown at 5.15pm every weekday on BBC One and is hosted by Alexander Armstrong of comedy double-act Armstrong & Miller, and Richard Osman of comedy double-act Armstrong & Osman. We shall investigate how we can use maths to analyse the show, improve our chances of winning it, and ultimately perhaps improve the show itself.
The aim of the game is in each round to give the most obscure correct answer to a given question. Each question ($Q$) has a large set of valid answers $A_Q$, questions perhaps asking contestants to name “Films starring Bruce Willis” or “Countries without an O in their name”. All the questions have been asked to 100 members of the public prior to the quiz (call this set $P$), and they each have 100 seconds to name as many examples as they can (giving rise to a set $A_p\subseteq A_Q$ for each $p\in P$. The contestant gets a point for every one of the 100 people who named their answer $a$:
\[ \mbox{score}(a) = \begin{cases}
| \{p\in P : a\in A_p \} | & \mbox{if}\ a\in A_Q \\
100 & \mbox{if}\ a\not\in A_Q.
\end{cases} \]
So an obvious answer like Die Hard or France will score a lot of points, and an obscure answer like Striking Distance or Central African Republic will score fewer points. Points are bad (hence the title) so it’s better to dredge up an obscure answer than stick with something safe. However an incorrect answer like Avatar or Mexico scores the maximum 100 points. At the end of the round the contestant with the most points is eliminated.
Art for a maths department
I don’t think the university maths department I work in has enough art in it. I have gazed covetously upon the walls of other departments I visit, covered with beautiful mathematically-inspired paintings and inspirational posters, serving as a backdrop to cabinets full of geometrical curiosities. I recently suggested to our Head of School that we could buy some art, and he said “That’s a good idea. Send me some suggestions.”
I was pretty delighted with that response, so I spent an enjoyable hour trawling the internet for art that would inspire and enrich our students and staff. We don’t really have anywhere obvious to put sculptures, so I wanted something you can hang on a wall. I had no idea how much money the Head of School was thinking of spending, so I assumed the worst and tried to stick to cheap posters and prints as a starting point. I wasn’t just looking for art – anything to decorate the walls, even if it ends up teaching the students something, is desirable.
My first port of call was my Arty Maths blog. I’ve been collecting nice bits of art that invoke or involve maths (and not art created purely to represent maths) for almost two years now. Unfortunately, it turns out I’ve almost exclusively been collecting sculptures and video works. That meant I had to do some googling!
Because I found some nice things, and in case anyone else is tasked with decorating a maths department and needs ideas, here’s what I found:
The Maths of Star Trek: The Original Series (Part I)
As you may well know, Star Trek was a science fiction TV show in the late 1960s. It featured futuristic technology and science fiction ideas such as warp drives, transporters, strange new worlds, time travel, and green alien space babes. And the possibility of all these things has, in the past, been discussed by experts, and nerds, in great detail. Especially that last one about green space babes.
But dammit, I’m a mathematician, not a physicist. So, instead of talking about the science of Star Trek yet again, what about the maths of Star Trek? After all, Star Trek is science fiction, but there is no such thing as maths fiction – so any mathematics featured on the show is sure to be on firmer ground. Right? Or as Spock himself says in ‘The Conscience of the King’;
SPOCK: Even in this corner of the galaxy, Captain, two plus two equals four.
Should we even expect much maths to feature on a simple space adventure show? In fact, many interesting mathematical ideas were raised during the show’s short run of 79 episodes, including; the probability we are alone in universe; a paradox that upset 20th century mathematicians as well as 23rd century androids; the mathematics of alien and Earth biology; and the most important question of all – when on a dangerous away mission, does the colour of your shirt really affect your chances of survival?