A conversation about mathematics inspired by UUID 0412a969-5b27-4c28-9662-85ef2c201e0c. Presented by Katie Steckles and Peter Rowlett.
Podcast: Play in new window | Download
Subscribe: RSS | List of episodes
Something that whipped round Twitter over the weekend is an early version of a paper by Francisco Aragón Artacho, David Bailey, Jonathan Borwein and Peter Borwein, investigating the usefulness of planar walks on the digits of real numbers as a way of measuring their randomness.
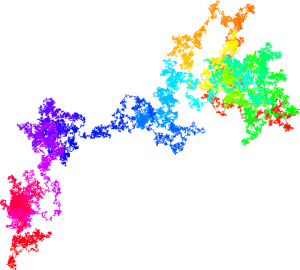
A million step walk on the concatenation of the base 10 digits of the prime numbers, converted to base 4
A problem with real numbers is to decide whether their digits (in whatever base) are “random” or not. As always, a strict definition of randomness is up to either the individual or the enlightened metaphysicist, but one definition of randomness is normality – every finite string of digits occurs with uniform asymptotic frequency in the decimal (or octal or whatever) representation of the number. Not many results on this subject exist, so people try visual tools to see what randomness looks like, comparing potentially normal numbers like $\pi$ with pseudorandom and non-random numbers. In fact, the (very old) question of whether $\pi$ is normal was one of the main motivators for this study.
Alex Bellos, author of Alex’s Adventures in Numberland / Here’s Looking at Euclid, has started another survey about numbers, following his survey to find the world’s favourite number.
This time round, he wants “random” numbers. Answering the survey is very easy: just go to randomnumberservey.net and type a number in the box.
Alex says he hopes to have the results ready by 2013.
Link: the random number survey
Source: Alex Bellos’s blog