Here’s the fourth match in Round 1 of The Big Internet Math-Off. Today, we’re pitting Edmund Harriss against Colin Wright.

Take a look at both pitches, vote for the bit of maths that made you do the loudest “Aha!”, and if you know any more cool facts about either of the topics presented here, please write a comment below!

Edmund Harriss – Penrose tiles

Edmund Harriss is a mathematical artist and a professor at University of Arkansas. Among other things, he’s the co-author of Snowflake, Seashell, Star, a mathematical colouring-in book. You can find him on Twitter at @Gelada, or on his homepage.

Two shapes.

They can fit together

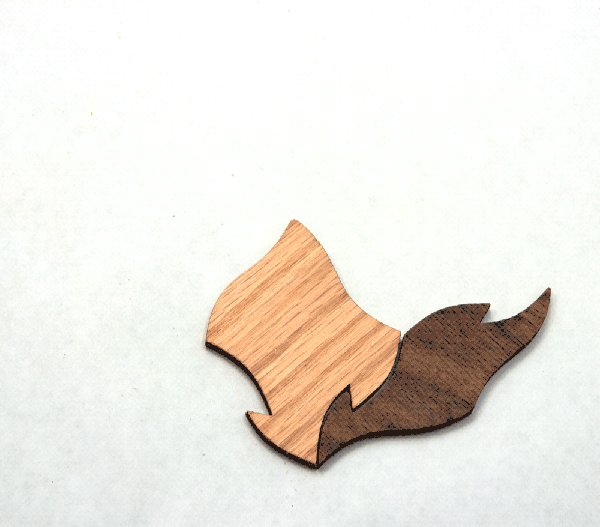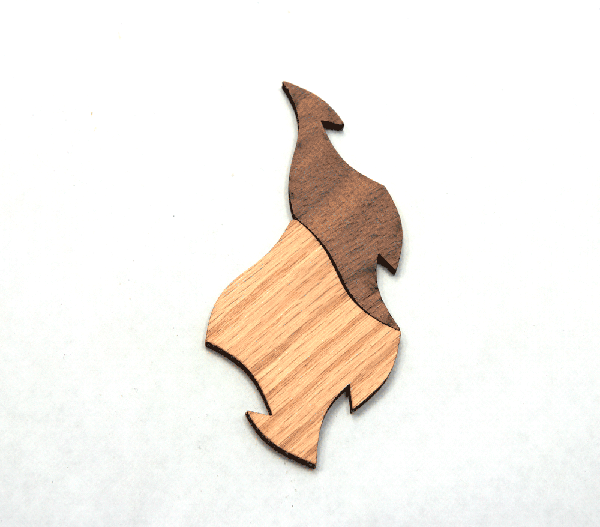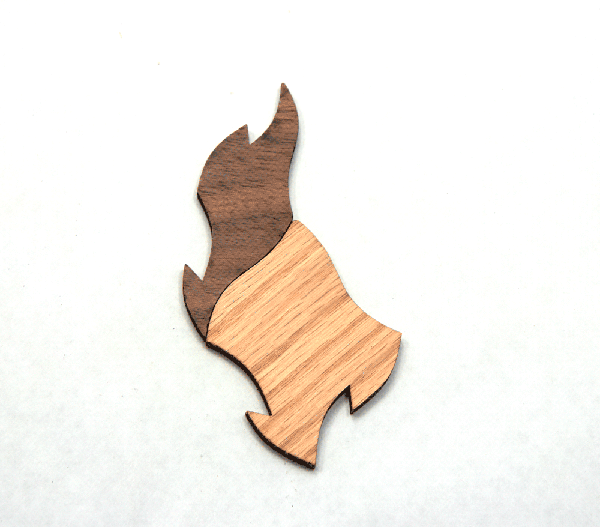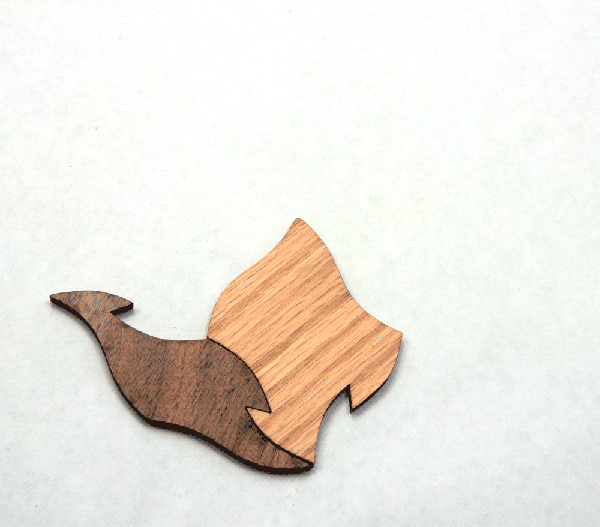
and form loops.

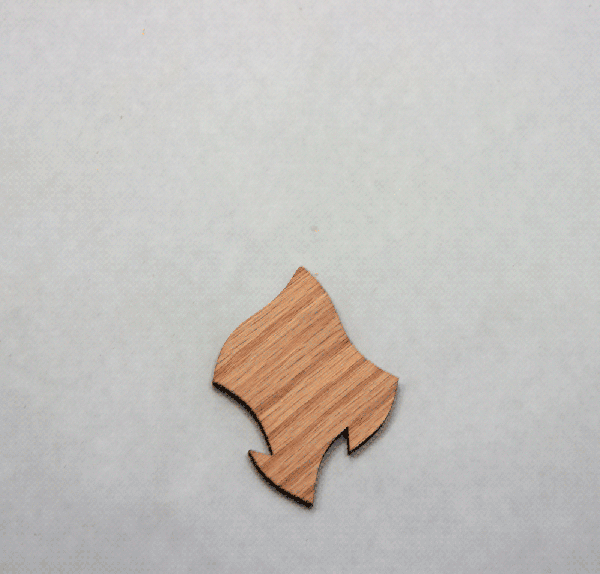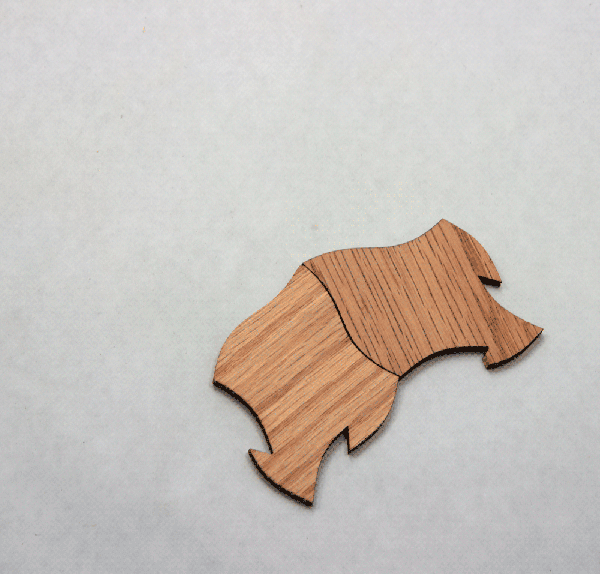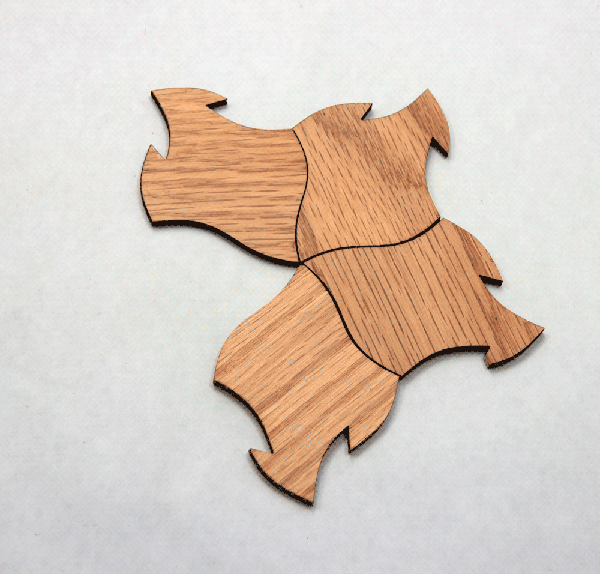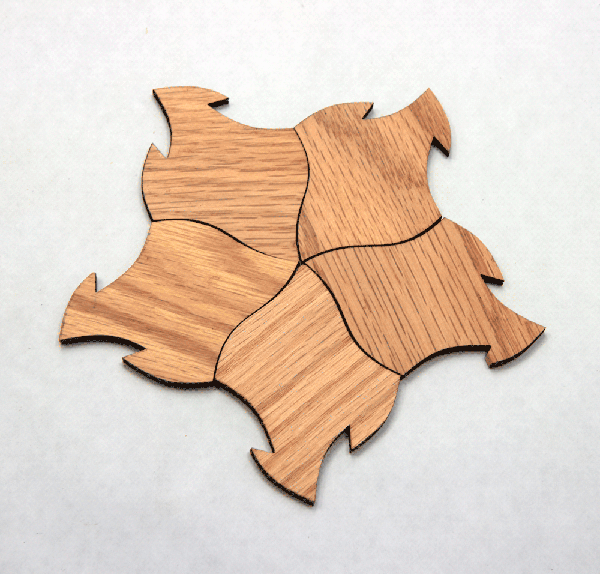
Though you can get stuck if you are not careful: the two thin tiles can fit together in two ways, one is fine

but with this pair any attempt to extend in the V will fail.

With work you can build large regions of tiles

or go even further.

Can you go on forever? Amazingly you can, but the patterns produced will never simply repeat. Such tiles are called aperiodic, in contrast to the repeating periodic tilings (like bricks in a wall).

Their discovery was initially regarded as an interesting curiosity, but then with the discovery of quasicrystals similar patterns were found in nature. This itself was questioned by many chemists and physicists as they believed that crystals had to be periodic. In fact when they were first discovered by Dan Shechtman, he was asked to leave his research institute! I know of no other examples of people being fired for work that would eventually win a Nobel prize.

Find more about the Penrose tiles on Wikipedia’s page on the Penrose tiling, or the Tilings Encyclopedia.

Contact Edmund if you are interested in obtaining the tiles shown here.

Colin Wright – 13 piles of 4 cards

Colin Wright is a maths communicator, organiser of the MathsJam Annual Gathering, and best known as the juggling guy. You can find him on Twitter as @ColinTheMathmo, on Mastodon as @ColinTheMathmo@mathstodon.xyz, or at solipsys.co.uk.

So here’s a weird thing, but you need to play along at home.

Take an ordinary deck of playing cards, no jokers, 52 cards, 13 ranks, four suits. Clearly I’m not going to be able to affect the deck – this isn’t a magic trick. It’s a sort of puzzle.

Arrange the ordering of the cards however you like. You can shuffle, you can put them in order, whatever you choose, and then deal them out into 13 piles, face up, with four in each pile.

You can see the 13 top cards, and there is an extremely small chance that they are all different ranks. As an exercise for the interested reader you can work out the probability, but that’s not where I’m going with this. Of course, since I said you could arrange the deck however you like you could make it so, but if they’re random there is basically no chance that all the ranks of those 13 top cards are different.

But now, in each pile, you are allowed to choose which card goes on top – you’re allowed to choose which card is visible. So in each pile, of the four cards that are there, you can pick which one to have on top.

The question is: can you make them all different?

Another way of saying that is this: If I let you choose one card from each pile, can you select a complete “straight”, from Ace to King?

Try that – see if you can get some feeling for just how hard the challenge is, and where the difficulties might lie. There is a chance that you get an easy arrangement, so try it a few times to get a feel.

As we noted earlier, there’s a vanishingly small chance that the top cards were already all of different ranks, but suppose that the original ordering was random, or that the original ordering was devised by an adversary. Can you still choose one in each pile to make them all different?

What are the odds? Clearly it’s sometimes possible, but is the probability of success close to 1? Close to 0? Somewhere in between?

There are a lot of orderings of a deck of playing cards.

(To get some feeling for the number of orderings of a deck of playing cards, suppose you had a different ordering every nano-second since the Big Bang, for each of 10 billion people on each of 10 planets around each of 100 billion stars in each of 100 billion galaxies. You’d still need over a million billion universes to get all the possible orderings.)

With so many orderings you might feel that a sufficiently clever adversary could find an arrangement that makes this task impossible.

But no. Here’s the thing:

For every initial arrangement of the cards, it’s possible to choose one from each pile and end up with one of each rank.

Are you surprised? Maybe, maybe not, but let’s continue.

Having chosen one of each rank, one from each pile, remove them, and now you have 13 piles of three. Can you do it again?

And again?

And again? (Actually, the fourth one you get for free).

If that’s possible (and it is!) then you have taken that original deal of thirteen piles of four, and arranged each pile so that the four slices each contain every rank.

I find that somewhat unexpected.

So, which bit of maths has tickled your fancy the most? Vote now!

 Loading ...

The poll closes at 9am BST on the 6th. Whoever wins the most votes will get the chance to tell us about more fun maths in round 2.

Come back tomorrow for our fifth match in round 1, pitting Tiago Hirth against Evelyn Lamb, or check out the announcement post for your follow-along wall chart!