Here’s a selection of news stories about mathematical prizes that have been awarded/announced in the last couple of months.
You're reading: Posts Tagged: Jon Borwein
Walking on π in the NSF Visualization Challenge
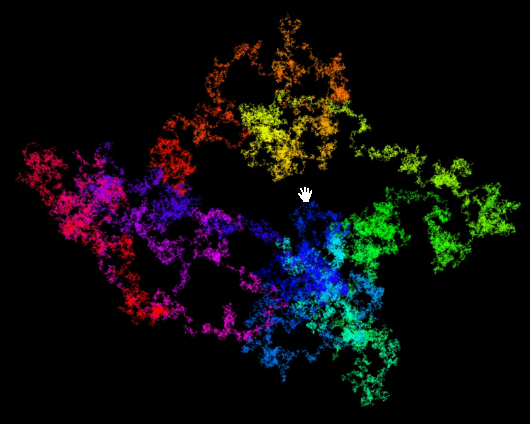
Fran Aragón Artacho has emailed to tell us that he and Jon Borwein have entered their image of a walk on the first 100 billion digits of π in the National Science Foundation (of the USA)’s International Science & Engineering Visualization Challenge. Fran says:
Jon Borwein and I have submitted our picture of a walk based on 100 billion digits of pi to a visualisation contest from the NSF (National Science Foundation). The winners will appear in Science (one will be selected for the front cover!). And we have good news: we are one of the 10 finalists in the Illustration category!
WLTM real number. Must be normal and enjoy long walks on the plane
Something that whipped round Twitter over the weekend is an early version of a paper by Francisco Aragón Artacho, David Bailey, Jonathan Borwein and Peter Borwein, investigating the usefulness of planar walks on the digits of real numbers as a way of measuring their randomness.
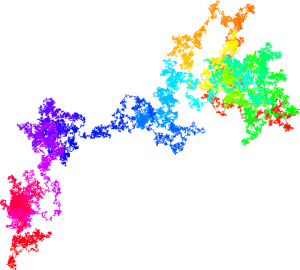
A million step walk on the concatenation of the base 10 digits of the prime numbers, converted to base 4
A problem with real numbers is to decide whether their digits (in whatever base) are “random” or not. As always, a strict definition of randomness is up to either the individual or the enlightened metaphysicist, but one definition of randomness is normality – every finite string of digits occurs with uniform asymptotic frequency in the decimal (or octal or whatever) representation of the number. Not many results on this subject exist, so people try visual tools to see what randomness looks like, comparing potentially normal numbers like $\pi$ with pseudorandom and non-random numbers. In fact, the (very old) question of whether $\pi$ is normal was one of the main motivators for this study.