If you’ve heard of Felix Klein, it’s probably due to the Klein bottle, that strange four-dimensional object that is the subject of a new video here on The Aperiodical starring Katie Steckles and Matt Parker. Who is Klein and, apart from the bottle, what did he do?
Klein’s Times obituary records that he would point out “with a smile” that his date of birth comprised three squares of primes. So then, I will refer to his birth as taking place on the $( 5^2 )^{\textrm{th}}$ day of the $(2^2 )^{\textrm{th}}$ month in the year $43^2$ in Prussia. You might like to notice that today is the $( 5^2)^{\textrm{th}}$ day of the $( 2^2 )^{\textrm{th}}$ month as well, so it is the 163rd anniversary of Klein’s birth.
Klein originally intended a career in physics but got into geometry through a doctoral dissertation on the applications of line geometry to mechanics and later work on the foundations of line geometry. The MacTutor Archive biography of Klein says that his contribution to geometry
has become so much a part of our present mathematical thinking that it is hard for us to realise the novelty of his results and also the fact that they were not universally accepted by all his contemporaries.
He collaborated with Sophus Lie to study the Kummer Surface, an irreducible algebraic surface of degree 4 (leading to the Klein configuration, an invariant of that space) and, later, W-curves, which are curves invariant under certain projective transformations. Klein’s legacy in the field includes Klein Quartics and Klein Quadrics, the Klein model and the Klein Geometry.
Lie also introduced Klein to the idea of a group; Klein is said to have considered his work on function theory, including results in group theory, to be his major contribution to mathematics. The Klein Four Group (a group so inspirational, it has its own Barbershop Quartet) and Kleinian Groups are both named after him – the sheer number of mathematical objects which bear his name is testament to how fundamental much of his work was.
On being appointed to a chair at the University of Erlangen-Nüremburg aged just 23, Klein published a manifesto outlining what would become known as the Erlanger Programm. In this influential research programme, he first promoted the use of group theory in describing geometry, as well as placing more emphasis on projective geometry and attempting to unify the different types of language used in geometry via symmetry groups. This work profoundly influenced the evolution of mathematics. The Wikipedia page gives a good summary of the contemporary mathematical context from which the Erlanger Programm arose.
One of Klein’s major projects was the modernisation of mathematical education. As well as producing copious lecture notes, he coedited the landmark Encyklopädie der mathematischen Wissenschaften mit Einschluss ihre Anwendungen (Encyclopaedia of Mathematical Sciences including their Applications) with Wilhelm Meyer, and for fifty years edited the journal Mathematische Annalen, which covers a wide spectrum of modern mathematics and continues to be well regarded to this day.
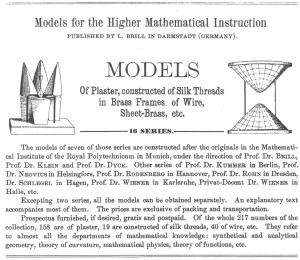Klein was keen to promote the use of physical models as a way of improving the understanding of abstract mathematical objects. He and Alexander von Brill enlisted graduate students to construct, from brass wire and plaster, models of surfaces that had previously only existed as equations. By drawing geodesic lines on the models, mathematicians could see with their own eyes the curvature of a space. David Hilbert got so carried away with this that he drew parabolic curves all over a bust of Apollo Belvedere to test a theory that beauty was linked to the equal distribution of parallel parabolae on the human face (it wasn’t).
Klein exhibited the models at the Chicago World’s Fair in 1893, resulting in replicas being sold to maths departments and museums all over the world. Artists such as Naum Gabo, Henry Moore and Man Ray were influenced greatly by these weird shapes, the like of which had never been seen before. An exhibition at the Science Museum in London is currently displaying some of the works inspired by Klein’s models.4,5
The MacTutor biography also refers to a “great talent at teaching” with a list of seven students at Technische Hochschule in Munich who also merit biographies in the MacTutor Archive. Klein was a driving force behind the decision in 1893 to allow women to study at Göttingen, and supervised Grace Chisholm Young, the first woman to earn a doctorate in mathematics there.
Later in his career Klein took an interest in school teaching. He was Chair of the International Commission on Mathematical Instruction at the Rome International Mathematical Congress of 1908 and worked through the German branch of the Commission to publish volumes on the teaching of mathematics in Germany.
In the preface to a translation of his Elementary Mathematics From An Advanced Standpoint – Arithmetic – Algebra – Analysis, which can be viewed on Amazon, he states his wish that the work
may prove useful by inducing many of the teachers of our higher schools to renewed use of independent thought in determining the best way of presenting the material of instruction. This book is designed solely as such as a mental spur, not as a detailed handbook.
In the preface to the third edition, Klein wrote that his two volumes of Elementary Mathematics from an Advanced Standpoint were intended to bring to the attention of secondary maths and science teachers “the significance for their professional work of their academic studies, especially their studies in pure mathematics.” Klein was writing not a textbook but a guide to higher mathematics, to encourage school teachers to a greater awareness of and interest in higher level mathematics. These aims are not unfamiliar to modern readers.
Indeed, The Klein Project seeks to replicate this vision for a modern audience. Taking inspiration from the intent of Klein’s book, its website states the intention of the project to create
a 300-page book written to inspire teachers to present to their students a more complete picture of the growing and interconnected field represented by the mathematical sciences in today’s world.
Today, 163 years after his birth, Klein should be remembered not just as someone who invented an impossible bottle, but as an excellent researcher who made many important advances in mathematics as well as wider contributions to the discipline, through excellent teaching, cultivation of the literature, and a legacy which continues to inspire both mathematicians and non-mathematicians to appreciate the beauty of higher mathematics.
Further reading
- MacTutor Klein biography
- Biography: Felix Klein – American Mathematical Monthly
- Wikipedia – Felix Klein
- “Mathematical Objects” – Cabinet magazine, issue 41.
- Theory and History of Geometric Models – Irene Polo-Blanco
- The Princeton Companion to Mathematics
Nice article. There is one point that I would like to clarify about the nature of the Klein bottle. This topological structure of the Klein bottle is actually 2 dimensional, not 4. It is true that it cannot be embedded in any real space of dimension less than 4, though. However, the dimension of a manifold (surfaces are 2-dimensional manifolds) is a characteristic of the manifold itself, not of any of the spaces into which it embeds. In other words, dimension is an intrinsic property. To understand what I mean by dimension here, just observe that if you cut out any tiny disk on the surface of a Klein bottle, you can map that to a tiny disk in the real plane. In other words, the Klein bottle looks ‘locally’ like the plane, so it is similarly 2-dimensional.
Another thing worth mentioning is that the connections that Klein forged between groups and geometry are still fundamental to how the two fields function today. A tremendous number results in 3-manifold topology (and surface topology) are basically results that are actually about the fundamental group.
For instance, the recently proven Virtual Haken Conjecture says that a 3-manifold has a finite cover with an embedded surface (sorry if this is getting a bit far afield)… What about that sounds like group theory? It turns out that the proof relies crucially on group theory in almost every regard. In particular, hyperbolic group theory.. and hyperbolic geometry was another topic Klein helped introduce.
Thanks for that.
How do you know that it was Hilbert who traced the parabolic curves on the Apollo Belvedere? In Hilbert & Cohn Vossen’s book, Geometry and the Imagination, there is the following:
“F. Klein used the parabolic curves for a peculiar investigation. To test his hypothesis that the artistic beauty of a face was based on certain mathematical relations, he had all the parabolic curves marked out on the Apollo Belvedere, a statue renowned for the high degree of classical beauty in its features. But the curves did not possess a particularly simple form, nor did they follow any general law that could be discerned”.
That doesn’t exactly sound like Hilbert did the tracing.