Yesterday I gave a talk to the Nottingham Trent University Maths Society, ‘A brief history of mathematics: 5,000 years from Egypt to Nottingham Trent’. I had a slide in this where I said something about what the Greek style of proof means for mathematics. It has helped me put my finger on something of why mathematics isn’t like science, and I thought I would share it here so I can look it up when I’ve forgotten again.
You're reading: Posts Tagged: history
Recent history
I am preparing a talk for our undergraduate Maths Society (perhaps ill-advisedly) with the title ‘A brief history of mathematics: 5,000 years from Egypt to Nottingham Trent’. This will be a stampede through some very selected hightlights, starting with some arm waving about pyramids and ending with something modern. In fact, as well as some recent results that have been reported on this site this year, I intend to end with a recent piece of research published in the department (and a colleague has promised a nice picture of a brain for this ((Something to do with complexity and computational graph theory.))).
Relatedly, a colleague who teaches on our Financial Mathematics degree suggested I include Black–Scholes in my talk, as one of the most recent results included in an undergraduate degree. It’s from 1973. Can we do better? I asked Twitter.
Éditions Hermann donates its Bourbaki archives to the Bibliothèque nationale de France
A collection of material pertaining to Nicolas Bourbaki, author of the famous Elements of Mathematics, has been donated to the French national library by his publisher, éditions Hermann. Bourbaki set out to reframe modern maths in terms of set theory, to give the subject a coherence that would lead to more rigour and cross-application of results.
The donated material consists of “original texts, corrected proofs of the Elements of Mathematics, as well as various items related to the publication of the books including catalogues, press releases, contemporary journal articles on Bourbaki, and letters.”
More information (all in French)
Les éditions Hermann font don d’archives Nicolas Bourbaki à la Bibliothèque nationale de France – (PDF) press release from the BNF and Hermann.
Les Archives Bourbaki à la BNF – potted history of Bourbaki in Libération.
Nicolas Bourbaki fait son entrée à la BNF! – hour-long radio programme on France Culture, including contributions from Pierre Cartier, former avatar of Bourbaki, and Guillaume Fau, curator of manuscripts at the BNF.
Nicolas Bourbaki entry on MacTutor (in English)
Mathematics: a culture of historical inaccuracy?
Earlier this year, back when I somehow managed to find time to write blog posts (sorry!), I wrote a couple of pieces on incorrect but oft-repeated stories in history of mathematics, basically describing some issues and expressing my frustration. These were Apparently Gauss got in this bar fight with Hilbert… and Why do we enjoy maths history misconceptions?
Today Thony Christie wrote on Twitter (as @rmathematicus) with a link to this post by Dennis Des Chene (aka “Scaliger”): On bad anecdotes and good fun. As Thony points out, this is an “excellent piece of maths history myth busting” and I am writing this quick note to commend you to read it.
Bletchley Park Turing First Day Cover
For my recent birthday I was given a wonderful present: a special UK stamp commemorating Alan Turing, who was born 100 years ago today. The stamp was issued by the Royal Mail not for the Turing centenary but as one of a series of special stamp sets to mark the Queen’s Diamond Jubilee.
This stamp is particularly special because it is one of 1000 which originated at the Bletchley Park Post Office that were stuck onto a specially designed envelope (Turing, mathematics and patterns) and cancelled on the day the stamp was issued, 23 February 2012, using a special Bombe-themed postmark.
Historical anniversaries: are they worth celebrating?
It probably hasn’t escaped your attention that this year marks 100 years since Alan Turing was born, and that the actual anniversary of his birth is tomorrow. There is massive interest in this fact, both from specialist maths and computing outlets and the mainstream press. What, though, is the significance? You don’t need me to tell you that any anniversary is fairly arbitrary. The earth has gone a whole number of times around the sun since the event (within some margin of error). What, really, does this mean? And attributing special interest to one particular number of anniversaries just because it is a factor of ten or a neat fraction of one hundred is wholly meaningless.
So I don’t fall for anything like that, right? Wrong. For a couple of years now I have been tweeting a link to a biography of a mathematician who was born or died on each date to the Twitter feed @mathshistory on behalf of the British Society for the History of Mathematics. This generates some interest and I am delighted when it does so.
So can I justify anniversaries as having some tremendous significance or is this all just a cynical attempt to grab attention? To a great extent it is the latter. BSHM have a charitable aim to “promote and develop for the public benefit, awareness, knowledge, study and teaching of the history of mathematics”. If anniversaries are going to generate greater awareness of and interest in history, then I’m in.
For the daily tweeting I rely entirely on the excellent MacTutor History of Mathematics archive (so much so that some people think I run the site, or MacTutor runs the Twitter feed).
Basically, I choose a mathematician who was born or died on each day according to a bunch of constraints. People sometimes tweet and say “why have you chosen X; what about Y?”
The basic ground rules are: one tweet per day, each mathematician once per year. This causes some conflicts that people don’t naturally understand. For example, on 23rd January I tweeted about James Lighthill, who MacTutor describes as “one of the foremost English applied mathematicians of his day”. Why, wondered Twitter user @gemmarobles, was I ignoring David Hilbert, also born that day? Well, I included Lighthill on his birthday because he died on the same day that Lexis was born, who MacTutor report as “initiating the study of time series”. And Lexis died on the day Galois was born. And although there are a few mathematicians who were born or died on 14th February, when Hilbert died, there is no clear issue with placing Hilbert on that day. So why did I choose Lighthill over Hilbert? Because Galois was born on the day Lexis died. At some point, an arbitrary decision needs to be made and this has consequences down the line.
Apart from these basic constraints, I have a bunch of extra rules. I am doing this to try to generate interest, so I try include some variety. I like to try to tweet from different eras and different mathematical areas on adjacent days if possible. I favour time periods when few dates are known or cultures without many mathematicians in the database because these have fewer opportunities to get picked. I also think it is good to highlight women in mathematics or other important issues such as race or disability, again if possible. It is also pleasing to make people aware of the mathematical contributions of mathematicians who are better known for something else, or people who were not principally mathematicians but made a contribution. Anyone who meets some of these criteria might see favour over other mathematicians associated with the same day.
Still, much as I like to include mathematicians that people won’t know and highlight time periods and issues they haven’t thought about, it is the big hitters, Euler and Gauss and other well known names, who get the large numbers of retweets and interest. So I include them because that is how followership of the account grows and links to maths history content spread. If people are only going to take an interest on a famous anniversary, at least they are taking an interest at all.
Do I think the world has gone over the top on Turing? I do think there is value to be had. Leveraging Turing’s name and the interest generated by his centenary to attempt to do some good for gay rights is a noble undertaking (although I have my doubts over the precise details). Using Turing to try to generate extra interest in the history of mathematics, cryptography and computing is worthwhile. If we aren’t going to get people’s attention at the big 100, when will we? I remember seeing a lecture by Robin Wilson where he lamented the relative lack of interest in the 300th anniversary of Euler’s birth in 2007, which could have been a great opportunity to raise the profile of mathematics in wider culture. It’s clear to see why the 305th anniversary this year just hasn’t got the same traction.
However, I worry about the others involved with the war work at Bletchley Park or the early development of computers who are getting eclipsed, and, for that matter, all the other history of maths and computing stories that are worth telling but can’t get the attention. Celebrating the big names supports the idea that advances are made in giant leaps by great men (mostly men), whereas history is constantly being made in small steps. On top of this, I worry that the attention people are giving Turing is fairly superficial. People aren’t, I think, gaining a wider understanding of the historical context into which Turing fits, or of the place of mathematics research in our culture. And I worry that this interest won’t be sustained. What happens in the cold light of Sunday morning when it’s all over? Perhaps we can sustain some interest until the end of the centenary year but will ‘Turing100’ have a lasting impact on people’s minds? Turing died in 1954. Will we ignore him again until 2054?
Anyway, must dash. I have to draft my exciting Turing centenary day post for tomorrow.
Carnival of Mathematics 85
 |
| 85 by brighterorange |
Introduction
Welcome to a new Carnival of Mathematics! Traditionally the Carnival opens with facts about the number, this time 85, but first I have an important point of admin to address.
From Carnival of Mathematics 59 in November 2009 until Carnival of Mathematics 84 in December 2011, the Carnival was coordinated by Mike Croucher. Mike said in a blog post (of course!) that he had “had a lot of fun doing so” but that: “Recently, however, I have struggled to find the time to give the CoM the attention it deserves and so it is time to hand over the baton.” We should all be very grateful to Mike for his effort over these two years. You can still find Mike blogging over at Walking Randomly.
Now, as a result, the Carnival has a new home (including an index of previous Carnivals) at The Aperiodical, a not-quite-yet-formally-announced blogging collaboration between Katie Steckles, Christian Perfect and me. We’ll do our best to look after the series for the time being. If you’ve been following the Carnival for a while you’ll know it only works because of a parade of volunteer hosts. The next few Carnivals are lined up but if you’d like to volunteer to host one on your blog later in the year please contact Katie. The other necessary element is submissions, and we have plenty of these this time, so let’s get to business.
 |
| Funky new Carnival logo by Katie Steckles |
85
This is Carnival of Mathematics 85. The ever-faithful Number Gossip tells me that 85 has no unique or even rare properties, being merely composite, deficient, evil, odd, square-free and a Smith number. Beyond mathematics, Wikipedia tells me that 85 is the atomic number of astatine, the ISBN Group Identifier for books published in Brazil and the lower bound (due to incomplete research) found by Jorge Stolfi (2004) for The Hollywood Constant, the smallest non-negative integer that has never been used in the title of a movie.
Serious mathematics
(Not that the rest isn’t!)
Brent Yorgey at The Math Less Traveled wrote a series of four posts in response to Depressing Expressions by Patrick Vennebush over at Math Jokes 4 Mathy Folks. In these, he is working to prove why a certain iterative arithmetic algorithm always results in a factorial. Brent says:
In particular I’m proving it using a *combinatorial* proof, a lovely proof technique that (in my opinion) isn’t used or taught as widely as it ought.
In part four Making Our Equation Count, he says,
I go through the different bits of the equation we’re trying to prove, and explain (with pictures) how to interpret each of them combinatorially.
Rebecka Peterson at Epsilon-Delta writes Extraneous Solutions of Log Equations–A Graphical Explanation. In submitting this post, Rebecka wrote:
Last semester a College Algebra student of mine asked why we sometimes get extraneous solutions when solving log equations. It was such a good question. I tried to do it justice in this post.
Drawing inspiration from the award of the Leroy P. Steele Prize for Mathematical Exposition to Aschbacher, Lyons, Smith, and Solomon for a work about the classification of finite simple groups, Gianluigi Filippelli at Doc Madhattan offers a post about finite simple groups and connections with physics in The classification of finite simple groups.
Frederick Koh from White Group Mathematics submitted Understanding MATTERS (4) saying:
Of late I noticed quite a few students (in online forums) experiencing difficulties in comprehending the concept of calculating distances between 3 dimensional vector planes in space, hence I am sharing an in depth explanation behind how things work.
Rohit Gupta at Kali & the Kaleidoscope investigates a new visualization of Möbius’ Mu, “a notorious function to classify all integers in three different boxes” in The 3 Pills of Möbius.
Art
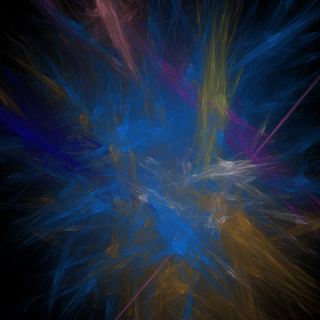
Thomas Egense from Thomas’ mathematical adventures writes about an attempt to create mathematics-inspired art algorithmically using something called “Fractal flames”. Thomas defines fractal flames, details the algorithmic work involved, and gives several examples of the generated artwork, including the image below (used with permission).
Thomas Egense also submitted Dimensions (series of nine videos embedded below) calling this an “impressive graphical visualization of hard to grasp mathematical concepts like higher dimensions and topology”.
Last week Gathering for Gardner 10, the meeting of mathematicians, magicians, puzzlers and others inspired by the life and work of Martin Gardner, took place. Edmund Harriss, from Maxwell’s Demon, previewed his G4G10 talk in a blog post, The 2×1 rectangle and Domes. Edmund begins with the humble 2×1 rectangle, “not one of mathematics most celebrated shapes”, and ends up with structures built as accommodation at the Burning Man event.
Pop culture
Matt over at Math Goes Pop! writes in The Probability Games about the process used to select participants to take part in a fight to the death in the book and film The Hunger Games. Matt says “the rules here practically beg for some mathematical analysis” and Katie Steckles, who submitted the post, called this “just the kind of unnecessary mathematical analysis of a situation I like to see”.
Video game mathematics
Drawing inspiration from the recent arXiv preprint Classic Nintendo Games are (NP-)Hard bringing video games “out of nerdy obscurity and into cutting edge computer science”, Sam Alexander from Xamuel.com writes Toward the Mathematics of Video Game Glitches. Sam noticed a minor error in that article based on a glitch in Super Mario Brothers. Running with this theme, he defines video game glitches, game theoretically speaking, and focusing on “glitches which the player can exploit to win the game faster than intended”, defines a theorem (which he describes as “committing horrendous crimes against mathematics”!).
 |
| SNES vs. Xbox Triple60 by avail |
Performance and puzzles
Ben Nuttall writes about his experience as a maths busker.
“What is Maths Busking?” I hear you ask. Maths Busking is a street performance of mathematics whereby the buskers demonstrate mathematical ideas and engage the public in thinking like a mathematician
As well as a fuller explanation of maths busking, Ben shares some of the ‘busks’ he performed, his thoughts on the experience and some photos.
 |
| Birmingham City Centre by Maths Busking |
Katie Steckles, writing at The Aperiodical, discusses a variant of a popular mathematics ‘mind reading’ trick. I don’t want to spoil the puzzle in case you want to play along at home, so go over and check out On Disreputable Numbers.
Paul Taylor, also at The Aperiodical, writes about a puzzle he designed for Katie Steckles’ Puzzlebomb. Puzzlebomb is a monthly puzzle sheet featuring all-new types of puzzles. Following the release of the April Puzzlebomb, Paul made the following claim on Twitter:
I guarantee there has never been, and will never be, another puzzle quite like Hilbert’s Space Filling Crossword
In Words to Fill Space he justifies this assertion and describes how he created the puzzle.
Paul also writes, again at The Aperiodical, about a class of puzzle in which a number of prisoners are all given hats and their fate depends on their ability to correctly determine the colour of their own hat. Paul offers “a nice variation on the theme that I heard about at a recent MathsJam” as Another black and white hats puzzle.
Maths Jam is a monthly meeting of maths enthusiasts in pubs worldwide to share stuff they like. “Puzzles, games, problems, or just anything they think is cool or interesting“. A recent development is blogging roundups of what happened at Maths Jam meetings. Recent outings, full of puzzles and mathematical goodies, include: Newcastle (February), Manchester (March), London (February) and Melbourne (January), and a set of photos from various February Maths Jam meetings.
 |
| Maths Jam London February 2012 |
Last month I attended Newcastle Maths Jam. While there we played with a puzzle that Out of the Norm states as:
By relabelling the faces of two dice, can you design a new, unusual pair of six-sided dice that achieves rolls with the same frequencies as a pair of normal dice? All the faces must have a positive number of spots.
Dice and Dissection: a puzzle discusses this puzzle and gives a nice diagrammatic way to view the solution.
History and society
John Cook of The Endeavour writes about a wedding invitation written under the collective pseudonym of that “semi-secret group of French mathematicians”, Nicolas Bourbaki. In Nicolas Bourbaki’s wedding invitation, John explains some of the mathematical references and reveals how the invitation “nearly cost Bourbaki member André Weil his life”.
A post on Pat’sBlog goes to primary sources to highlight an error in Wikipedia in relation to the origins of the four-fours problem. That is,
using four fours and whatever mathematical operations that were allowed to make a number, or a set of numbers.
The origins of the problem apparently lie in earlier similar problems, including a problem of four threes. Read about it in Before There Were Four-Fours, There Were Four-Threes.
Guillermo Bautista at Mathematics and Multimedia writes A US President’s Proof of the Pythagorean Theorem about the proof of the Pythagorean theorem formulated by James Garfield, the 20th president of the United States of America.
Alexander Bogomolny of CTK Insights offers a little piece of mathematics in the history of chronology given in Florian Diacu’s The Lost Millennium in a post entitled Chinese Remainder Theorem: an Application to Chronology. Submitting this post, Alexander said:
Chinese Remainder Theorem is a staple of early puzzle books, both European and Eastern. This is very satisfying to learn that the theorem finds practical and important applications in the science of chronology. The book by Florian Diacu where I found this application is an exquisitely written compendium of history and mathematics of calendrical calculations. The book deserves every praise and gets my wholehearted recommendation.
In a blog post here on Travels in a Mathematical World, I was very taken with an answer given to a question about working outside traditional academia by Neil deGrasse Tyson in an interview with Samuel Hansen for the Strongly Connected Components Podcast episode 45. The whole interview (18 mins) is worth listening to. My reflections can be found as Culturally an academic.
Over at Second-Rate Minds, Samuel Hansen writes about The True Importance of Friends, explaining the origin of a result in social network theory and its implications in epidemiology.
I really noticed the absence of the Carnival back in February when I thought I might submit a couple of blog posts which got a particularly warm reaction and found the submissions form deactivated. The posts were Apparently Gauss got in this bar fight with Hilbert… and the follow-up Why do we enjoy maths history misconceptions?
Technology
John Chase from Random Walks writes about the surprising features of Microsoft Office Equation Editor, in which he makes the bold claim: “LaTeX lovers will love it”. Find out why at Microsoft Office Equation Editor.
I’ve saved the last word for this revived Carnival to the previous coordinator, Mike Croucher. Mike’s month of math software for March 2012 offers the latest news in the world of mathematical software (a month of math software has been a monthly series since January 2011).
The End
Well, that’s that for this Carnival. You can help spread the word by blogging, tweeting, etc. about the revival of the Carnival and directing people to this edition. If you’re hungry for more mathematics blog posts then there’s the previous Carnival of Mathematics 84, the latest Math Teachers At Play Carnival 48 is over at Math Is Not A Four Letter Word and you can get a weekly selection of blog posts from the Mathblogging.org Weekly Picks.
Future outings for the Carnival of Mathematics are queued over at The Aperiodical. Carnival of Mathematics 86 will be posted in May by Brent at The Math Less Travelled. Submissions for this are now open, so keep your eye out for great posts and get writing!